Question Number 90435 by naka3546 last updated on 23/Apr/20

Commented by MJS last updated on 23/Apr/20
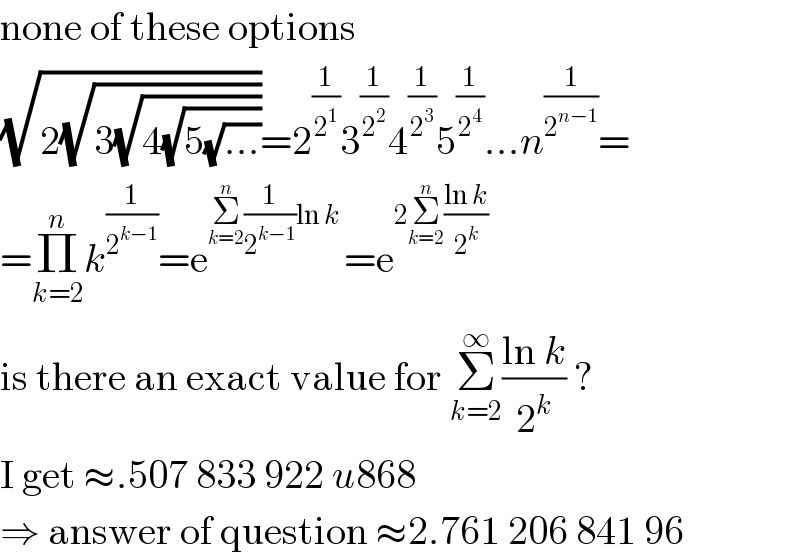
$$\mathrm{none}\:\mathrm{of}\:\mathrm{these}\:\mathrm{options} \\ $$$$\sqrt{\mathrm{2}\sqrt{\mathrm{3}\sqrt{\mathrm{4}\sqrt{\mathrm{5}\sqrt{…}}}}}=\mathrm{2}^{\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{1}} }} \mathrm{3}^{\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }} \mathrm{4}^{\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{3}} }} \mathrm{5}^{\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{4}} }} …{n}^{\frac{\mathrm{1}}{\mathrm{2}^{{n}−\mathrm{1}} }} = \\ $$$$=\underset{{k}=\mathrm{2}} {\overset{{n}} {\prod}}{k}^{\frac{\mathrm{1}}{\mathrm{2}^{{k}−\mathrm{1}} }} =\mathrm{e}^{\underset{{k}=\mathrm{2}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{\mathrm{2}^{{k}−\mathrm{1}} }\mathrm{ln}\:{k}\:} =\mathrm{e}^{\mathrm{2}\underset{{k}=\mathrm{2}} {\overset{{n}} {\sum}}\frac{\mathrm{ln}\:{k}}{\mathrm{2}^{{k}} }} \\ $$$$\mathrm{is}\:\mathrm{there}\:\mathrm{an}\:\mathrm{exact}\:\mathrm{value}\:\mathrm{for}\:\underset{{k}=\mathrm{2}} {\overset{\infty} {\sum}}\frac{\mathrm{ln}\:{k}}{\mathrm{2}^{{k}} }\:? \\ $$$$\mathrm{I}\:\mathrm{get}\:\approx.\mathrm{507}\:\mathrm{833}\:\mathrm{922}\:{u}\mathrm{868} \\ $$$$\Rightarrow\:\mathrm{answer}\:\mathrm{of}\:\mathrm{question}\:\approx\mathrm{2}.\mathrm{761}\:\mathrm{206}\:\mathrm{841}\:\mathrm{96} \\ $$
