Menu Close

Question-91326

Tinku Tara 0 Comments
Pin




Question Number 91326 by mhmd last updated on 29/Apr/20
Commented by mathmax by abdo last updated on 30/Apr/20
I =∫ (dx/((x−1)^2 (√(x^2 −x+1)))) ⇒ I =_(x−1=t) ∫ (dt/(t^2 (√((t+1)^2 −(t+1)+1)))) =∫ (dt/(t^2 (√(t^2 +2t+1−t−1+1)))) =∫ (dt/(t^2 (√(t^2 +t+1)))) =∫ (dt/(t^2 (√((t+(1/2))^2 +(3/4))))) =_(t+(1/2)=((√3)/2) sh(u)) (2/( (√3)))∫ (1/((((√3)/2)sh(u)−(1/2))^2 ch(u)))×((√3)/2)ch(u)du =4∫ (du/(((√3)shu−1)^2 )) =4 ∫ (du/(3sh^2 (u)−2(√3)shu +1)) =4 ∫ (du/((3/2)(ch(2u)−1)−2(√3)shu +1)) =8 ∫ (du/(3ch(2u)−3−4(√3)shu+2)) =8 ∫ (du/(3sh(2u)−4(√3)sh(u)−1)) =8 ∫ (du/(3((e^(2u) −e^(−2u) )/2)−4(√3)×((e^u −e^(−u) )/2)−1)) =16 ∫ (du/(3e^(2u) −3e^(−2u) −4(√3)e^u +4(√3)e^(−u) −2)) =_(e^u =z) 16 ∫ (dz/(z(3 z^2 −3z^(−2) −4(√3)z+4(√3)z^(−1) −2))) =16 ∫ ((z^2 dz)/(z(3z^4 −3−4(√3)z^3 +4(√3)z −2z^2 ))) =16 ∫ ((z^2 dz)/(z(3z^4 −4(√3)z^3 −2z^2 +4(√3)z −3))) decomposition of F(z) =(z^2 /(z(3z^4 −4(√3)z^3 −2z^2 +4(√3)z −3))) ...be continued...
$${I}\:=\int\:\:\frac{{dx}}{\left({x}−\mathrm{1}\right)^{\mathrm{2}} \sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}}\:\Rightarrow\:{I}\:=_{{x}−\mathrm{1}={t}} \:\:\:\:\int\:\:\frac{{dt}}{{t}^{\mathrm{2}} \sqrt{\left({t}+\mathrm{1}\right)^{\mathrm{2}} −\left({t}+\mathrm{1}\right)+\mathrm{1}}} \\ $$$$=\int\:\:\frac{{dt}}{{t}^{\mathrm{2}} \sqrt{{t}^{\mathrm{2}} \:+\mathrm{2}{t}+\mathrm{1}−{t}−\mathrm{1}+\mathrm{1}}}\:=\int\:\:\frac{{dt}}{{t}^{\mathrm{2}} \sqrt{{t}^{\mathrm{2}} +{t}+\mathrm{1}}}\:=\int\:\:\frac{{dt}}{{t}^{\mathrm{2}} \sqrt{\left({t}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{4}}}} \\ $$$$=_{{t}+\frac{\mathrm{1}}{\mathrm{2}}=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:{sh}\left({u}\right)} \:\:\:\:\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}\int\:\:\frac{\mathrm{1}}{\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{sh}\left({u}\right)−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} {ch}\left({u}\right)}×\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{ch}\left({u}\right){du} \\ $$$$=\mathrm{4}\int\:\:\:\frac{{du}}{\left(\sqrt{\mathrm{3}}{shu}−\mathrm{1}\right)^{\mathrm{2}} }\:=\mathrm{4}\:\int\:\:\frac{{du}}{\mathrm{3}{sh}^{\mathrm{2}} \left({u}\right)−\mathrm{2}\sqrt{\mathrm{3}}{shu}\:+\mathrm{1}} \\ $$$$=\mathrm{4}\:\int\:\:\frac{{du}}{\frac{\mathrm{3}}{\mathrm{2}}\left({ch}\left(\mathrm{2}{u}\right)−\mathrm{1}\right)−\mathrm{2}\sqrt{\mathrm{3}}{shu}\:+\mathrm{1}}\:=\mathrm{8}\:\int\:\:\frac{{du}}{\mathrm{3}{ch}\left(\mathrm{2}{u}\right)−\mathrm{3}−\mathrm{4}\sqrt{\mathrm{3}}{shu}+\mathrm{2}} \\ $$$$=\mathrm{8}\:\int\:\frac{{du}}{\mathrm{3}{sh}\left(\mathrm{2}{u}\right)−\mathrm{4}\sqrt{\mathrm{3}}{sh}\left({u}\right)−\mathrm{1}}\:=\mathrm{8}\:\int\:\:\frac{{du}}{\mathrm{3}\frac{{e}^{\mathrm{2}{u}} −{e}^{−\mathrm{2}{u}} }{\mathrm{2}}−\mathrm{4}\sqrt{\mathrm{3}}×\frac{{e}^{{u}} −{e}^{−{u}} }{\mathrm{2}}−\mathrm{1}} \\ $$$$=\mathrm{16}\:\int\:\:\frac{{du}}{\mathrm{3}{e}^{\mathrm{2}{u}} −\mathrm{3}{e}^{−\mathrm{2}{u}} \:−\mathrm{4}\sqrt{\mathrm{3}}{e}^{{u}} \:+\mathrm{4}\sqrt{\mathrm{3}}{e}^{−{u}} −\mathrm{2}} \\ $$$$=_{{e}^{{u}} \:\:={z}} \:\:\mathrm{16}\:\:\int\:\:\:\:\:\frac{{dz}}{{z}\left(\mathrm{3}\:{z}^{\mathrm{2}} −\mathrm{3}{z}^{−\mathrm{2}} −\mathrm{4}\sqrt{\mathrm{3}}{z}+\mathrm{4}\sqrt{\mathrm{3}}{z}^{−\mathrm{1}} −\mathrm{2}\right)} \\ $$$$=\mathrm{16}\:\int\:\:\frac{{z}^{\mathrm{2}} \:{dz}}{{z}\left(\mathrm{3}{z}^{\mathrm{4}} −\mathrm{3}−\mathrm{4}\sqrt{\mathrm{3}}{z}^{\mathrm{3}} \:+\mathrm{4}\sqrt{\mathrm{3}}{z}\:−\mathrm{2}{z}^{\mathrm{2}} \right)} \\ $$$$=\mathrm{16}\:\int\:\:\frac{{z}^{\mathrm{2}} \:{dz}}{{z}\left(\mathrm{3}{z}^{\mathrm{4}} −\mathrm{4}\sqrt{\mathrm{3}}{z}^{\mathrm{3}} −\mathrm{2}{z}^{\mathrm{2}} \:+\mathrm{4}\sqrt{\mathrm{3}}{z}\:−\mathrm{3}\right)} \\ $$$${decomposition}\:{of}\:{F}\left({z}\right)\:=\frac{{z}^{\mathrm{2}} }{{z}\left(\mathrm{3}{z}^{\mathrm{4}} −\mathrm{4}\sqrt{\mathrm{3}}{z}^{\mathrm{3}} −\mathrm{2}{z}^{\mathrm{2}} \:+\mathrm{4}\sqrt{\mathrm{3}}{z}\:−\mathrm{3}\right)} \\ $$$$…{be}\:{continued}… \\ $$
Answered by MJS last updated on 30/Apr/20
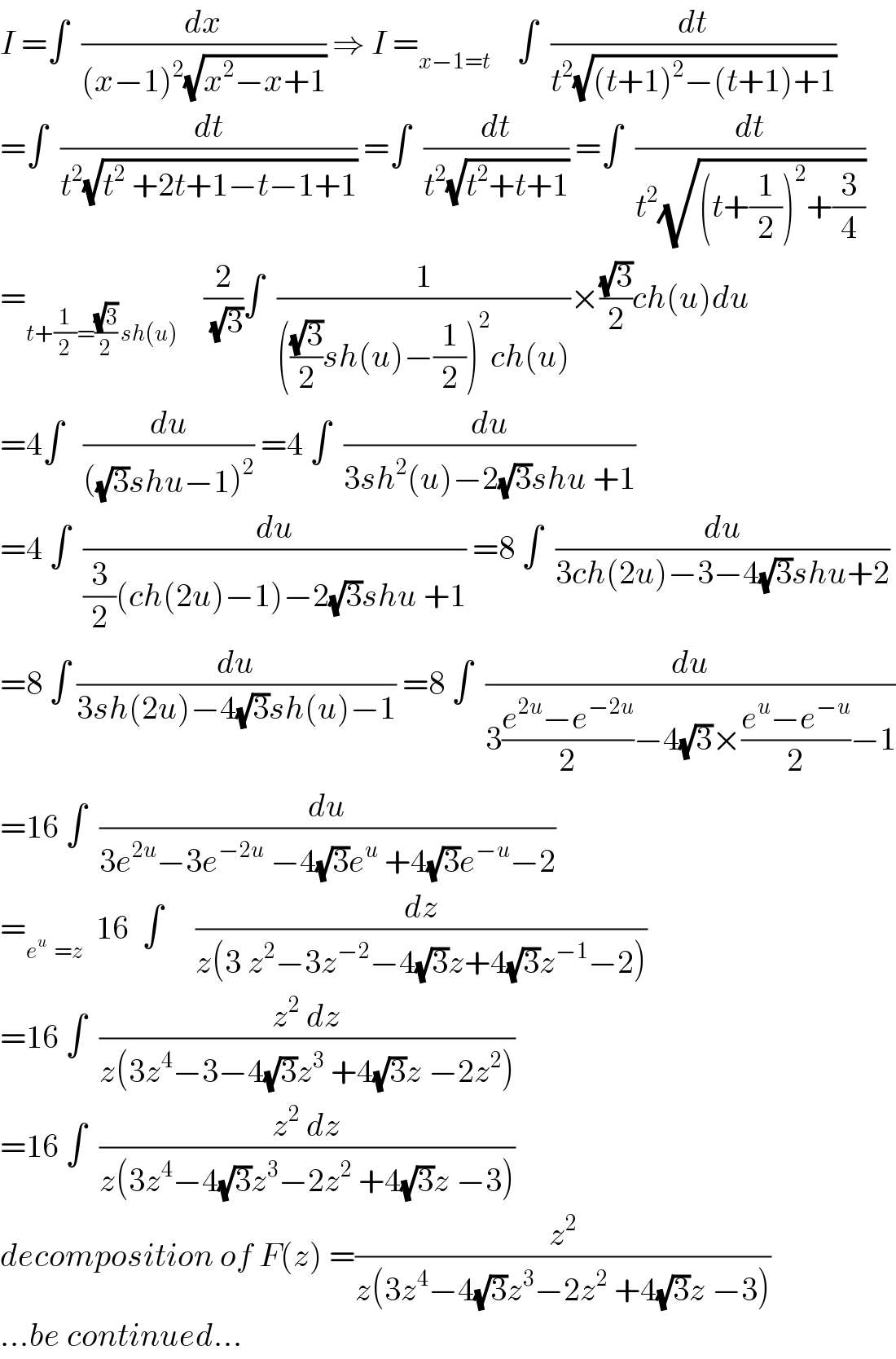
2) r=(√(x^2 −x+1)) ⇔ x=((1+(√(4r^2 −3)))/2) r=((√3)/2)cosh s s=ln t ⇒ x=(((√3)t^2 +2t−(√3))/(4t)) ⇔ t=((2x−1+2(√(x^2 −x+1)))/( (√3))) ∫(dx/((x−1)^2 (√(x^2 −x+1))))= [t=((2x−1+2(√(x^2 −x+1)))/( (√3))) → dx=(((√3)(t^2 +1))/(4t^2 ))dt] =((16)/3)∫(t/((t−(√3))^2 (t+((√3)/3))^2 ))dt= =(√3)∫(dt/((t−(√3))^2 ))−((√3)/3)∫(dt/((t+((√3)/3))^2 ))−(1/2)∫(dt/(t−(√3)))+(1/2)∫(dt/(t+((√3)/3)))= =−((√3)/(t−(√3)))+((√3)/(3t+(√3)))−(1/2)ln (t−(√3)) +(1/2)ln (t+((√3)/3)) = =−((2((√3)t+3))/((t−(√3))(3t+(√3))))+(1/2)ln ((3t+(√3))/(t−(√3))) = =−((√(x^2 −x+1))/(x−1))+(1/2)ln ((x+1+2(√(x^2 −x+1)))/(x−1)) +C
$$\left.\mathrm{2}\right) \\ $$$${r}=\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}\:\Leftrightarrow\:{x}=\frac{\mathrm{1}+\sqrt{\mathrm{4}{r}^{\mathrm{2}} −\mathrm{3}}}{\mathrm{2}} \\ $$$${r}=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{cosh}\:{s} \\ $$$${s}=\mathrm{ln}\:{t} \\ $$$$\Rightarrow\:{x}=\frac{\sqrt{\mathrm{3}}{t}^{\mathrm{2}} +\mathrm{2}{t}−\sqrt{\mathrm{3}}}{\mathrm{4}{t}}\:\Leftrightarrow\:{t}=\frac{\mathrm{2}{x}−\mathrm{1}+\mathrm{2}\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}}{\:\sqrt{\mathrm{3}}} \\ $$$$\int\frac{{dx}}{\left({x}−\mathrm{1}\right)^{\mathrm{2}} \sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}}= \\ $$$$\:\:\:\:\:\left[{t}=\frac{\mathrm{2}{x}−\mathrm{1}+\mathrm{2}\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}}{\:\sqrt{\mathrm{3}}}\:\rightarrow\:{dx}=\frac{\sqrt{\mathrm{3}}\left({t}^{\mathrm{2}} +\mathrm{1}\right)}{\mathrm{4}{t}^{\mathrm{2}} }{dt}\right] \\ $$$$=\frac{\mathrm{16}}{\mathrm{3}}\int\frac{{t}}{\left({t}−\sqrt{\mathrm{3}}\right)^{\mathrm{2}} \left({t}+\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}\right)^{\mathrm{2}} }{dt}= \\ $$$$=\sqrt{\mathrm{3}}\int\frac{{dt}}{\left({t}−\sqrt{\mathrm{3}}\right)^{\mathrm{2}} }−\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}\int\frac{{dt}}{\left({t}+\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}\right)^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{dt}}{{t}−\sqrt{\mathrm{3}}}+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{dt}}{{t}+\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}}= \\ $$$$=−\frac{\sqrt{\mathrm{3}}}{{t}−\sqrt{\mathrm{3}}}+\frac{\sqrt{\mathrm{3}}}{\mathrm{3}{t}+\sqrt{\mathrm{3}}}−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\left({t}−\sqrt{\mathrm{3}}\right)\:+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\left({t}+\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}\right)\:= \\ $$$$=−\frac{\mathrm{2}\left(\sqrt{\mathrm{3}}{t}+\mathrm{3}\right)}{\left({t}−\sqrt{\mathrm{3}}\right)\left(\mathrm{3}{t}+\sqrt{\mathrm{3}}\right)}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\frac{\mathrm{3}{t}+\sqrt{\mathrm{3}}}{{t}−\sqrt{\mathrm{3}}}\:= \\ $$$$=−\frac{\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}}{{x}−\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\frac{{x}+\mathrm{1}+\mathrm{2}\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}}{{x}−\mathrm{1}}\:+{C} \\ $$
Answered by MJS last updated on 30/Apr/20
1) ∫_0 ^π (x/(csc^3 x +cot^2 x csc x))dx= =∫_0 ^π ((xsin^3 x)/(1+cos^2 x))dx= by parts u′=((sin^3 x)/(1+cos^2 x)) → u=^((∗)) cos x −2arctan cos x v=x → v′=1 =[x(cos x −2arctan cos x)]_0 ^π −∫_0 ^π (cos x −2arctan cos x)dx= [the integral is zero!] =π((π/2)−1)
$$\left.\mathrm{1}\right) \\ $$$$\underset{\mathrm{0}} {\overset{\pi} {\int}}\frac{{x}}{\mathrm{csc}^{\mathrm{3}} \:{x}\:+\mathrm{cot}^{\mathrm{2}} \:{x}\:\mathrm{csc}\:{x}}{dx}= \\ $$$$=\underset{\mathrm{0}} {\overset{\pi} {\int}}\frac{{x}\mathrm{sin}^{\mathrm{3}} \:{x}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \:{x}}{dx}= \\ $$$$\:\:\:\:\:\mathrm{by}\:\mathrm{parts} \\ $$$$\:\:\:\:\:{u}'=\frac{\mathrm{sin}^{\mathrm{3}} \:{x}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \:{x}}\:\rightarrow\:{u}\overset{\left(\ast\right)} {=}\mathrm{cos}\:{x}\:−\mathrm{2arctan}\:\mathrm{cos}\:{x} \\ $$$$\:\:\:\:\:{v}={x}\:\rightarrow\:{v}'=\mathrm{1} \\ $$$$=\left[{x}\left(\mathrm{cos}\:{x}\:−\mathrm{2arctan}\:\mathrm{cos}\:{x}\right)\right]_{\mathrm{0}} ^{\pi} −\underset{\mathrm{0}} {\overset{\pi} {\int}}\left(\mathrm{cos}\:{x}\:−\mathrm{2arctan}\:\mathrm{cos}\:{x}\right){dx}= \\ $$$$\:\:\:\:\:\left[\mathrm{the}\:\mathrm{integral}\:\mathrm{is}\:\mathrm{zero}!\right] \\ $$$$=\pi\left(\frac{\pi}{\mathrm{2}}−\mathrm{1}\right) \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *