Question Number 93284 by naka3546 last updated on 12/May/20

Commented by mr W last updated on 12/May/20
![no unique solution! [EFGH]≥2[ABCD]=4M](https://www.tinkutara.com/question/Q93301.png)
$${no}\:{unique}\:{solution}! \\ $$$$\left[{EFGH}\right]\geqslant\mathrm{2}\left[{ABCD}\right]=\mathrm{4}{M} \\ $$
Commented by mr W last updated on 12/May/20

$${EFGH}\:{can}\:{be}\:{as}\:{large}\:{as}\:{you}\:{like}, \\ $$$${till}\:\infty. \\ $$$${Every}\:{square}\:{with}\:{vertexes}\:{on}\:{the}\: \\ $$$${lines}\:{L}_{\mathrm{1}} ,{L}_{\mathrm{2}} ,{L}_{\mathrm{3}} ,{L}_{\mathrm{4}} \:{is}\:{a}\:{solution}. \\ $$
Commented by mr W last updated on 12/May/20

Commented by naka3546 last updated on 12/May/20

$${thank}\:\:{you}\:,\:\:{sir}. \\ $$$${The}\:\:{options}\:\:{are}\:\:\mathrm{6}{M}\:\:{and}\:\:\mathrm{8}{M}\:\: \\ $$
Commented by mr W last updated on 12/May/20
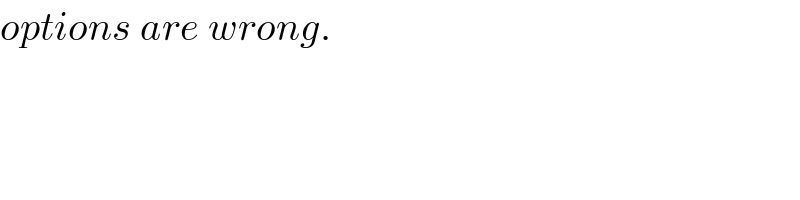
$${options}\:{are}\:{wrong}. \\ $$
