Question Number 93991 by i jagooll last updated on 16/May/20

Commented by i jagooll last updated on 16/May/20

$$\mathrm{find}\:\mathrm{the}\:\mathrm{shaded}\:\mathrm{area} \\ $$$$ \\ $$
Commented by i jagooll last updated on 16/May/20
$$\mathrm{anyone}\:\mathrm{help}\:\mathrm{me}\:\mathrm{this}\:\mathrm{question} \\ $$
Commented by Tony Lin last updated on 16/May/20
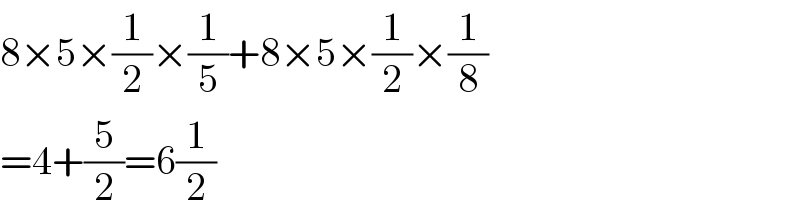
$$\mathrm{8}×\mathrm{5}×\frac{\mathrm{1}}{\mathrm{2}}×\frac{\mathrm{1}}{\mathrm{5}}+\mathrm{8}×\mathrm{5}×\frac{\mathrm{1}}{\mathrm{2}}×\frac{\mathrm{1}}{\mathrm{8}} \\ $$$$=\mathrm{4}+\frac{\mathrm{5}}{\mathrm{2}}=\mathrm{6}\frac{\mathrm{1}}{\mathrm{2}} \\ $$
Commented by Kunal12588 last updated on 16/May/20
sir Tony, your explanation will be appreciated. pls
Commented by i jagooll last updated on 16/May/20

$$\mathrm{sir}\:\mathrm{Tony}\:\mathrm{how}\:\mathrm{this}\:\mathrm{formula}? \\ $$
Commented by Tony Lin last updated on 16/May/20

Commented by i jagooll last updated on 16/May/20

$$\mathrm{next}\:\mathrm{sir}?\:\mathrm{please} \\ $$
Commented by Kunal12588 last updated on 16/May/20
8×5 is area of rect., ×1/2 is of triangle but why ×1/5 and ×1/8?
Commented by Tony Lin last updated on 16/May/20
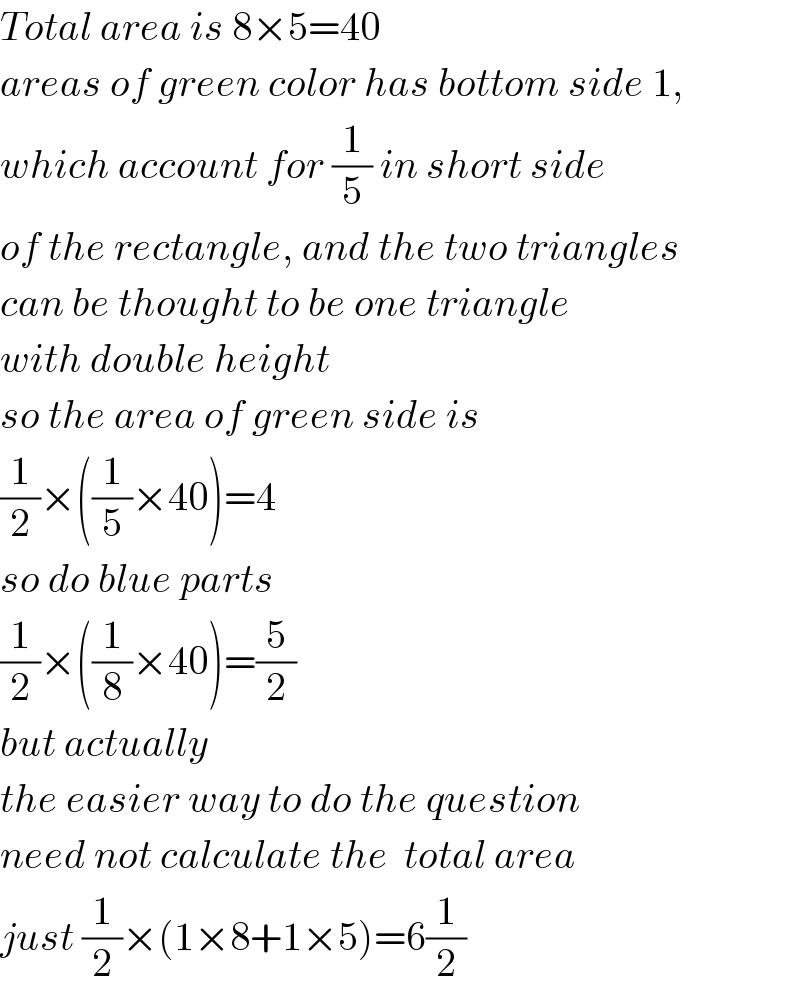
$${Total}\:{area}\:{is}\:\mathrm{8}×\mathrm{5}=\mathrm{40} \\ $$$${areas}\:{of}\:{green}\:{color}\:{has}\:{bottom}\:{side}\:\mathrm{1}, \\ $$$${which}\:{account}\:{for}\:\frac{\mathrm{1}}{\mathrm{5}}\:{in}\:{short}\:{side} \\ $$$${of}\:{the}\:{rectangle},\:{and}\:{the}\:{two}\:{triangles} \\ $$$${can}\:{be}\:{thought}\:{to}\:{be}\:{one}\:{triangle}\: \\ $$$${with}\:{double}\:{height} \\ $$$${so}\:{the}\:{area}\:{of}\:{green}\:{side}\:{is} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}×\left(\frac{\mathrm{1}}{\mathrm{5}}×\mathrm{40}\right)=\mathrm{4} \\ $$$${so}\:{do}\:{blue}\:{parts} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}×\left(\frac{\mathrm{1}}{\mathrm{8}}×\mathrm{40}\right)=\frac{\mathrm{5}}{\mathrm{2}} \\ $$$${but}\:{actually} \\ $$$${the}\:{easier}\:{way}\:{to}\:{do}\:{the}\:{question} \\ $$$${need}\:{not}\:{calculate}\:{the}\:\:{total}\:{area}\: \\ $$$${just}\:\frac{\mathrm{1}}{\mathrm{2}}×\left(\mathrm{1}×\mathrm{8}+\mathrm{1}×\mathrm{5}\right)=\mathrm{6}\frac{\mathrm{1}}{\mathrm{2}} \\ $$
Commented by Kunal12588 last updated on 16/May/20
thanks sir, and yes second method is much easier, and easy to understand.
Commented by i jagooll last updated on 16/May/20
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{mr}\:\mathrm{Toni} \\ $$
Answered by mr W last updated on 16/May/20

Commented by mr W last updated on 16/May/20
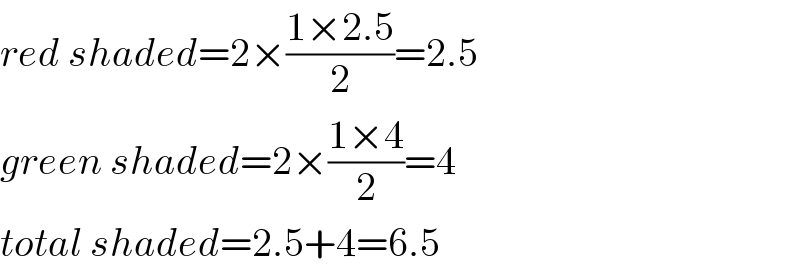
$${red}\:{shaded}=\mathrm{2}×\frac{\mathrm{1}×\mathrm{2}.\mathrm{5}}{\mathrm{2}}=\mathrm{2}.\mathrm{5} \\ $$$${green}\:{shaded}=\mathrm{2}×\frac{\mathrm{1}×\mathrm{4}}{\mathrm{2}}=\mathrm{4} \\ $$$${total}\:{shaded}=\mathrm{2}.\mathrm{5}+\mathrm{4}=\mathrm{6}.\mathrm{5} \\ $$
Commented by i jagooll last updated on 16/May/20

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{mr}\:\mathrm{W} \\ $$
