Question Number 94523 by peter frank last updated on 19/May/20
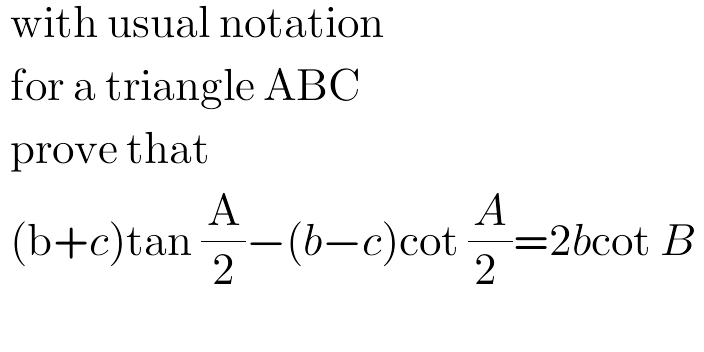
Commented by PRITHWISH SEN 2 last updated on 19/May/20
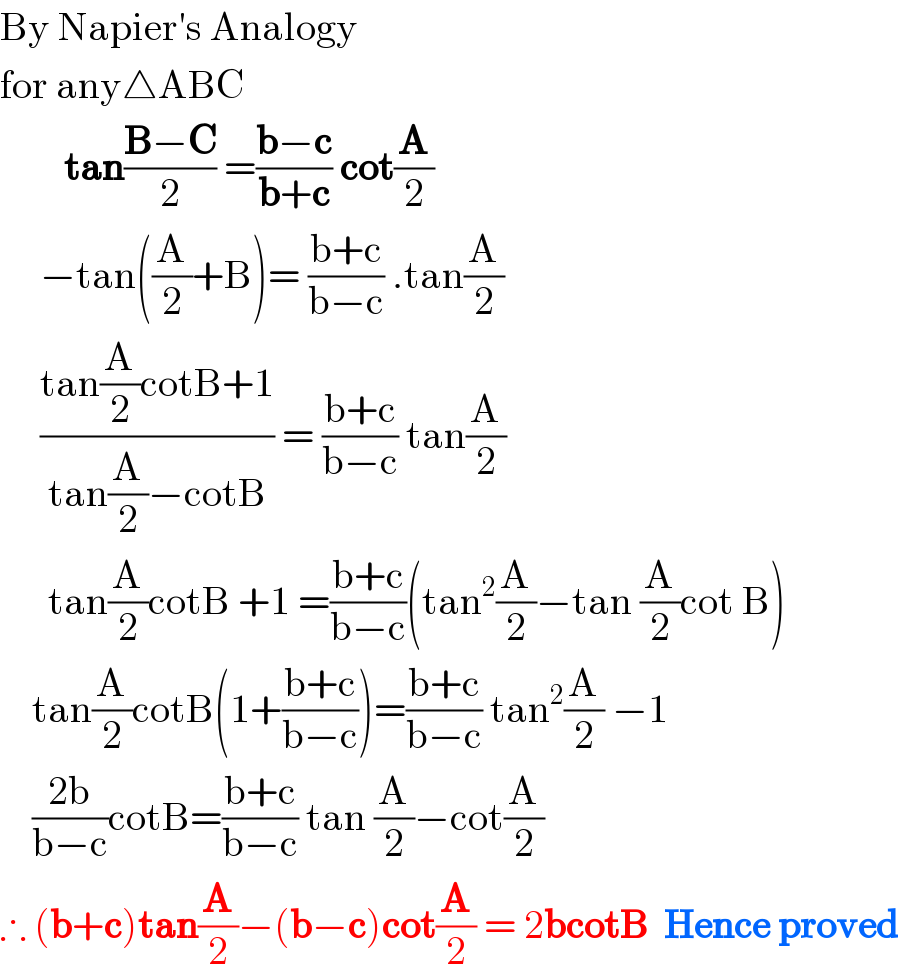
$$\mathrm{By}\:\mathrm{Napier}'\mathrm{s}\:\mathrm{Analogy}\: \\ $$$$\mathrm{for}\:\mathrm{any}\bigtriangleup\mathrm{ABC} \\ $$$$\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{tan}}\frac{\boldsymbol{\mathrm{B}}−\boldsymbol{\mathrm{C}}}{\mathrm{2}}\:=\frac{\boldsymbol{\mathrm{b}}−\boldsymbol{\mathrm{c}}}{\boldsymbol{\mathrm{b}}+\boldsymbol{\mathrm{c}}}\:\boldsymbol{\mathrm{cot}}\frac{\boldsymbol{\mathrm{A}}}{\mathrm{2}} \\ $$$$\:\:\:\:\:−\mathrm{tan}\left(\frac{\mathrm{A}}{\mathrm{2}}+\mathrm{B}\right)=\:\frac{\mathrm{b}+\mathrm{c}}{\mathrm{b}−\mathrm{c}}\:.\mathrm{tan}\frac{\mathrm{A}}{\mathrm{2}} \\ $$$$\:\:\:\:\:\frac{\mathrm{tan}\frac{\mathrm{A}}{\mathrm{2}}\mathrm{cotB}+\mathrm{1}}{\mathrm{tan}\frac{\mathrm{A}}{\mathrm{2}}−\mathrm{cotB}}\:=\:\frac{\mathrm{b}+\mathrm{c}}{\mathrm{b}−\mathrm{c}}\:\mathrm{tan}\frac{\mathrm{A}}{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\mathrm{tan}\frac{\mathrm{A}}{\mathrm{2}}\mathrm{cotB}\:+\mathrm{1}\:=\frac{\mathrm{b}+\mathrm{c}}{\mathrm{b}−\mathrm{c}}\left(\mathrm{tan}^{\mathrm{2}} \frac{\mathrm{A}}{\mathrm{2}}−\mathrm{tan}\:\frac{\mathrm{A}}{\mathrm{2}}\mathrm{cot}\:\mathrm{B}\right) \\ $$$$\:\:\:\:\mathrm{tan}\frac{\mathrm{A}}{\mathrm{2}}\mathrm{cotB}\left(\mathrm{1}+\frac{\mathrm{b}+\mathrm{c}}{\mathrm{b}−\mathrm{c}}\right)=\frac{\mathrm{b}+\mathrm{c}}{\mathrm{b}−\mathrm{c}}\:\mathrm{tan}^{\mathrm{2}} \frac{\mathrm{A}}{\mathrm{2}}\:−\mathrm{1} \\ $$$$\:\:\:\:\frac{\mathrm{2b}}{\mathrm{b}−\mathrm{c}}\mathrm{cotB}=\frac{\mathrm{b}+\mathrm{c}}{\mathrm{b}−\mathrm{c}}\:\mathrm{tan}\:\frac{\mathrm{A}}{\mathrm{2}}−\mathrm{cot}\frac{\mathrm{A}}{\mathrm{2}} \\ $$$$\therefore\:\left(\boldsymbol{\mathrm{b}}+\boldsymbol{\mathrm{c}}\right)\boldsymbol{\mathrm{tan}}\frac{\boldsymbol{\mathrm{A}}}{\mathrm{2}}−\left(\boldsymbol{\mathrm{b}}−\boldsymbol{\mathrm{c}}\right)\boldsymbol{\mathrm{cot}}\frac{\boldsymbol{\mathrm{A}}}{\mathrm{2}}\:=\:\mathrm{2}\boldsymbol{\mathrm{bcotB}}\:\:\boldsymbol{\mathrm{Hence}}\:\boldsymbol{\mathrm{proved}} \\ $$
