Question Number 94922 by peter frank last updated on 21/May/20

Answered by mr W last updated on 22/May/20
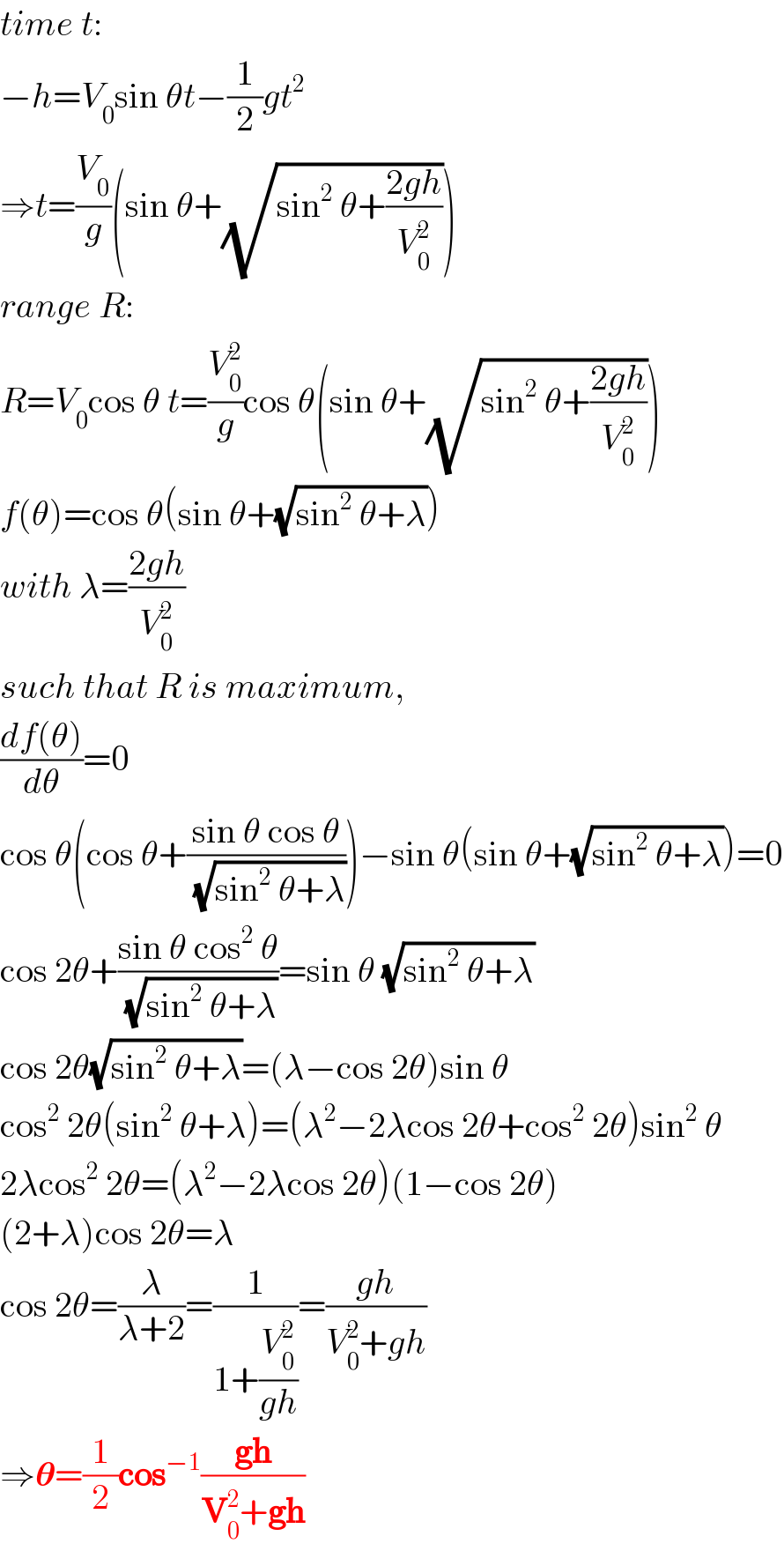
$${time}\:{t}: \\ $$$$−{h}={V}_{\mathrm{0}} \mathrm{sin}\:\theta{t}−\frac{\mathrm{1}}{\mathrm{2}}{gt}^{\mathrm{2}} \\ $$$$\Rightarrow{t}=\frac{{V}_{\mathrm{0}} }{{g}}\left(\mathrm{sin}\:\theta+\sqrt{\mathrm{sin}^{\mathrm{2}} \:\theta+\frac{\mathrm{2}{gh}}{{V}_{\mathrm{0}} ^{\mathrm{2}} }}\right) \\ $$$${range}\:{R}: \\ $$$${R}={V}_{\mathrm{0}} \mathrm{cos}\:\theta\:{t}=\frac{{V}_{\mathrm{0}} ^{\mathrm{2}} }{{g}}\mathrm{cos}\:\theta\left(\mathrm{sin}\:\theta+\sqrt{\mathrm{sin}^{\mathrm{2}} \:\theta+\frac{\mathrm{2}{gh}}{{V}_{\mathrm{0}} ^{\mathrm{2}} }}\right) \\ $$$${f}\left(\theta\right)=\mathrm{cos}\:\theta\left(\mathrm{sin}\:\theta+\sqrt{\mathrm{sin}^{\mathrm{2}} \:\theta+\lambda}\right) \\ $$$${with}\:\lambda=\frac{\mathrm{2}{gh}}{{V}_{\mathrm{0}} ^{\mathrm{2}} } \\ $$$${such}\:{that}\:{R}\:{is}\:{maximum}, \\ $$$$\frac{{df}\left(\theta\right)}{{d}\theta}=\mathrm{0} \\ $$$$\mathrm{cos}\:\theta\left(\mathrm{cos}\:\theta+\frac{\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta}{\:\sqrt{\mathrm{sin}^{\mathrm{2}} \:\theta+\lambda}}\right)−\mathrm{sin}\:\theta\left(\mathrm{sin}\:\theta+\sqrt{\mathrm{sin}^{\mathrm{2}} \:\theta+\lambda}\right)=\mathrm{0} \\ $$$$\mathrm{cos}\:\mathrm{2}\theta+\frac{\mathrm{sin}\:\theta\:\mathrm{cos}^{\mathrm{2}} \:\theta}{\:\sqrt{\mathrm{sin}^{\mathrm{2}} \:\theta+\lambda}}=\mathrm{sin}\:\theta\:\sqrt{\mathrm{sin}^{\mathrm{2}} \:\theta+\lambda} \\ $$$$\mathrm{cos}\:\mathrm{2}\theta\sqrt{\mathrm{sin}^{\mathrm{2}} \:\theta+\lambda}=\left(\lambda−\mathrm{cos}\:\mathrm{2}\theta\right)\mathrm{sin}\:\theta \\ $$$$\mathrm{cos}^{\mathrm{2}} \:\mathrm{2}\theta\left(\mathrm{sin}^{\mathrm{2}} \:\theta+\lambda\right)=\left(\lambda^{\mathrm{2}} −\mathrm{2}\lambda\mathrm{cos}\:\mathrm{2}\theta+\mathrm{cos}^{\mathrm{2}} \:\mathrm{2}\theta\right)\mathrm{sin}^{\mathrm{2}} \:\theta \\ $$$$\mathrm{2}\lambda\mathrm{cos}^{\mathrm{2}} \:\mathrm{2}\theta=\left(\lambda^{\mathrm{2}} −\mathrm{2}\lambda\mathrm{cos}\:\mathrm{2}\theta\right)\left(\mathrm{1}−\mathrm{cos}\:\mathrm{2}\theta\right) \\ $$$$\left(\mathrm{2}+\lambda\right)\mathrm{cos}\:\mathrm{2}\theta=\lambda \\ $$$$\mathrm{cos}\:\mathrm{2}\theta=\frac{\lambda}{\lambda+\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{1}+\frac{{V}_{\mathrm{0}} ^{\mathrm{2}} }{{gh}}}=\frac{{gh}}{{V}_{\mathrm{0}} ^{\mathrm{2}} +{gh}} \\ $$$$\Rightarrow\boldsymbol{\theta}=\frac{\mathrm{1}}{\mathrm{2}}\boldsymbol{\mathrm{cos}}^{−\mathrm{1}} \frac{\boldsymbol{\mathrm{gh}}}{\boldsymbol{\mathrm{V}}_{\mathrm{0}} ^{\mathrm{2}} +\boldsymbol{\mathrm{gh}}} \\ $$
Commented by peter frank last updated on 24/May/20
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir} \\ $$
