Question-95767

Question Number 95767 by PengagumRahasiamu last updated on 27/May/20

Answered by prakash jain last updated on 27/May/20

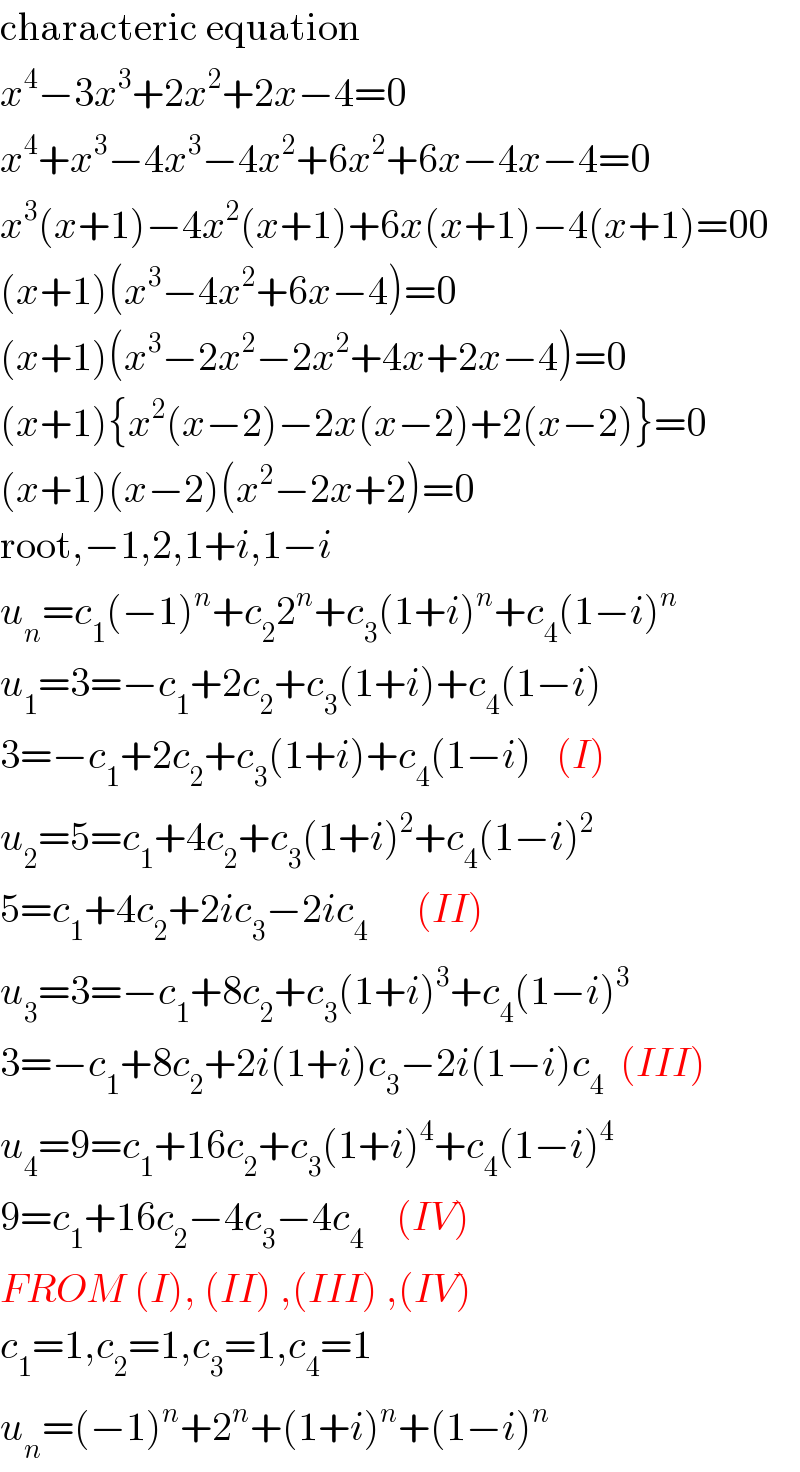
characteric equation

x^{4} - 3 x^{3} + 2 x^{2} + 2 x - 4 = 0

x^{4} + x^{3} - 4 x^{3} - 4 x^{2} + 6 x^{2} + 6 x - 4 x - 4 = 0

x^{3} (x + 1) - 4 x^{2} (x + 1) + 6 x (x + 1) - 4 (x + 1) = 00

(x + 1) (x^{3} - 4 x^{2} + 6 x - 4) = 0

(x + 1) (x^{3} - 2 x^{2} - 2 x^{2} + 4 x + 2 x - 4) = 0

(x + 1) {x^{2} (x - 2) - 2 x (x - 2) + 2 (x - 2)} = 0

(x + 1) (x - 2) (x^{2} - 2 x + 2) = 0

root, - 1, 2, 1 + i, 1 - i

u_{n} = c_{1} {(- 1)}^{n} + c_{2} 2^{n} + c_{3} {(1 + i)}^{n} + c_{4} {(1 - i)}^{n}

u_{1} = 3 = - c_{1} + 2 c_{2} + c_{3} (1 + i) + c_{4} (1 - i)

3 = - c_{1} + 2 c_{2} + c_{3} (1 + i) + c_{4} (1 - i) (I)

u_{2} = 5 = c_{1} + 4 c_{2} + c_{3} {(1 + i)}^{2} + c_{4} {(1 - i)}^{2}

5 = c_{1} + 4 c_{2} + 2 {i c}_{3} - 2 {i c}_{4} (I I)

u_{3} = 3 = - c_{1} + 8 c_{2} + c_{3} {(1 + i)}^{3} + c_{4} {(1 - i)}^{3}

3 = - c_{1} + 8 c_{2} + 2 i (1 + i) c_{3} - 2 i (1 - i) c_{4} (I I I)

u_{4} = 9 = c_{1} + 16 c_{2} + c_{3} {(1 + i)}^{4} + c_{4} {(1 - i)}^{4}

9 = c_{1} + 16 c_{2} - 4 c_{3} - 4 c_{4} (I V)

F R O M (I), (I I), (I I I), (I V)

c_{1} = 1, c_{2} = 1, c_{3} = 1, c_{4} = 1

u_{n} = {(- 1)}^{n} + 2^{n} + {(1 + i)}^{n} + {(1 - i)}^{n}

Commented by bobhans last updated on 27/May/20

great

Commented by PengagumRahasiamu last updated on 21/Jul/20

Thank you Sir