Question Number 97454 by bemath last updated on 08/Jun/20
Answered by john santu last updated on 08/Jun/20
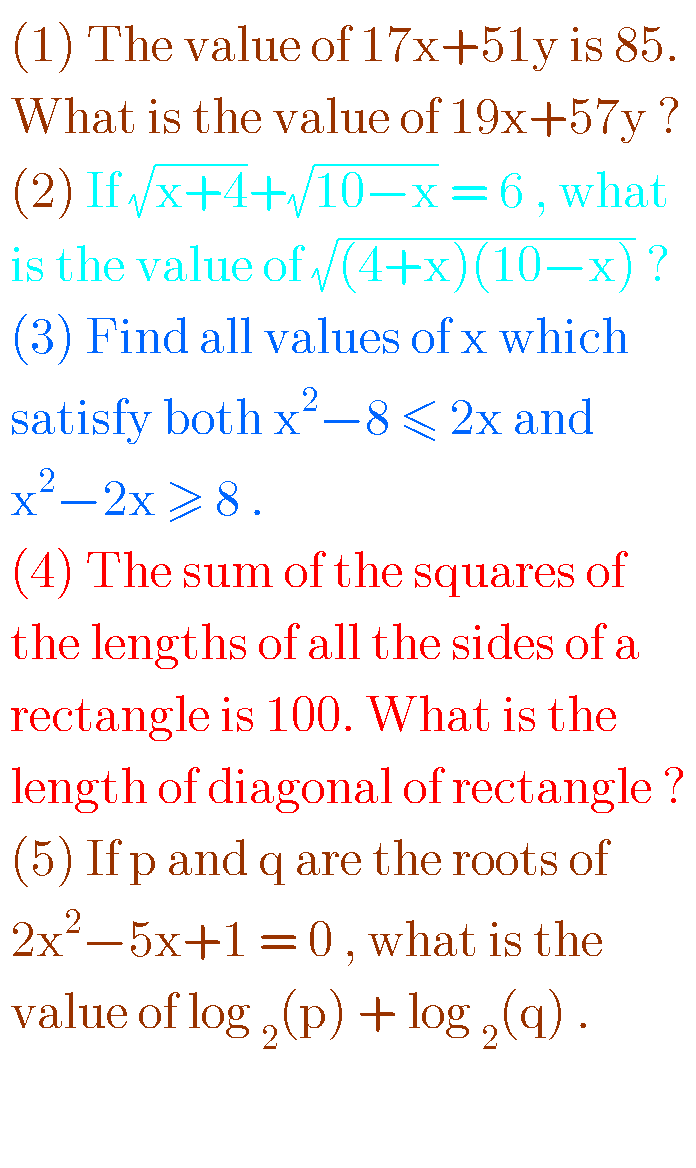
![(1)17x+51y=85 [ : 17 ] ⇒ x+3y = 5 ⇒ 19x+57y = 19×5=95 (2) (√(10−x)) = 6−(√(4+x)) (square) 15+x = 6(√(4+x)) [ square ] x^2 −6x+81 = 0 . Then (√((4+x)(10−x))) = (√(121−(x^2 −6x+81))) = 11 (3)the first inequality is equivalent to x^2 −2x−8 ≤ 0, while the second is equivalent to x^2 −2x−8≥0 since their intersection is x^2 −2x−8 = 0 , or (x+2)(x−4)=0 it follows that x = −2 ; 4 (4) Let a and b represent the length and width of rectangle Then 2a^2 +2b^2 =100 or a^2 +b^2 =50 The length of diagonal is (√(a^2 +b^2 )) = 5(√2) (5)since both p and q are positive, log _2 (p)+log _2 (q) = log _2 (pq) but p &q are roots of the quadratic equation 2x^2 −5x+1=0 , so pq = (1/2) , such that log _2 (pq)=log _2 ((1/2)) = −1](https://www.tinkutara.com/question/Q97455.png)
$$\left(\mathrm{1}\right)\mathrm{17x}+\mathrm{51y}=\mathrm{85}\:\left[\::\:\mathrm{17}\:\right]\: \\ $$$$\Rightarrow\:\mathrm{x}+\mathrm{3y}\:=\:\mathrm{5}\:\Rightarrow\:\mathrm{19x}+\mathrm{57y}\:=\:\mathrm{19}×\mathrm{5}=\mathrm{95} \\ $$$$\left(\mathrm{2}\right)\:\sqrt{\mathrm{10}−\mathrm{x}}\:=\:\mathrm{6}−\sqrt{\mathrm{4}+\mathrm{x}}\:\left(\mathrm{square}\right) \\ $$$$\mathrm{15}+\mathrm{x}\:=\:\mathrm{6}\sqrt{\mathrm{4}+\mathrm{x}}\:\left[\:\mathrm{square}\:\right] \\ $$$$\mathrm{x}^{\mathrm{2}} −\mathrm{6x}+\mathrm{81}\:=\:\mathrm{0}\:.\:\mathrm{Then}\: \\ $$$$\sqrt{\left(\mathrm{4}+\mathrm{x}\right)\left(\mathrm{10}−\mathrm{x}\right)}\:=\:\sqrt{\mathrm{121}−\left(\mathrm{x}^{\mathrm{2}} −\mathrm{6x}+\mathrm{81}\right)}\:=\:\mathrm{11} \\ $$$$\left(\mathrm{3}\right)\mathrm{the}\:\mathrm{first}\:\mathrm{inequality}\:\mathrm{is}\:\mathrm{equivalent} \\ $$$$\mathrm{to}\:\mathrm{x}^{\mathrm{2}} −\mathrm{2x}−\mathrm{8}\:\leqslant\:\mathrm{0},\:\mathrm{while}\:\mathrm{the}\:\mathrm{second} \\ $$$$\mathrm{is}\:\mathrm{equivalent}\:\mathrm{to}\:\mathrm{x}^{\mathrm{2}} −\mathrm{2x}−\mathrm{8}\geqslant\mathrm{0} \\ $$$$\mathrm{since}\:\mathrm{their}\:\mathrm{intersection}\:\mathrm{is} \\ $$$$\mathrm{x}^{\mathrm{2}} −\mathrm{2x}−\mathrm{8}\:=\:\mathrm{0}\:,\:\mathrm{or}\:\left(\mathrm{x}+\mathrm{2}\right)\left(\mathrm{x}−\mathrm{4}\right)=\mathrm{0} \\ $$$$\mathrm{it}\:\mathrm{follows}\:\mathrm{that}\:\mathrm{x}\:=\:−\mathrm{2}\:;\:\mathrm{4}\: \\ $$$$\left(\mathrm{4}\right)\:\mathrm{Let}\:{a}\:\mathrm{and}\:{b}\:\mathrm{represent}\:\mathrm{the}\: \\ $$$$\mathrm{length}\:\mathrm{and}\:\mathrm{width}\:\mathrm{of}\:\mathrm{rectangle}\: \\ $$$$\mathrm{Then}\:\mathrm{2}{a}^{\mathrm{2}} +\mathrm{2}{b}^{\mathrm{2}} =\mathrm{100}\:\mathrm{or}\:{a}^{\mathrm{2}} +{b}^{\mathrm{2}} =\mathrm{50} \\ $$$$\mathrm{The}\:\mathrm{length}\:\mathrm{of}\:\mathrm{diagonal}\:\mathrm{is}\:\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }\:=\:\mathrm{5}\sqrt{\mathrm{2}}\: \\ $$$$\left(\mathrm{5}\right)\mathrm{since}\:\mathrm{both}\:\mathrm{p}\:\mathrm{and}\:\mathrm{q}\:\mathrm{are}\:\mathrm{positive}, \\ $$$$\mathrm{log}\:_{\mathrm{2}} \left(\mathrm{p}\right)+\mathrm{log}\:_{\mathrm{2}} \left(\mathrm{q}\right)\:=\:\mathrm{log}\:_{\mathrm{2}} \left(\mathrm{pq}\right) \\ $$$$\mathrm{but}\:\mathrm{p}\:\&\mathrm{q}\:\mathrm{are}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{quadratic} \\ $$$$\mathrm{equation}\:\mathrm{2x}^{\mathrm{2}} −\mathrm{5x}+\mathrm{1}=\mathrm{0}\:,\:\mathrm{so}\: \\ $$$$\mathrm{pq}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\:,\:\mathrm{such}\:\mathrm{that}\:\mathrm{log}\:_{\mathrm{2}} \left(\mathrm{pq}\right)=\mathrm{log}\:_{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)\:=\:−\mathrm{1} \\ $$
Commented by bemath last updated on 08/Jun/20
coolll
Answered by som(math1967) last updated on 08/Jun/20

$$\mathrm{1}.\mathrm{17x}+\mathrm{51y}=\mathrm{85} \\ $$$$\Rightarrow\mathrm{17}\left(\mathrm{x}+\mathrm{3y}\right)=\mathrm{85} \\ $$$$\Rightarrow\mathrm{x}+\mathrm{3y}=\mathrm{5} \\ $$$$\Rightarrow\mathrm{19x}+\mathrm{57y}=\mathrm{95}\:\mathrm{ans} \\ $$
