Question Number 97891 by pranesh last updated on 10/Jun/20
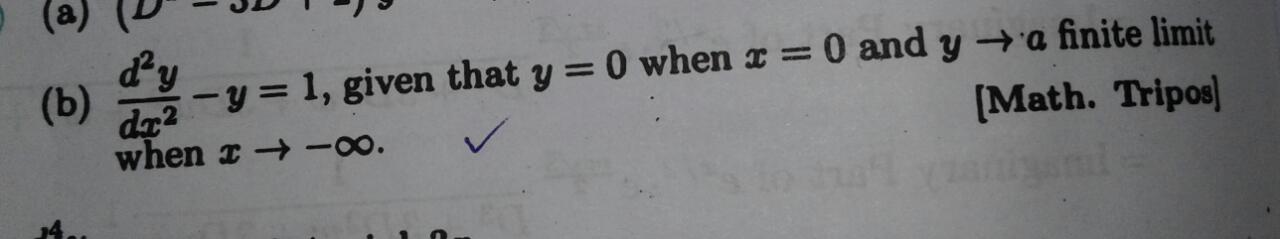
Commented by mr W last updated on 10/Jun/20

Commented by bemath last updated on 10/Jun/20
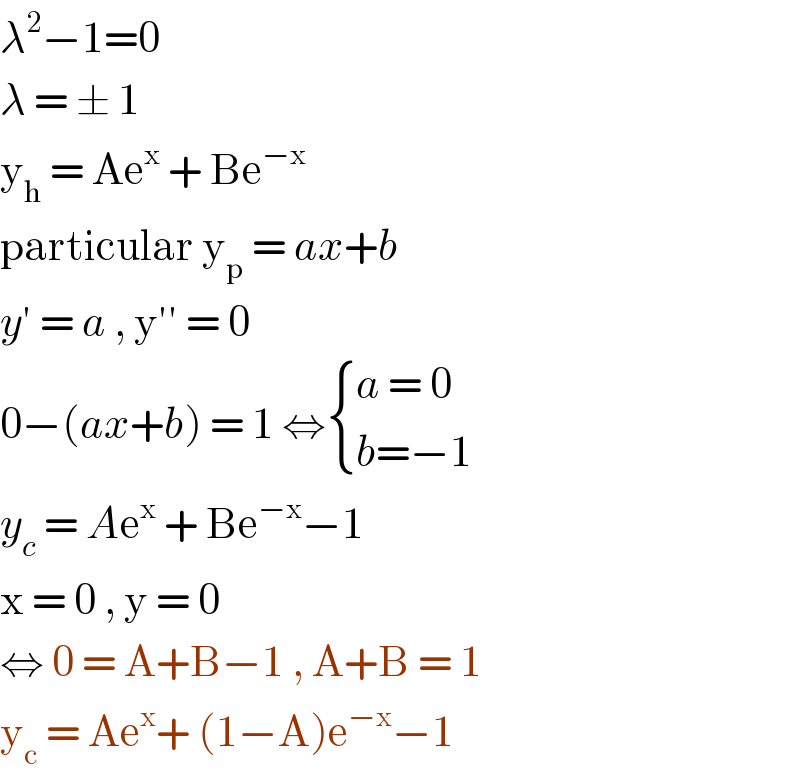
Commented by mr W last updated on 10/Jun/20
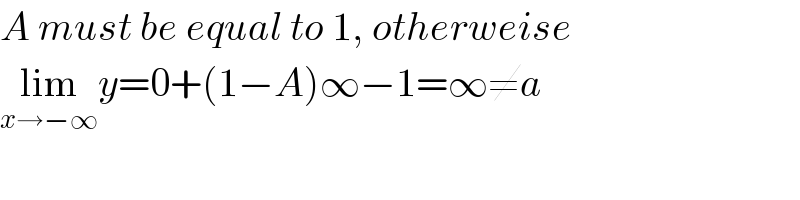
Commented by bemath last updated on 10/Jun/20
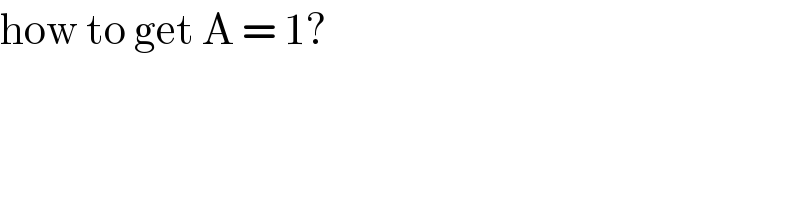
Commented by mr W last updated on 10/Jun/20
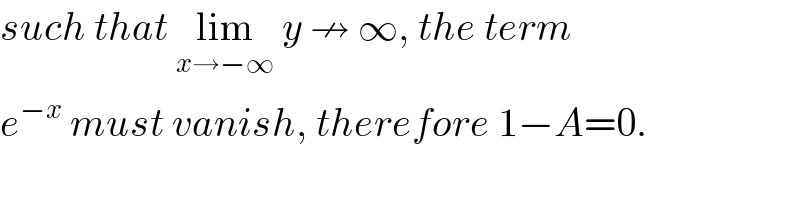
Commented by bemath last updated on 10/Jun/20