Question Number 98201 by pranesh last updated on 12/Jun/20

Answered by Rio Michael last updated on 12/Jun/20
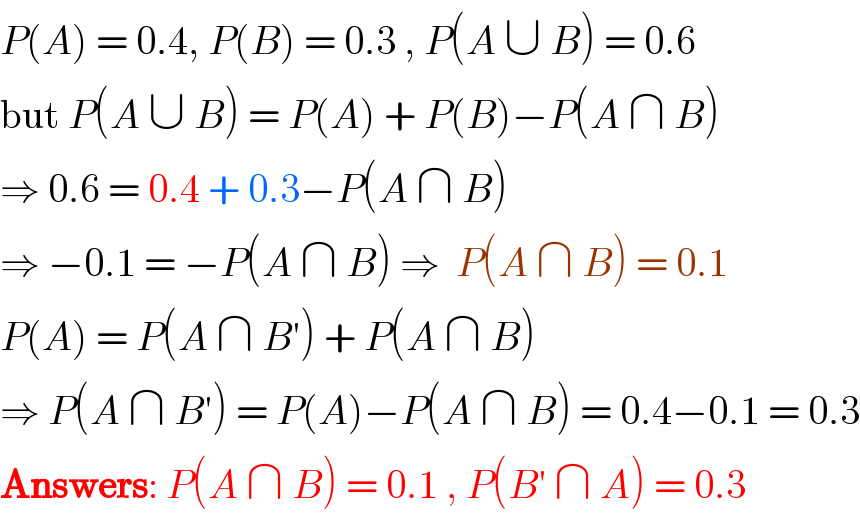
$${P}\left({A}\right)\:=\:\mathrm{0}.\mathrm{4},\:{P}\left({B}\right)\:=\:\mathrm{0}.\mathrm{3}\:,\:{P}\left({A}\:\cup\:{B}\right)\:=\:\mathrm{0}.\mathrm{6} \\ $$$$\mathrm{but}\:{P}\left({A}\:\cup\:{B}\right)\:=\:{P}\left({A}\right)\:+\:{P}\left({B}\right)−{P}\left({A}\:\cap\:{B}\right) \\ $$$$\Rightarrow\:\mathrm{0}.\mathrm{6}\:=\:\mathrm{0}.\mathrm{4}\:+\:\mathrm{0}.\mathrm{3}−{P}\left({A}\:\cap\:{B}\right) \\ $$$$\Rightarrow\:−\mathrm{0}.\mathrm{1}\:=\:−{P}\left({A}\:\cap\:{B}\right)\:\Rightarrow\:\:{P}\left({A}\:\cap\:{B}\right)\:=\:\mathrm{0}.\mathrm{1} \\ $$$${P}\left({A}\right)\:=\:{P}\left({A}\:\cap\:{B}'\right)\:+\:{P}\left({A}\:\cap\:{B}\right) \\ $$$$\Rightarrow\:{P}\left({A}\:\cap\:{B}'\right)\:=\:{P}\left({A}\right)−{P}\left({A}\:\cap\:{B}\right)\:=\:\mathrm{0}.\mathrm{4}−\mathrm{0}.\mathrm{1}\:=\:\mathrm{0}.\mathrm{3} \\ $$$$\boldsymbol{\mathrm{Answers}}:\:{P}\left({A}\:\cap\:{B}\right)\:=\:\mathrm{0}.\mathrm{1}\:,\:{P}\left({B}'\:\cap\:{A}\right)\:=\:\mathrm{0}.\mathrm{3} \\ $$
