Question Number 127160 by kaivan.ahmadi last updated on 27/Dec/20
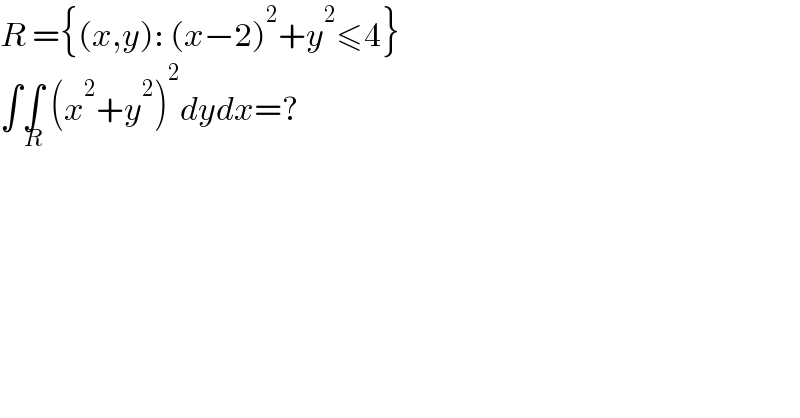
$${R}\:=\left\{\left({x},{y}\right):\:\left({x}−\mathrm{2}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} \leqslant\mathrm{4}\right\} \\ $$$$\int\underset{{R}} {\int}\:\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} \right)^{\mathrm{2}} {dydx}=? \\ $$
Answered by mathmax by abdo last updated on 27/Dec/20
![we use the diffeomorphism { ((x−2=rcosθ)),((y=rsinθ)) :} (x−2)^2 +y^2 ≤4 ⇒r^2 ≤4 ⇒0≤r≤2 ∫∫_R (x^2 +y^2 )^2 dxdy =∫_0 ^2 ∫_(−π) ^π ((rcosθ +2)^2 +r^2 sin^2 θ)^2 rdrdθ = ∫_0 ^2 ∫_(−π) ^π {r^2 cos^2 θ +4rcosθ +4+r^2 sin^2 θ)^2 drdθ = ∫_0 ^2 ∫_(−π) ^π {r^2 +4rcosθ +4)^2 drdθ =∫_0 ^2 ∫_(−π) ^π {(r^2 +4)^2 +8rcosθ(r^2 +4)+16r^2 cos^2 θ}drdθ =2π ∫_0 ^2 (r^2 +4)^(2 ) dr +8 ∫_0 ^2 (r^3 +4r)dr∫_(−π) ^π cosθ dθ(→0)+16∫_0 ^2 r^2 dr∫_(−π) ^π cos^2 θ dθ ∫_0 ^2 (r^2 +4)^2 dr =∫_0 ^2 (r^4 +8r^2 +16)dr =[(r^5 /5)+(8/3)r^3 +16r]_0 ^2 =.... ∫_0 ^2 r^2 dr =[(r^3 /3)]_0 ^2 =(8/3) ∫_(−π) ^π cos^2 θ dθ =2∫_0 ^π ((1+cos(2θ))/2)dθ =π rest to collect the values...](https://www.tinkutara.com/question/Q127183.png)
$$\mathrm{we}\:\mathrm{use}\:\mathrm{the}\:\mathrm{diffeomorphism}\:\:\begin{cases}{\mathrm{x}−\mathrm{2}=\mathrm{rcos}\theta}\\{\mathrm{y}=\mathrm{rsin}\theta}\end{cases} \\ $$$$\left(\mathrm{x}−\mathrm{2}\right)^{\mathrm{2}} \:+\mathrm{y}^{\mathrm{2}} \:\leqslant\mathrm{4}\:\Rightarrow\mathrm{r}^{\mathrm{2}} \:\leqslant\mathrm{4}\:\Rightarrow\mathrm{0}\leqslant\mathrm{r}\leqslant\mathrm{2} \\ $$$$\int\int_{\mathrm{R}} \left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{y}^{\mathrm{2}} \right)^{\mathrm{2}} \mathrm{dxdy}\:=\int_{\mathrm{0}} ^{\mathrm{2}} \:\int_{−\pi} ^{\pi} \left(\left(\mathrm{rcos}\theta\:+\mathrm{2}\right)^{\mathrm{2}} \:+\mathrm{r}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \theta\right)^{\mathrm{2}} \:\mathrm{rdrd}\theta \\ $$$$=\:\int_{\mathrm{0}} ^{\mathrm{2}} \int_{−\pi} ^{\pi} \left\{\mathrm{r}^{\mathrm{2}} \:\mathrm{cos}^{\mathrm{2}} \theta\:+\mathrm{4rcos}\theta\:+\mathrm{4}+\mathrm{r}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \theta\right)^{\mathrm{2}} \mathrm{drd}\theta \\ $$$$=\:\int_{\mathrm{0}} ^{\mathrm{2}} \int_{−\pi} ^{\pi} \left\{\mathrm{r}^{\mathrm{2}} \:+\mathrm{4rcos}\theta\:+\mathrm{4}\right)^{\mathrm{2}} \mathrm{drd}\theta\:\: \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{2}} \:\int_{−\pi} ^{\pi} \left\{\left(\mathrm{r}^{\mathrm{2}} \:+\mathrm{4}\right)^{\mathrm{2}} \:+\mathrm{8rcos}\theta\left(\mathrm{r}^{\mathrm{2}} +\mathrm{4}\right)+\mathrm{16r}^{\mathrm{2}} \:\mathrm{cos}^{\mathrm{2}} \theta\right\}\mathrm{drd}\theta \\ $$$$=\mathrm{2}\pi\:\int_{\mathrm{0}} ^{\mathrm{2}} \left(\mathrm{r}^{\mathrm{2}} \:+\mathrm{4}\right)^{\mathrm{2}\:} \mathrm{dr}\:+\mathrm{8}\:\int_{\mathrm{0}} ^{\mathrm{2}} \:\left(\mathrm{r}^{\mathrm{3}} \:+\mathrm{4r}\right)\mathrm{dr}\int_{−\pi} ^{\pi} \:\mathrm{cos}\theta\:\mathrm{d}\theta\left(\rightarrow\mathrm{0}\right)+\mathrm{16}\int_{\mathrm{0}} ^{\mathrm{2}} \mathrm{r}^{\mathrm{2}} \mathrm{dr}\int_{−\pi} ^{\pi} \:\mathrm{cos}^{\mathrm{2}} \theta\:\mathrm{d}\theta \\ $$$$\int_{\mathrm{0}} ^{\mathrm{2}} \left(\mathrm{r}^{\mathrm{2}} \:+\mathrm{4}\right)^{\mathrm{2}} \:\mathrm{dr}\:=\int_{\mathrm{0}} ^{\mathrm{2}} \left(\mathrm{r}^{\mathrm{4}} \:+\mathrm{8r}^{\mathrm{2}} \:+\mathrm{16}\right)\mathrm{dr}\:=\left[\frac{\mathrm{r}^{\mathrm{5}} }{\mathrm{5}}+\frac{\mathrm{8}}{\mathrm{3}}\mathrm{r}^{\mathrm{3}} \:+\mathrm{16r}\right]_{\mathrm{0}} ^{\mathrm{2}} \:=…. \\ $$$$\int_{\mathrm{0}} ^{\mathrm{2}} \:\mathrm{r}^{\mathrm{2}} \mathrm{dr}\:=\left[\frac{\mathrm{r}^{\mathrm{3}} }{\mathrm{3}}\right]_{\mathrm{0}} ^{\mathrm{2}} \:=\frac{\mathrm{8}}{\mathrm{3}} \\ $$$$\int_{−\pi} ^{\pi} \:\mathrm{cos}^{\mathrm{2}} \theta\:\mathrm{d}\theta\:=\mathrm{2}\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{1}+\mathrm{cos}\left(\mathrm{2}\theta\right)}{\mathrm{2}}\mathrm{d}\theta\:=\pi\:\:\mathrm{rest}\:\mathrm{to}\:\mathrm{collect}\:\mathrm{the}\:\mathrm{values}… \\ $$
