Question Number 168744 by LEKOUMA last updated on 16/Apr/22

$${Resolve} \\ $$$$\left.\mathrm{1}\right)\:\int\frac{\sqrt{\mathrm{1}+\mathrm{cos}\:{x}}}{\mathrm{sin}\:{x}}{dx} \\ $$$$\left.\mathrm{2}\right)\:\int\frac{{dx}}{\mathrm{1}+\sqrt[{\mathrm{3}}]{{x}+\mathrm{1}}} \\ $$$$\left.\mathrm{3}\right)\:\int\frac{{x}\mathrm{tan}\:{x}}{\mathrm{cos}\:^{\mathrm{4}} {x}}{dx} \\ $$$$\left.\mathrm{4}\right)\:\int\frac{{dx}}{\mathrm{1}+\sqrt{{x}}+\sqrt{\mathrm{1}+{x}}} \\ $$
Answered by bobhans last updated on 17/Apr/22
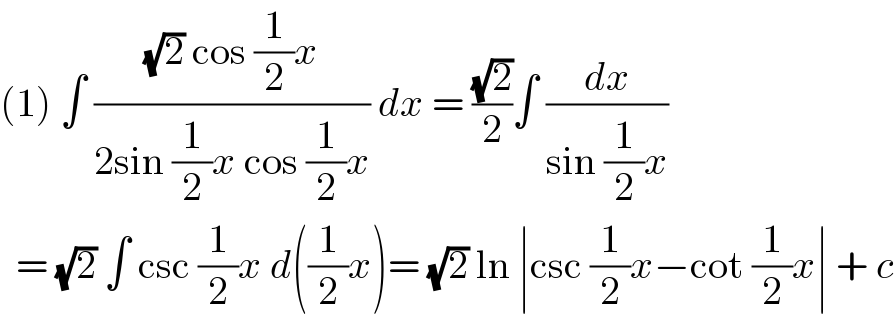
$$\left(\mathrm{1}\right)\:\int\:\frac{\sqrt{\mathrm{2}}\:\mathrm{cos}\:\frac{\mathrm{1}}{\mathrm{2}}{x}}{\mathrm{2sin}\:\frac{\mathrm{1}}{\mathrm{2}}{x}\:\mathrm{cos}\:\frac{\mathrm{1}}{\mathrm{2}}{x}}\:{dx}\:=\:\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\int\:\frac{{dx}}{\mathrm{sin}\:\frac{\mathrm{1}}{\mathrm{2}}{x}}\: \\ $$$$\:\:=\:\sqrt{\mathrm{2}}\:\int\:\mathrm{csc}\:\frac{\mathrm{1}}{\mathrm{2}}{x}\:{d}\left(\frac{\mathrm{1}}{\mathrm{2}}{x}\right)=\:\sqrt{\mathrm{2}}\:\mathrm{ln}\:\mid\mathrm{csc}\:\frac{\mathrm{1}}{\mathrm{2}}{x}−\mathrm{cot}\:\frac{\mathrm{1}}{\mathrm{2}}{x}\mid\:+\:{c} \\ $$
Answered by bobhans last updated on 17/Apr/22
![(2) ∫ (dx/(1+((x+1))^(1/3) )) ; [ x=t^3 −1 ] = ∫ ((3t^2 dt)/(1+t)) = 3∫ (((t+1)^2 −2t−1)/(1+t)) dt = 3[ ∫ (t+1)dt−∫ ((2(t+1)−1)/(1+t)) dt ] = 3 [(((t+1)^2 )/2)−2t+ln ∣1+t∣ ] +c = 3[ ((1+((x+1))^(1/3) )/2) −2((x+1))^(1/3) +ln ∣1+((x+1))^(1/3) ∣ ]+c](https://www.tinkutara.com/question/Q168770.png)
$$\left(\mathrm{2}\right)\:\int\:\frac{{dx}}{\mathrm{1}+\sqrt[{\mathrm{3}}]{{x}+\mathrm{1}}}\:;\:\left[\:{x}={t}^{\mathrm{3}} −\mathrm{1}\:\right] \\ $$$$\:=\:\int\:\frac{\mathrm{3}{t}^{\mathrm{2}} \:{dt}}{\mathrm{1}+{t}}\:=\:\mathrm{3}\int\:\frac{\left({t}+\mathrm{1}\right)^{\mathrm{2}} −\mathrm{2}{t}−\mathrm{1}}{\mathrm{1}+{t}}\:{dt} \\ $$$$\:=\:\mathrm{3}\left[\:\int\:\left({t}+\mathrm{1}\right){dt}−\int\:\frac{\mathrm{2}\left({t}+\mathrm{1}\right)−\mathrm{1}}{\mathrm{1}+{t}}\:{dt}\:\right] \\ $$$$\:=\:\mathrm{3}\:\left[\frac{\left({t}+\mathrm{1}\right)^{\mathrm{2}} }{\mathrm{2}}−\mathrm{2}{t}+\mathrm{ln}\:\mid\mathrm{1}+{t}\mid\:\right]\:+{c}\: \\ $$$$\:=\:\mathrm{3}\left[\:\frac{\mathrm{1}+\sqrt[{\mathrm{3}}]{{x}+\mathrm{1}}}{\mathrm{2}}\:−\mathrm{2}\sqrt[{\mathrm{3}}]{{x}+\mathrm{1}}\:+\mathrm{ln}\:\mid\mathrm{1}+\sqrt[{\mathrm{3}}]{{x}+\mathrm{1}}\mid\:\right]+{c}\: \\ $$
Answered by MJS_new last updated on 17/Apr/22
![∫(dx/(1+(√x)+(√(x+1))))= [t=(√x)+(√(x+1)) → dx=((2(√x)(√(x+1)))/t)dt; x=(((t^2 −1)^2 )/(4t^2 ))] =(1/2)∫(((t−1)(t^2 +1))/t^3 )dt= =(1/2)∫(1−(1/t)+(1/t^2 )−(1/t^3 ))dt= =(t/2)−(1/2)ln t −(1/(2t))+(1/(4t^2 ))= =((x+2(√x)−(√x)(√(x+1))−ln ((√x)+(√(x+1))))/2)+C](https://www.tinkutara.com/question/Q168802.png)
$$\int\frac{{dx}}{\mathrm{1}+\sqrt{{x}}+\sqrt{{x}+\mathrm{1}}}= \\ $$$$\:\:\:\:\:\left[{t}=\sqrt{{x}}+\sqrt{{x}+\mathrm{1}}\:\rightarrow\:{dx}=\frac{\mathrm{2}\sqrt{{x}}\sqrt{{x}+\mathrm{1}}}{{t}}{dt};\:{x}=\frac{\left({t}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} }{\mathrm{4}{t}^{\mathrm{2}} }\right] \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\left({t}−\mathrm{1}\right)\left({t}^{\mathrm{2}} +\mathrm{1}\right)}{{t}^{\mathrm{3}} }{dt}= \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\left(\mathrm{1}−\frac{\mathrm{1}}{{t}}+\frac{\mathrm{1}}{{t}^{\mathrm{2}} }−\frac{\mathrm{1}}{{t}^{\mathrm{3}} }\right){dt}= \\ $$$$=\frac{{t}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:{t}\:−\frac{\mathrm{1}}{\mathrm{2}{t}}+\frac{\mathrm{1}}{\mathrm{4}{t}^{\mathrm{2}} }= \\ $$$$=\frac{{x}+\mathrm{2}\sqrt{{x}}−\sqrt{{x}}\sqrt{{x}+\mathrm{1}}−\mathrm{ln}\:\left(\sqrt{{x}}+\sqrt{{x}+\mathrm{1}}\right)}{\mathrm{2}}+{C} \\ $$
