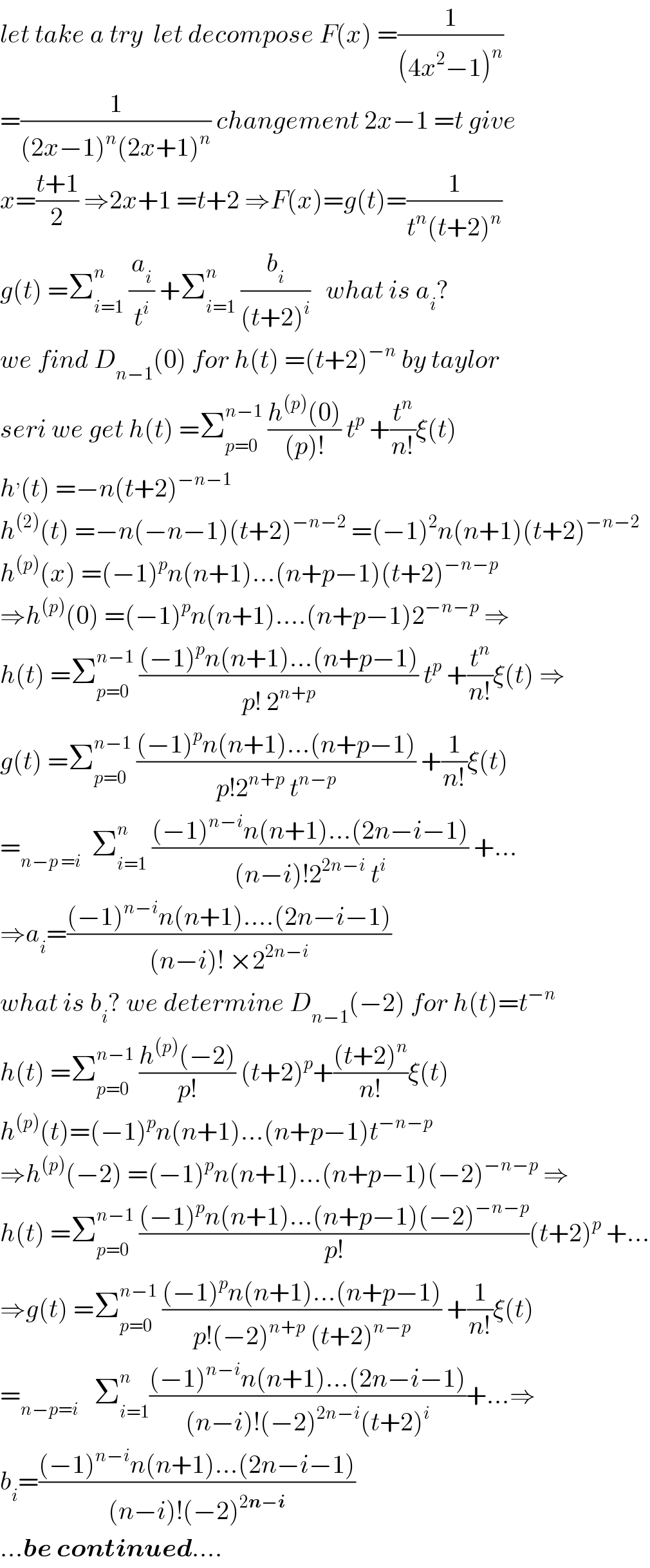S-n-k-1-1-4k-2-1-n-find-a-simpler-form-

Question Number 92925 by frc2crc last updated on 09/May/20

S_{n} = \underset{k = 1}{\sum^{\infty}} \frac{1}{{(4 k^{2} - 1)}^{n}}

f i n d a s i m p l e r f o r m

Commented by mr W last updated on 09/May/20

c h e c k y o u r q u e s t i o n p l e a s e .

\underset{k = 1}{\sum^{n o r \infty}}

Commented by frc2crc last updated on 09/May/20

\underset{k = 1}{\sum^{\infty}} \frac{1}{{(4 k^{2} - 1)}^{n}} t h i s s u m f o r a n y n > 0

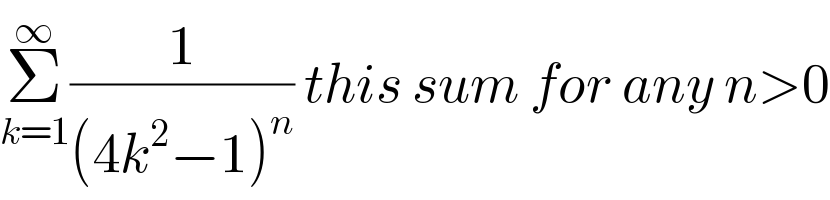
Commented by prakash jain last updated on 09/May/20

series is convergent for n ⩾ 1 . We

can use limit comparion test .

n = 1

\frac{1}{4 k^{2} - 1} = \frac{1}{2} (\frac{1}{2 k - 1} - \frac{1}{2 k + 1})

\Rightarrow \underset{k = 1}{\sum^{\infty}} \frac{1}{4 k^{2} - 1} = \frac{1}{2}

n = 2 can be evaluated using

known series expansion for π^{2} .

- - - - -

I will check if a generic closed

form formula is possible .

It may exists using zeta function .

Commented by abdomathmax last updated on 10/May/20

l e t t a k e a t r y l e t d e c o m p o s e F (x) = \frac{1}{{(4 x^{2} - 1)}^{n}}

= \frac{1}{{(2 x - 1)}^{n} {(2 x + 1)}^{n}} c h a n g e m e n t 2 x - 1 = t g i v e

x = \frac{t + 1}{2} \Rightarrow 2 x + 1 = t + 2 \Rightarrow F (x) = g (t) = \frac{1}{t^{n} {(t + 2)}^{n}}

g (t) = \sum_{i = 1}^{n} \frac{a_{i}}{t^{i}} + \sum_{i = 1}^{n} \frac{b_{i}}{{(t + 2)}^{i}} w h a t i s a_{i} ?

w e f i n d D_{n - 1} (0) f o r h (t) = {(t + 2)}^{- n} b y t a y l o r

s e r i w e g e t h (t) = \sum_{p = 0}^{n - 1} \frac{h^{(p)} (0)}{(p)!} t^{p} + \frac{t^{n}}{n!} ξ (t)

h^{,} (t) = - n {(t + 2)}^{- n - 1}

h^{(2)} (t) = - n (- n - 1) {(t + 2)}^{- n - 2} = {(- 1)}^{2} n (n + 1) {(t + 2)}^{- n - 2}

h^{(p)} (x) = {(- 1)}^{p} n (n + 1) \dots (n + p - 1) {(t + 2)}^{- n - p}

\Rightarrow h^{(p)} (0) = {(- 1)}^{p} n (n + 1) \dots . (n + p - 1) 2^{- n - p} \Rightarrow

h (t) = \sum_{p = 0}^{n - 1} \frac{{(- 1)}^{p} n (n + 1) \dots (n + p - 1)}{p! 2^{n + p}} t^{p} + \frac{t^{n}}{n!} ξ (t) \Rightarrow

g (t) = \sum_{p = 0}^{n - 1} \frac{{(- 1)}^{p} n (n + 1) \dots (n + p - 1)}{p! 2^{n + p} t^{n - p}} + \frac{1}{n!} ξ (t)

=_{n - p = i} \sum_{i = 1}^{n} \frac{{(- 1)}^{n - i} n (n + 1) \dots (2 n - i - 1)}{(n - i)! 2^{2 n - i} t^{i}} + \dots

\Rightarrow a_{i} = \frac{{(- 1)}^{n - i} n (n + 1) \dots . (2 n - i - 1)}{(n - i)! \times 2^{2 n - i}}

w h a t i s b_{i} ? w e d e t e r m i n e D_{n - 1} (- 2) f o r h (t) = t^{- n}

h (t) = \sum_{p = 0}^{n - 1} \frac{h^{(p)} (- 2)}{p!} {(t + 2)}^{p} + \frac{{(t + 2)}^{n}}{n!} ξ (t)

h^{(p)} (t) = {(- 1)}^{p} n (n + 1) \dots (n + p - 1) t^{- n - p}

\Rightarrow h^{(p)} (- 2) = {(- 1)}^{p} n (n + 1) \dots (n + p - 1) {(- 2)}^{- n - p} \Rightarrow

h (t) = \sum_{p = 0}^{n - 1} \frac{{(- 1)}^{p} n (n + 1) \dots (n + p - 1) {(- 2)}^{- n - p}}{p!} {(t + 2)}^{p} + \dots

\Rightarrow g (t) = \sum_{p = 0}^{n - 1} \frac{{(- 1)}^{p} n (n + 1) \dots (n + p - 1)}{p! {(- 2)}^{n + p} {(t + 2)}^{n - p}} + \frac{1}{n!} ξ (t)

=_{n - p = i} \sum_{i = 1}^{n} \frac{{(- 1)}^{n - i} n (n + 1) \dots (2 n - i - 1)}{(n - i)! {(- 2)}^{2 n - i} {(t + 2)}^{i}} + \dots \Rightarrow

b_{i} = \frac{{(- 1)}^{n - i} n (n + 1) \dots (2 n - i - 1)}{(n - i)! {(- 2)}^{2 n - i}}

\dots b e c o n t i n u e d \dots .