S-x-2x-2-nx-n-

Question Number 153847 by mathdanisur last updated on 11/Sep/21

S = x + {2 x}^{2} + \dots + {nx}^{n}

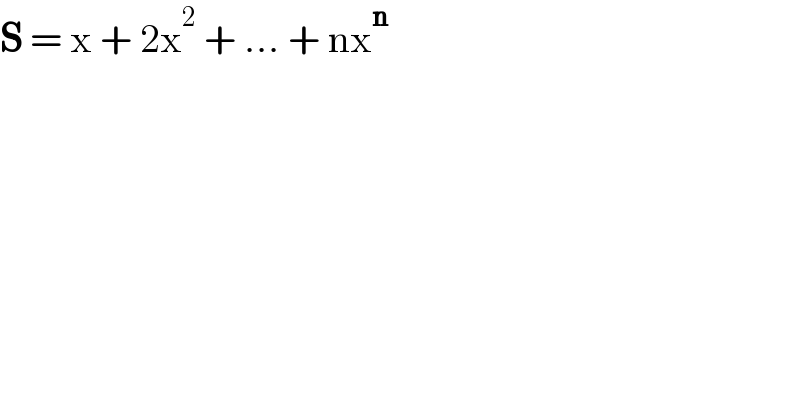
Answered by liberty last updated on 11/Sep/21

S = x + 2 x^{2} + 3 x^{3} + 4 x^{4} + \dots + {n x}^{n}

x S = x^{2} + 2 x^{3} + 3 x^{4} + \dots + (n - 1) x^{n} + {n x}^{n + 1}

(1 - x) S = x + x^{2} + x^{3} + x^{4} + \dots + x^{n} - {n x}^{n + 1}

(1 - x) S = \frac{x (1 - x^{n})}{1 - x} - {n x}^{n + 1}

S = \frac{x (1 - x^{n})}{{(1 - x)}^{2}} - \frac{{n x}^{n + 1}}{(1 - x)}

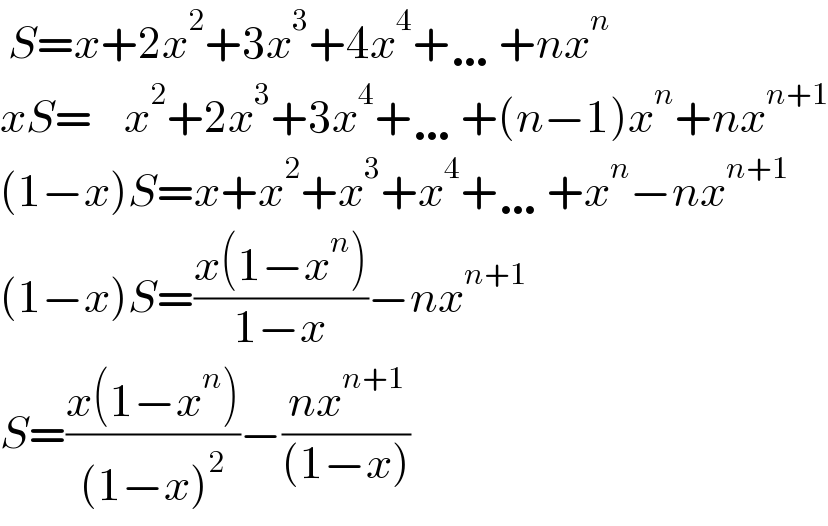
Commented by mathdanisur last updated on 11/Sep/21

thanks ser nice

Commented by peter frank last updated on 11/Sep/21

thanks

Answered by mr W last updated on 11/Sep/21

M e t h o d 1 :

x + x^{2} + \dots + x^{n} = \frac{x^{n} - 1}{x - 1}

1 + 2 x + \dots + {n x}^{n - 1} = \frac{{n x}^{n - 1}}{x - 1} - \frac{x^{n} - 1}{{(x - 1)}^{2}}

x + 2 x^{2} + \dots + {n x}^{n} = \frac{{n x}^{n}}{x - 1} - \frac{(x^{n} - 1) x}{{(x - 1)}^{2}}

M e t h o d 2 :

S = x + 2 x^{2} + 3 x^{3} + \dots + {n x}^{n}

x S = x^{2} + 2 x^{3} + \dots + (n - 1) x^{n} + {n x}^{n + 1}

(1 - x) S = x + x^{2} + x^{3} + \dots x^{n} - {n x}^{n + 1}

(1 - x) S = \frac{(x^{n} - 1) x}{x - 1} - {n x}^{n + 1}

\Rightarrow S = \frac{{n x}^{n + 1}}{x - 1} - \frac{(x^{n} - 1) x}{{(x - 1)}^{2}}

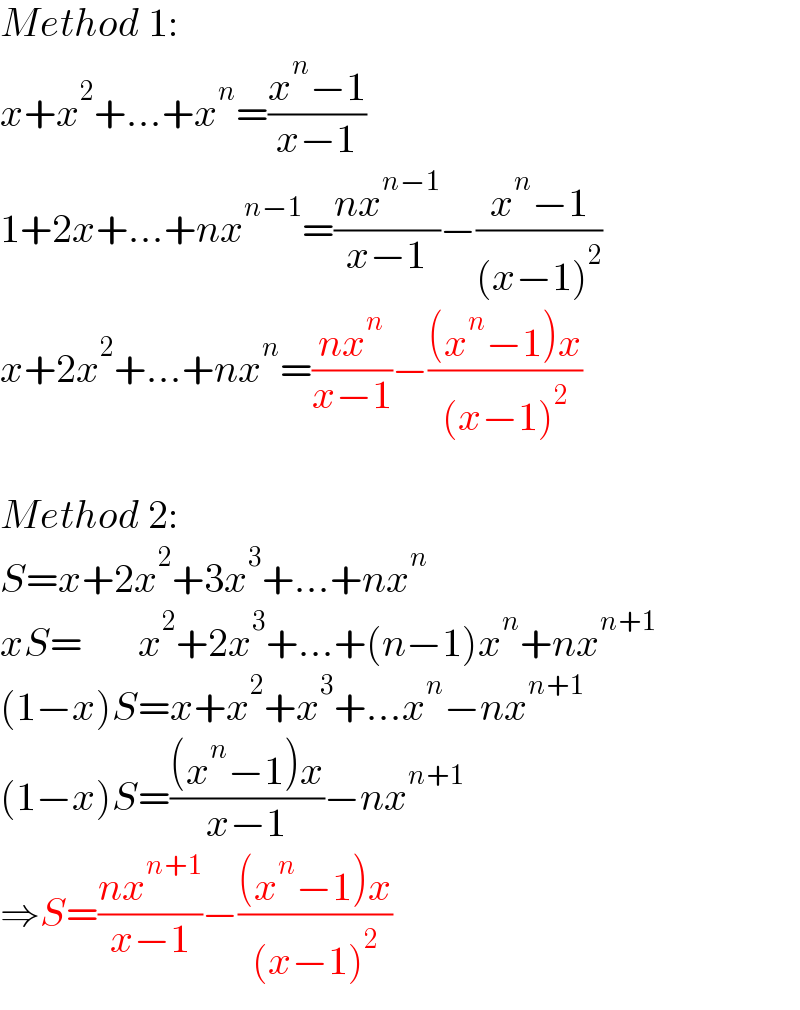
Commented by mathdanisur last updated on 11/Sep/21

Very nice thanks ser