sec-x-tan-x-dx-how-can-it-solve-

Question Number 151568 by tabata last updated on 21/Aug/21

\int \sqrt{s e c (x) + t a n (x)} d x

h o w c a n i t s o l v e

Answered by puissant last updated on 22/Aug/21

= \int \sqrt{\frac{1}{c o s x} + \frac{s i n x}{c o s x}} d x

t = t a n \frac{x}{2} \to d x = \frac{2}{1 + t^{2}} d t

K = \int \sqrt{\frac{1 + t^{2}}{1 - t^{2}} + \frac{2 t}{1 - t^{2}}} \times \frac{2}{1 + t^{2}} d t

= \int \sqrt{\frac{{(1 + t)}^{2}}{(1 - t) (1 + t)}} \times \frac{2}{1 + t^{2}} d t

= 2 \int \sqrt{\frac{1 + t}{1 - t}} \times \frac{1}{1 + t^{2}} d t

u^{2} = \frac{1 + t}{1 - t} \Rightarrow u^{2} - u^{2} t = 1 + t \Rightarrow t (1 + u^{2}) = u^{2} - 1

\Rightarrow t = \frac{u^{2} - 1}{u^{2} + 1} \to d t = \frac{2 u (u^{2} + 1) - 2 u (u^{2} - 1)}{{(u^{2} + 1)}^{2}} d u

\to d t = \frac{4 u}{{(u^{2} + 1)}^{2}} d u

K = 2 \int \frac{4 u^{2}}{{(u^{2} + 1)}^{2}} \times \frac{1}{1 + {(\frac{u^{2} - 1}{u^{2} + 1})}^{2}} d u

K = 2 \int \frac{4 u^{2}}{{(u^{2} + 1)}^{2}} \times \frac{{(u^{2} + 1)}^{2}}{{(u^{2} + 1)}^{2} + {(u^{2} - 1)}^{2}} d u

K = 8 \int \frac{u^{2}}{u^{4} + 2 u^{2} + 1 + u^{4} - 2 u^{2} + 1} d u

K = 8 \int \frac{u^{2}}{2 (u^{4} + 1)} d u

K = 4 \int \frac{u^{2}}{u^{4} + 1} d u

t o b e c o n t i n u e d \dots

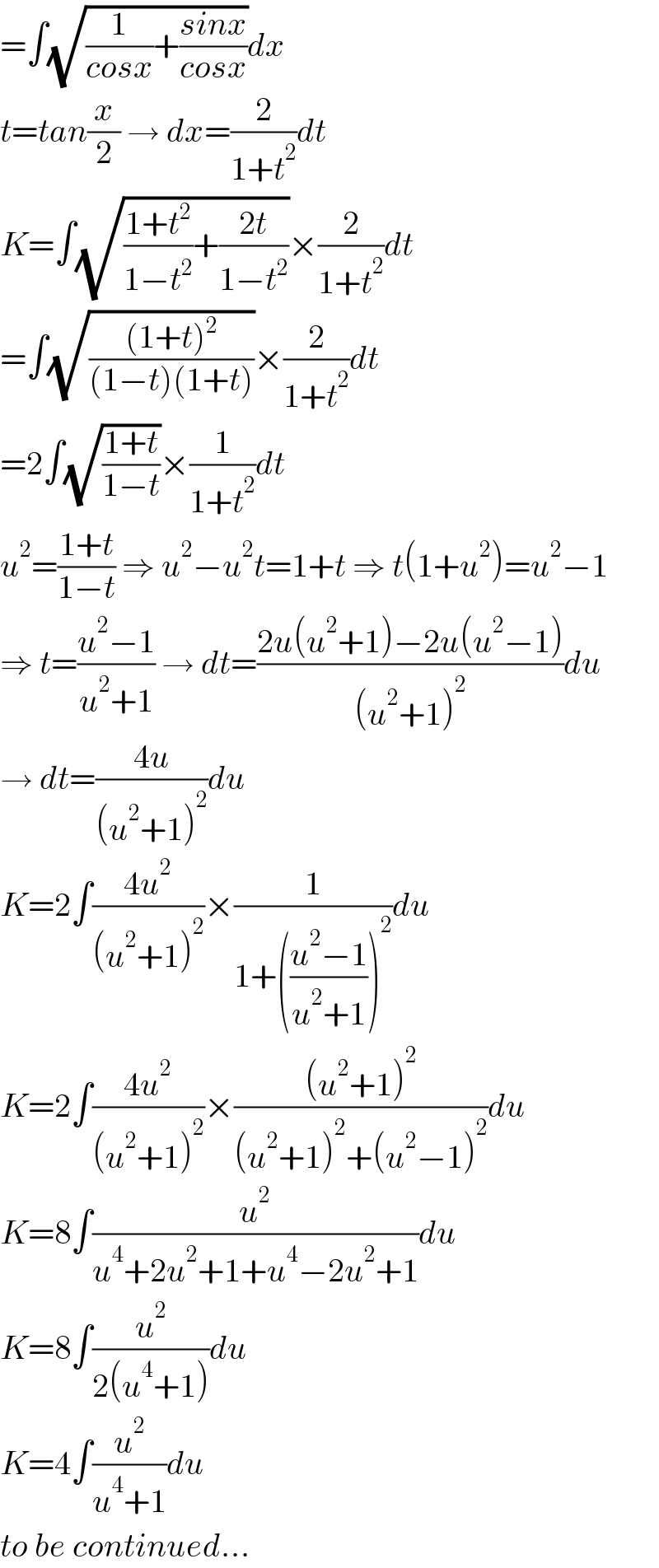
Commented by peter frank last updated on 22/Aug/21

4 \int . \frac{1}{2} . \frac{{2 u}^{2}}{1 + u^{4}} = 2 \int \frac{{2 u}^{2}}{1 + u^{4}}

2 \int \frac{{2 u}^{2}}{1 + u^{4}} = 4 \int \frac{(u^{2} + 1) + (u^{2} - 1)}{1 + u^{4}} du

4 [\int \frac{1 + u^{2}}{1 + u^{4}} du + \int \frac{1 - u^{2}}{1 + u^{4}} du]

4 [\int \frac{1 + \frac{1}{u^{2}}}{{(u - \frac{1}{u})}^{2} + 2} + \int \frac{1 - \frac{1}{u^{2}}}{{(u + \frac{1}{u})}^{2} + 2}

t = u - \frac{1}{u}

dt = (1 + \frac{1}{u^{2}}) du

x = u + \frac{1}{u}

dx = (1 - \frac{1}{u^{2}}) du

4 [\int \frac{1}{2} . \frac{dt}{t^{2} + 2} + \int \frac{1}{2} . \int \frac{dx}{x^{2} - 2}

2 [\frac{1}{\sqrt{2}} \tan^{- 1} (\frac{t}{\sqrt{2}}) + \frac{1}{2 \sqrt{2}} \ln (\frac{x - \sqrt{2}}{x + \sqrt{2}})

Answered by peter frank last updated on 22/Aug/21

\int \sqrt{\frac{1}{\cos x} + \frac{\sin x}{\cos x}} dx

\int \sqrt{\frac{1 + \sin x}{\cos x}} dx

\int \sqrt{\frac{{(\sin \frac{x}{2} + \cos \frac{x}{2})}^{2}}{\cos^{2} \frac{x}{2} - \sin^{2} \frac{x}{2}}} dx

\int \sqrt{\frac{{(\sin \frac{x}{2} + \cos \frac{x}{2})}^{2}}{(\cos \frac{x}{2} + \sin \frac{x}{2}) (\cos \frac{x}{2} - \sin \frac{x}{2})}} dx

\int \sqrt{\frac{(\sin \frac{x}{2} + \cos \frac{x}{2})}{(\cos \frac{x}{2} - \sin \frac{x}{2})}} dx

\int \sec^{2} \frac{x}{2} . \sqrt{\frac{(\tan \frac{x}{2} + 1)}{(1 - \tan \frac{x}{2})}} dx

u = \tan \frac{x}{2}

du = \frac{1}{2} \sec^{2} \frac{x}{2} dx

\int \sqrt{\frac{u + 1}{1 - u}} du . 2

\int 2 \sqrt{\frac{u + 1}{1 - u} . \frac{u + 1}{u - 1}} du .

\int 2 . \frac{u + 1}{\sqrt{1 - u^{2}}} du .

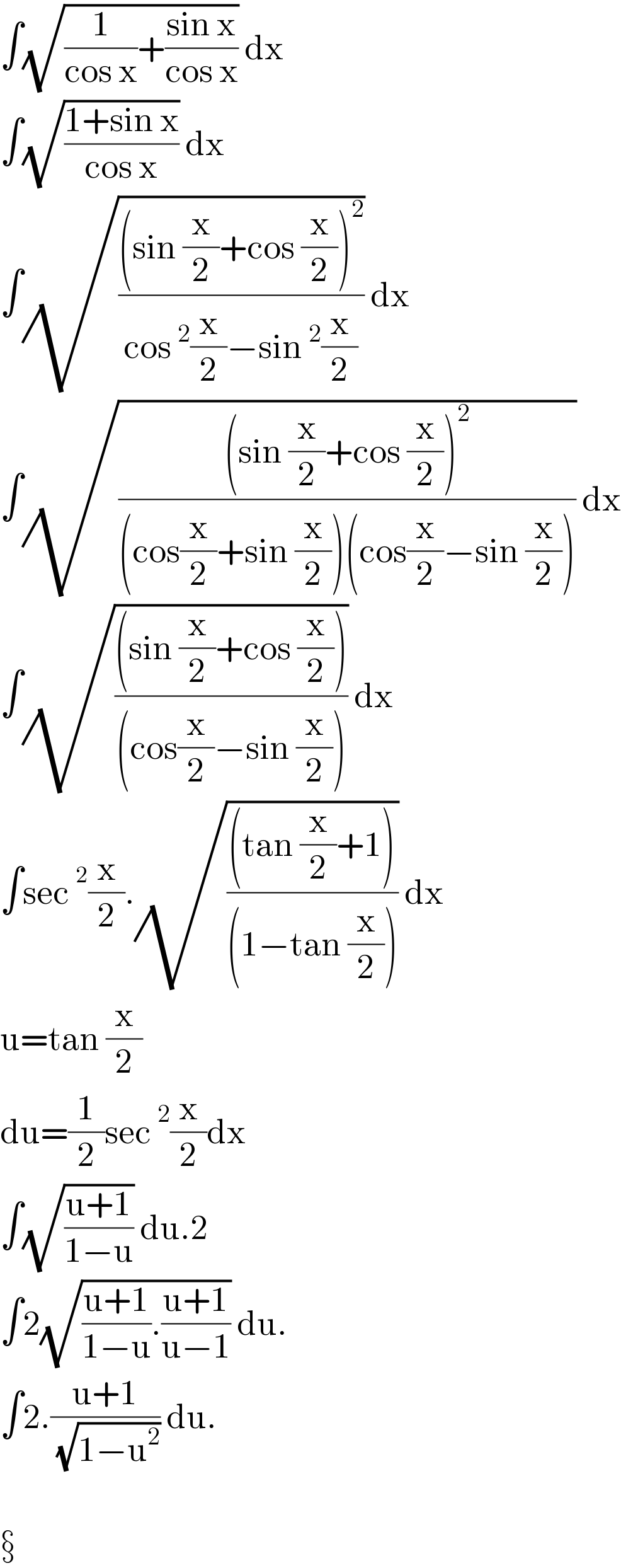
Commented by talminator2856791 last updated on 22/Aug/21

the font is so big! hahahahahah

Answered by MJS_new last updated on 22/Aug/21

\int \sqrt{\sec x + \tan x} d x =

[t = \tan \frac{x}{2} \to d x = \frac{2 d t}{1 + t^{2}}]

= 2 \int \frac{\sqrt{1 + t}}{(1 + t^{2}) \sqrt{1 - t}} d t =

[u = \frac{\sqrt{1 - t}}{\sqrt{1 + t}} \to d t = - \sqrt{(1 - t) {(1 + t)}^{3}} d u]

= - 4 \int \frac{d u}{u^{4} + 1} =

= \frac{1}{\sqrt{2}} \int \frac{2 u - \sqrt{2}}{u^{2} - \sqrt{2} u + 1} d u - \int \frac{d u}{u^{2} - \sqrt{2} u + 1} -

- \frac{1}{\sqrt{2}} \int \frac{2 u + \sqrt{2}}{u^{2} + \sqrt{2} u + 1} d u - \int \frac{d u}{u^{2} + \sqrt{2} u + 1} =

= \frac{\sqrt{2}}{2} \ln (u^{2} - \sqrt{2} u + 1) - \sqrt{2} \arctan (\sqrt{2} u - 1) -

+ \frac{\sqrt{2}}{2} \ln (u^{2} + \sqrt{2} u + 1) - \sqrt{2} \arctan (\sqrt{2} u + 1)

\dots

Commented by peter frank last updated on 22/Aug/21

thank you

Answered by Ar Brandon last updated on 22/Aug/21

I = \int \sqrt{\sec x + \tan x} d x = \int \sqrt{\frac{1 + \sin x}{\cos x}} d x

= \int \sqrt{\frac{{(\cos \frac{x}{2} + \sin \frac{x}{2})}^{2}}{\cos x}} d x = \int \frac{\cos \frac{x}{2} + \sin \frac{x}{2}}{\sqrt{\cos x}} d x

= \int \frac{\cos \frac{x}{2}}{\sqrt{1 - {2 \sin}^{2} \frac{x}{2}}} d x + \int \frac{\sin \frac{x}{2}}{\sqrt{{2 \cos}^{2} \frac{x}{2} - 1}} d x

= \sqrt{2} \arcsin (\sqrt{2} \sin \frac{x}{2}) - \sqrt{2} argcosh (\sqrt{2} \cos \frac{x}{2}) + C

= \sqrt{2} \arcsin (\sqrt{2} \sin \frac{x}{2}) - \sqrt{2} \ln ∣ \sqrt{2} \cos \frac{x}{2} + \sqrt{{2 \cos}^{2} \frac{x}{2} - 1} ∣ + C

Commented by peter frank last updated on 22/Aug/21

thank you