Question Number 111620 by mathdave last updated on 04/Sep/20
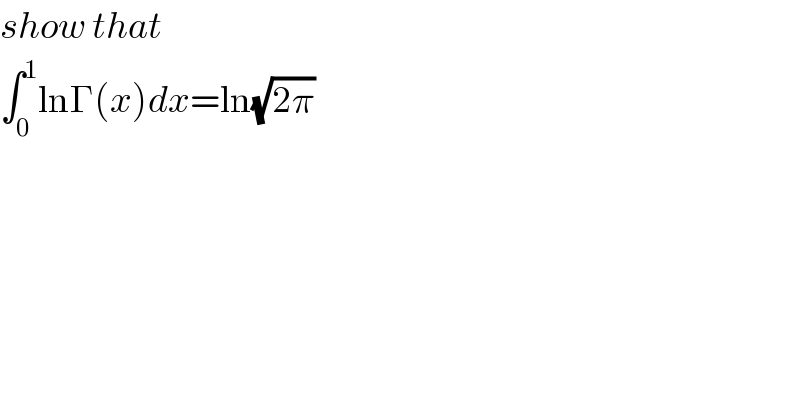
$${show}\:{that} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\Gamma\left({x}\right){dx}=\mathrm{ln}\sqrt{\mathrm{2}\pi} \\ $$
Answered by mathmax by abdo last updated on 04/Sep/20
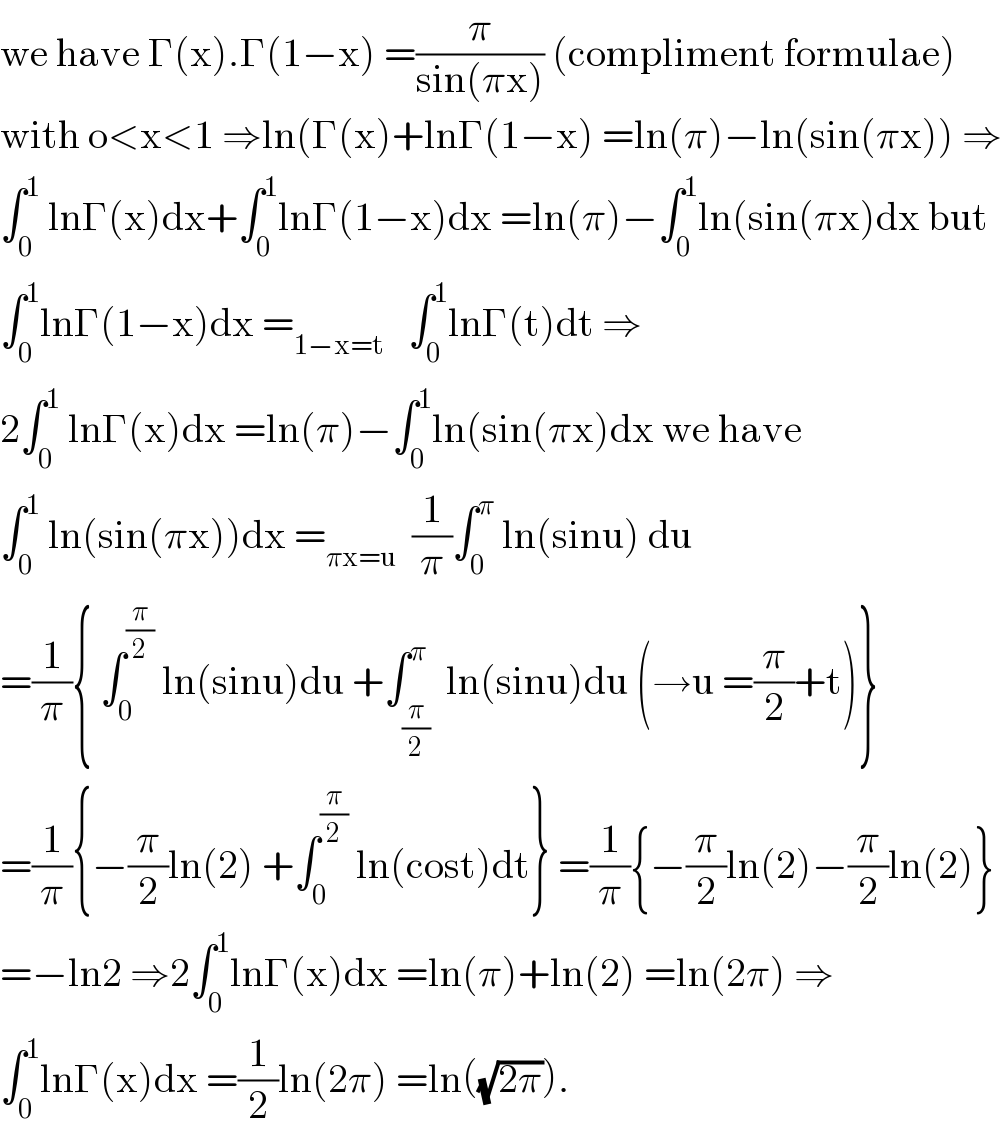
$$\mathrm{we}\:\mathrm{have}\:\Gamma\left(\mathrm{x}\right).\Gamma\left(\mathrm{1}−\mathrm{x}\right)\:=\frac{\pi}{\mathrm{sin}\left(\pi\mathrm{x}\right)}\:\left(\mathrm{compliment}\:\mathrm{formulae}\right) \\ $$$$\mathrm{with}\:\mathrm{o}<\mathrm{x}<\mathrm{1}\:\Rightarrow\mathrm{ln}\left(\Gamma\left(\mathrm{x}\right)+\mathrm{ln}\Gamma\left(\mathrm{1}−\mathrm{x}\right)\:=\mathrm{ln}\left(\pi\right)−\mathrm{ln}\left(\mathrm{sin}\left(\pi\mathrm{x}\right)\right)\:\Rightarrow\right. \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{ln}\Gamma\left(\mathrm{x}\right)\mathrm{dx}+\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\Gamma\left(\mathrm{1}−\mathrm{x}\right)\mathrm{dx}\:=\mathrm{ln}\left(\pi\right)−\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\left(\mathrm{sin}\left(\pi\mathrm{x}\right)\mathrm{dx}\:\mathrm{but}\right. \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\Gamma\left(\mathrm{1}−\mathrm{x}\right)\mathrm{dx}\:=_{\mathrm{1}−\mathrm{x}=\mathrm{t}} \:\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\Gamma\left(\mathrm{t}\right)\mathrm{dt}\:\Rightarrow \\ $$$$\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{ln}\Gamma\left(\mathrm{x}\right)\mathrm{dx}\:=\mathrm{ln}\left(\pi\right)−\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\left(\mathrm{sin}\left(\pi\mathrm{x}\right)\mathrm{dx}\:\mathrm{we}\:\mathrm{have}\right. \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{ln}\left(\mathrm{sin}\left(\pi\mathrm{x}\right)\right)\mathrm{dx}\:=_{\pi\mathrm{x}=\mathrm{u}} \:\:\frac{\mathrm{1}}{\pi}\int_{\mathrm{0}} ^{\pi} \:\mathrm{ln}\left(\mathrm{sinu}\right)\:\mathrm{du} \\ $$$$=\frac{\mathrm{1}}{\pi}\left\{\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{sinu}\right)\mathrm{du}\:+\int_{\frac{\pi}{\mathrm{2}}} ^{\pi} \:\mathrm{ln}\left(\mathrm{sinu}\right)\mathrm{du}\:\left(\rightarrow\mathrm{u}\:=\frac{\pi}{\mathrm{2}}+\mathrm{t}\right)\right\} \\ $$$$=\frac{\mathrm{1}}{\pi}\left\{−\frac{\pi}{\mathrm{2}}\mathrm{ln}\left(\mathrm{2}\right)\:+\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{cost}\right)\mathrm{dt}\right\}\:=\frac{\mathrm{1}}{\pi}\left\{−\frac{\pi}{\mathrm{2}}\mathrm{ln}\left(\mathrm{2}\right)−\frac{\pi}{\mathrm{2}}\mathrm{ln}\left(\mathrm{2}\right)\right\} \\ $$$$=−\mathrm{ln2}\:\Rightarrow\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\Gamma\left(\mathrm{x}\right)\mathrm{dx}\:=\mathrm{ln}\left(\pi\right)+\mathrm{ln}\left(\mathrm{2}\right)\:=\mathrm{ln}\left(\mathrm{2}\pi\right)\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\Gamma\left(\mathrm{x}\right)\mathrm{dx}\:=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\left(\mathrm{2}\pi\right)\:=\mathrm{ln}\left(\sqrt{\mathrm{2}\pi}\right). \\ $$
Commented by mnjuly1970 last updated on 06/Sep/20
$${thank}\:{you}\:{so}\:{much}\:'\:{sir}'\:{for} \\ $$$${your}\:\:{effort}\:… \\ $$
Commented by Tawa11 last updated on 06/Sep/21

$$\mathrm{great}\:\mathrm{sir} \\ $$
