Question Number 49604 by Tawa1 last updated on 08/Dec/18
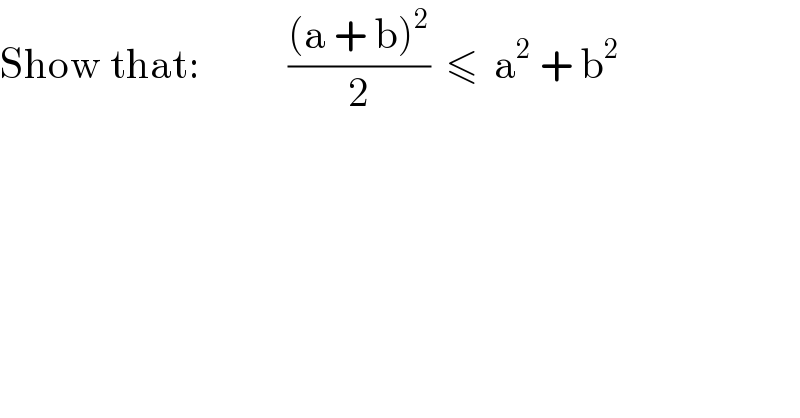
Answered by afachri last updated on 08/Dec/18
![let (a − b)^2 ≥ 0 a^2 − 2ab + b^2 ≥ 0 a^2 + b^2 ≥ 2ab a^2 + 2ab + b^2 ≥ 2ab + 2ab (a + b)^2 ≥ 4(ab) meanwhile : ab = (( (a + b)^2 − (a^2 + b^2 ) )/2) (a + b)^2 ≥ 4((( (a + b)^2 − (a^2 + b^2 ))/2)) (a + b)^(2 ) ≥ 2(a + b)^2 − 2(a^2 + b^2 )] −(a + b)^2 ≥ −2(a^2 + b^2 ) (((a + b)^2 )/2) ≤ (a^2 + b^2 )](https://www.tinkutara.com/question/Q49619.png)
Commented by Tawa1 last updated on 08/Dec/18
Commented by Tawa1 last updated on 08/Dec/18
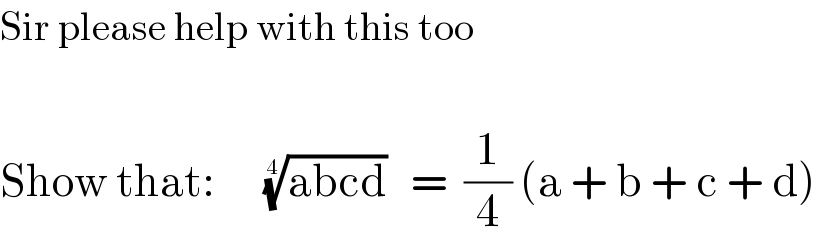
Commented by afachri last updated on 08/Dec/18
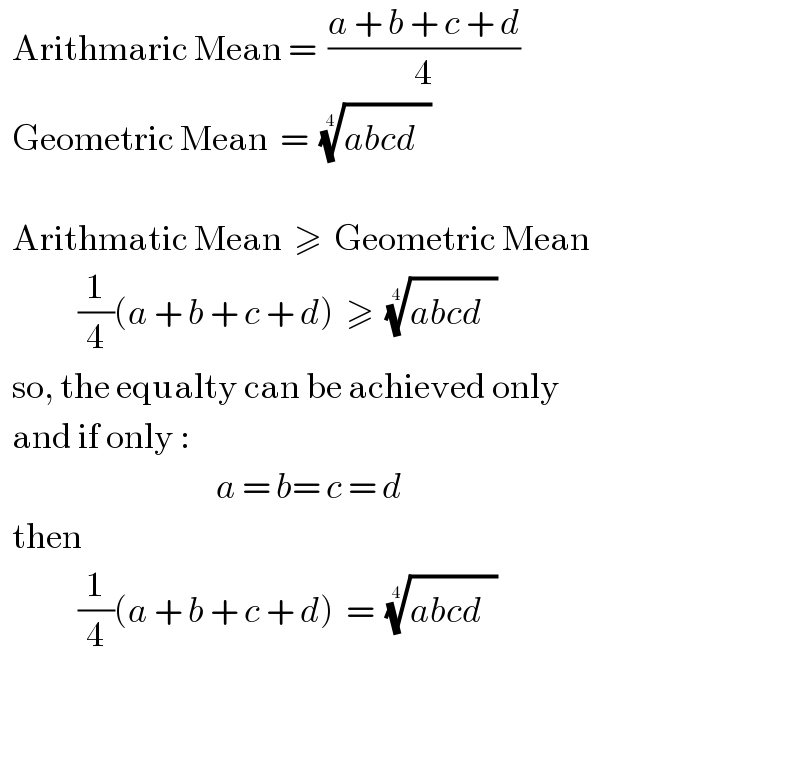
Commented by Tawa1 last updated on 08/Dec/18
Commented by afachri last updated on 08/Dec/18

Commented by Tawa1 last updated on 08/Dec/18

Commented by afachri last updated on 08/Dec/18
Commented by Tawa1 last updated on 08/Dec/18

Commented by Tawa1 last updated on 08/Dec/18
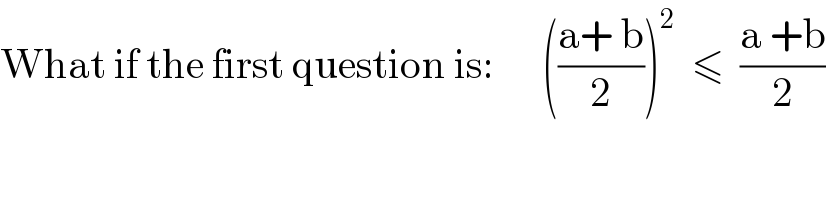
Commented by Tawa1 last updated on 08/Dec/18
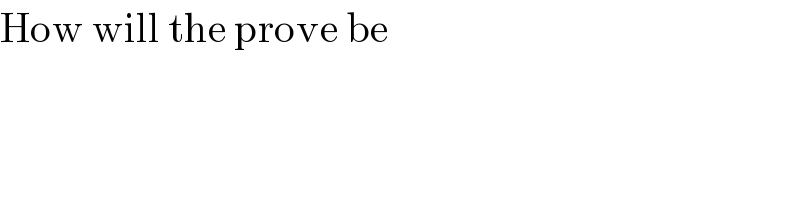
Commented by afachri last updated on 08/Dec/18

Answered by afachri last updated on 08/Dec/18
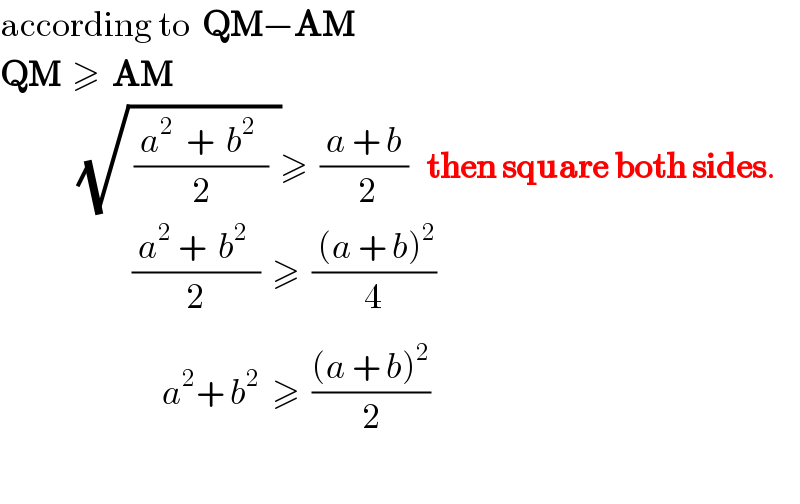
Commented by Tawa1 last updated on 08/Dec/18
Commented by Tawa1 last updated on 08/Dec/18
Commented by afachri last updated on 08/Dec/18

Commented by Tawa1 last updated on 08/Dec/18

Commented by afachri last updated on 08/Dec/18
Commented by Tawa1 last updated on 08/Dec/18

Commented by afachri last updated on 08/Dec/18