Menu Close

show-that-cos-4pi-5-cos-2pi-5-1-0-

Tinku Tara 0 Comments
Pin




Question Number 125686 by mathocean1 last updated on 12/Dec/20
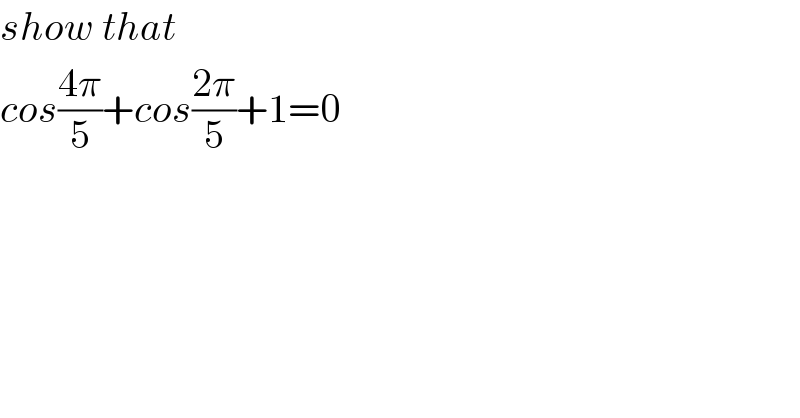
show that cos((4π)/5)+cos((2π)/5)+1=0
$${show}\:{that} \\ $$$${cos}\frac{\mathrm{4}\pi}{\mathrm{5}}+{cos}\frac{\mathrm{2}\pi}{\mathrm{5}}+\mathrm{1}=\mathrm{0} \\ $$
Commented by bramlexs22 last updated on 13/Dec/20
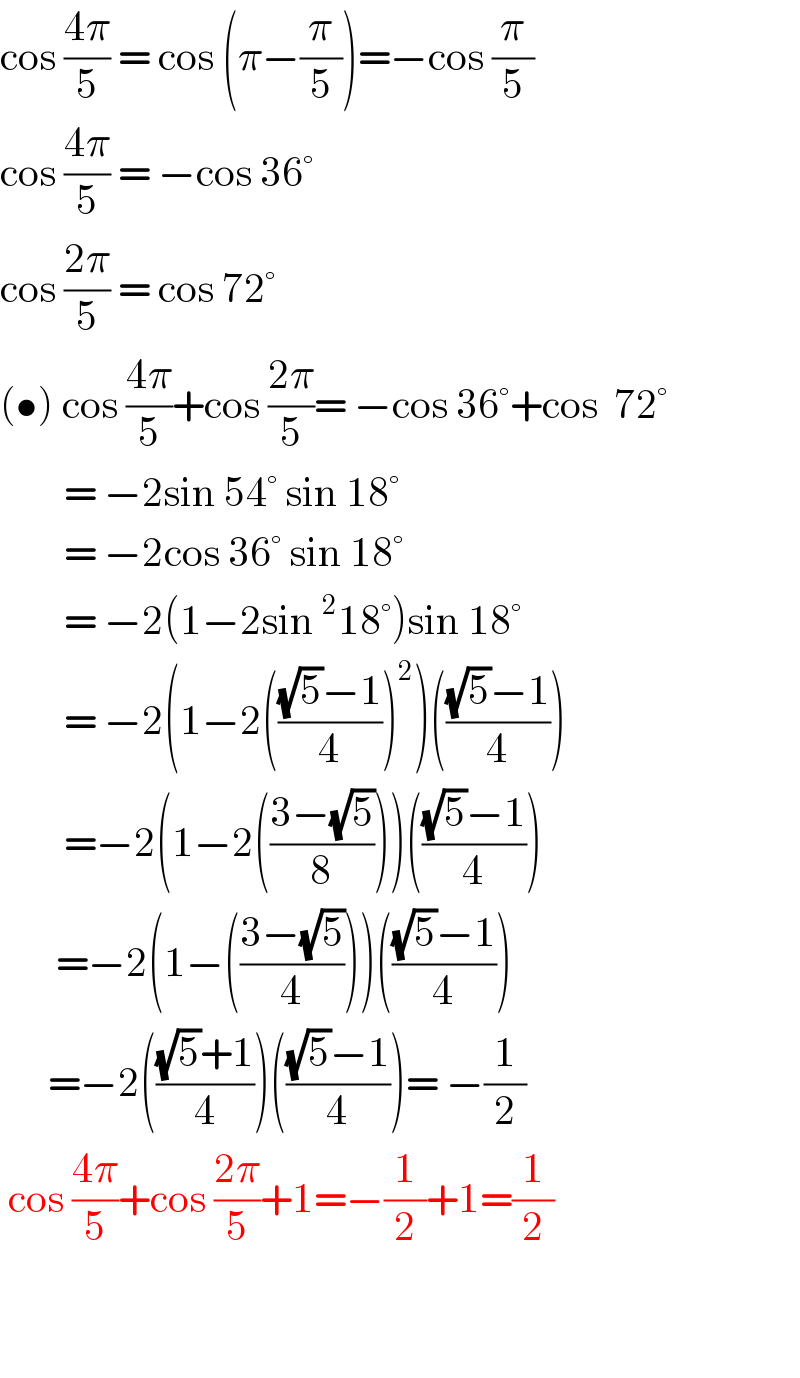
cos ((4π)/5) = cos (π−(π/5))=−cos (π/5) cos ((4π)/5) = −cos 36° cos ((2π)/5) = cos 72° (•) cos ((4π)/5)+cos ((2π)/5)= −cos 36°+cos 72° = −2sin 54° sin 18° = −2cos 36° sin 18° = −2(1−2sin^2 18°)sin 18° = −2(1−2((((√5)−1)/4))^2 )((((√5)−1)/4)) =−2(1−2(((3−(√5))/8)))((((√5)−1)/4)) =−2(1−(((3−(√5))/4)))((((√5)−1)/4)) =−2((((√5)+1)/4))((((√5)−1)/4))= −(1/2) cos ((4π)/5)+cos ((2π)/5)+1=−(1/2)+1=(1/2)
$$\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{5}}\:=\:\mathrm{cos}\:\left(\pi−\frac{\pi}{\mathrm{5}}\right)=−\mathrm{cos}\:\frac{\pi}{\mathrm{5}} \\ $$$$\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{5}}\:=\:−\mathrm{cos}\:\mathrm{36}°\: \\ $$$$\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{5}}\:=\:\mathrm{cos}\:\mathrm{72}° \\ $$$$\left(\bullet\right)\:\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{5}}=\:−\mathrm{cos}\:\mathrm{36}°+\mathrm{cos}\:\:\mathrm{72}° \\ $$$$\:\:\:\:\:\:\:\:=\:−\mathrm{2sin}\:\mathrm{54}°\:\mathrm{sin}\:\mathrm{18}°\: \\ $$$$\:\:\:\:\:\:\:\:=\:−\mathrm{2cos}\:\mathrm{36}°\:\mathrm{sin}\:\mathrm{18}° \\ $$$$\:\:\:\:\:\:\:\:=\:−\mathrm{2}\left(\mathrm{1}−\mathrm{2sin}\:^{\mathrm{2}} \mathrm{18}°\right)\mathrm{sin}\:\mathrm{18}° \\ $$$$\:\:\:\:\:\:\:\:=\:−\mathrm{2}\left(\mathrm{1}−\mathrm{2}\left(\frac{\sqrt{\mathrm{5}}−\mathrm{1}}{\mathrm{4}}\right)^{\mathrm{2}} \right)\left(\frac{\sqrt{\mathrm{5}}−\mathrm{1}}{\mathrm{4}}\right) \\ $$$$\:\:\:\:\:\:\:\:=−\mathrm{2}\left(\mathrm{1}−\mathrm{2}\left(\frac{\mathrm{3}−\sqrt{\mathrm{5}}}{\mathrm{8}}\right)\right)\left(\frac{\sqrt{\mathrm{5}}−\mathrm{1}}{\mathrm{4}}\right) \\ $$$$\:\:\:\:\:\:\:=−\mathrm{2}\left(\mathrm{1}−\left(\frac{\mathrm{3}−\sqrt{\mathrm{5}}}{\mathrm{4}}\right)\right)\left(\frac{\sqrt{\mathrm{5}}−\mathrm{1}}{\mathrm{4}}\right) \\ $$$$\:\:\:\:\:\:=−\mathrm{2}\left(\frac{\sqrt{\mathrm{5}}+\mathrm{1}}{\mathrm{4}}\right)\left(\frac{\sqrt{\mathrm{5}}−\mathrm{1}}{\mathrm{4}}\right)=\:−\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\:\mathrm{cos}\:\frac{\mathrm{4}\pi}{\mathrm{5}}+\mathrm{cos}\:\frac{\mathrm{2}\pi}{\mathrm{5}}+\mathrm{1}=−\frac{\mathrm{1}}{\mathrm{2}}+\mathrm{1}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$ \\ $$$$\: \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *