Show-that-cos-6-x-sin-6-x-1-8-5-3cos-4x-By-using-the-formula-a-3-b-3-a-b-a-2-ab-b-2-

Question Number 94862 by mathocean1 last updated on 21/May/20

Show that

\cos^{6} x + {s i n}^{6} x = \frac{1}{8} (5 + 3 c o s (4 x))

By using the formula :

a^{3} + b^{3} = (a + b) (a^{2} - ab + b^{2})

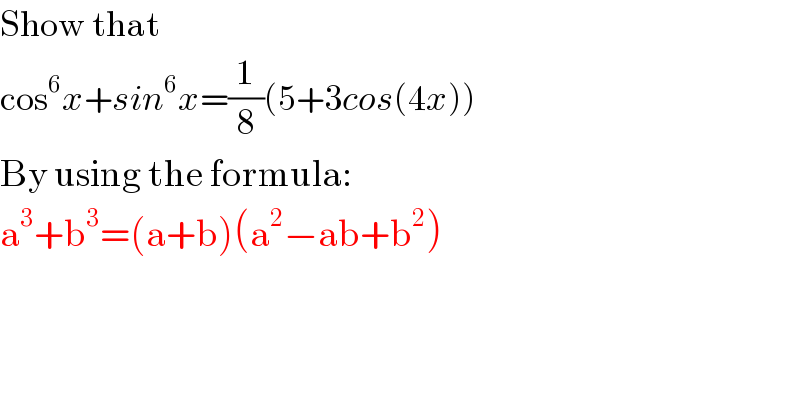
Commented by john santu last updated on 21/May/20

{(\cos^{2} x)}^{3} + {(\sin^{2} x)}^{3} =

1 \times (\cos^{4} x - \cos^{2} xsin^{2} x + \sin^{4} x)

= {{(\sin^{2} x + \cos^{2} x)}^{2} - 3 {(\cos xsin x)}^{2}}

= 1 - 3 {(\frac{1}{2} \sin 2 x)}^{2}

= 1 - \frac{3}{4} \sin^{2} 2 x

= 1 - \frac{3}{4} {\frac{1}{2} - \frac{1}{2} \cos 4 x}

= 1 - \frac{3}{8} + \frac{3}{4} \cos 4 x

= \frac{5}{8} + \frac{3}{4} \cos 4 x

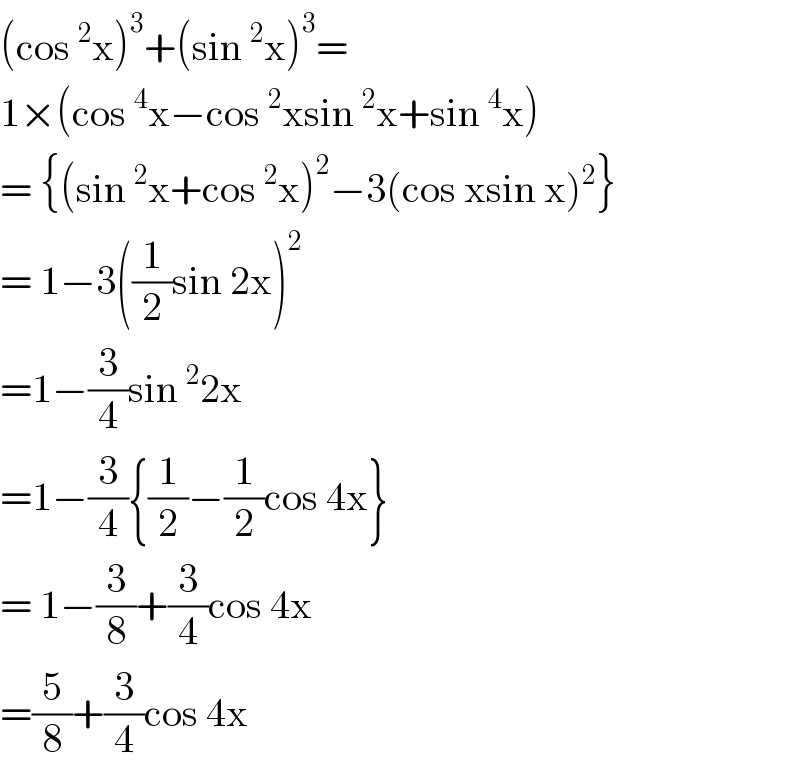
Commented by mathocean1 last updated on 21/May/20

Thank you sir!

Commented by niroj last updated on 21/May/20

��

Commented by i jagooll last updated on 21/May/20

��