Menu Close

Show-that-j-3-2-x-2-pix-sin-x-x-cos-x-

Tinku Tara 0 Comments
Pin




Question Number 82073 by TawaTawa last updated on 18/Feb/20
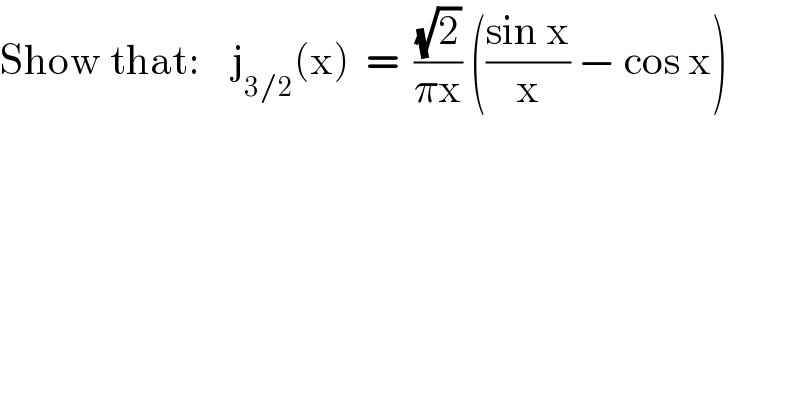
Show that: j_(3/2) (x) = ((√2)/(πx)) (((sin x)/x) − cos x)
$$\mathrm{Show}\:\mathrm{that}:\:\:\:\:\mathrm{j}_{\mathrm{3}/\mathrm{2}} \left(\mathrm{x}\right)\:\:=\:\:\frac{\sqrt{\mathrm{2}}}{\pi\mathrm{x}}\:\left(\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{x}}\:−\:\mathrm{cos}\:\mathrm{x}\right) \\ $$
Commented by jagoll last updated on 18/Feb/20
what is j_(3/2) ?
$${what}\:{is}\:{j}_{\frac{\mathrm{3}}{\mathrm{2}}} ? \\ $$
Commented by TawaTawa last updated on 18/Feb/20
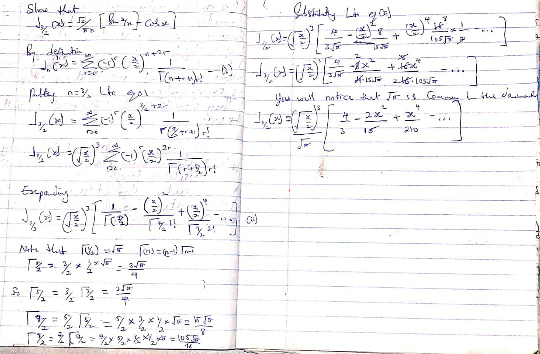
Commented by TawaTawa last updated on 18/Feb/20
Am lost here.
$$\mathrm{Am}\:\mathrm{lost}\:\mathrm{here}. \\ $$
Answered by mind is power last updated on 18/Feb/20
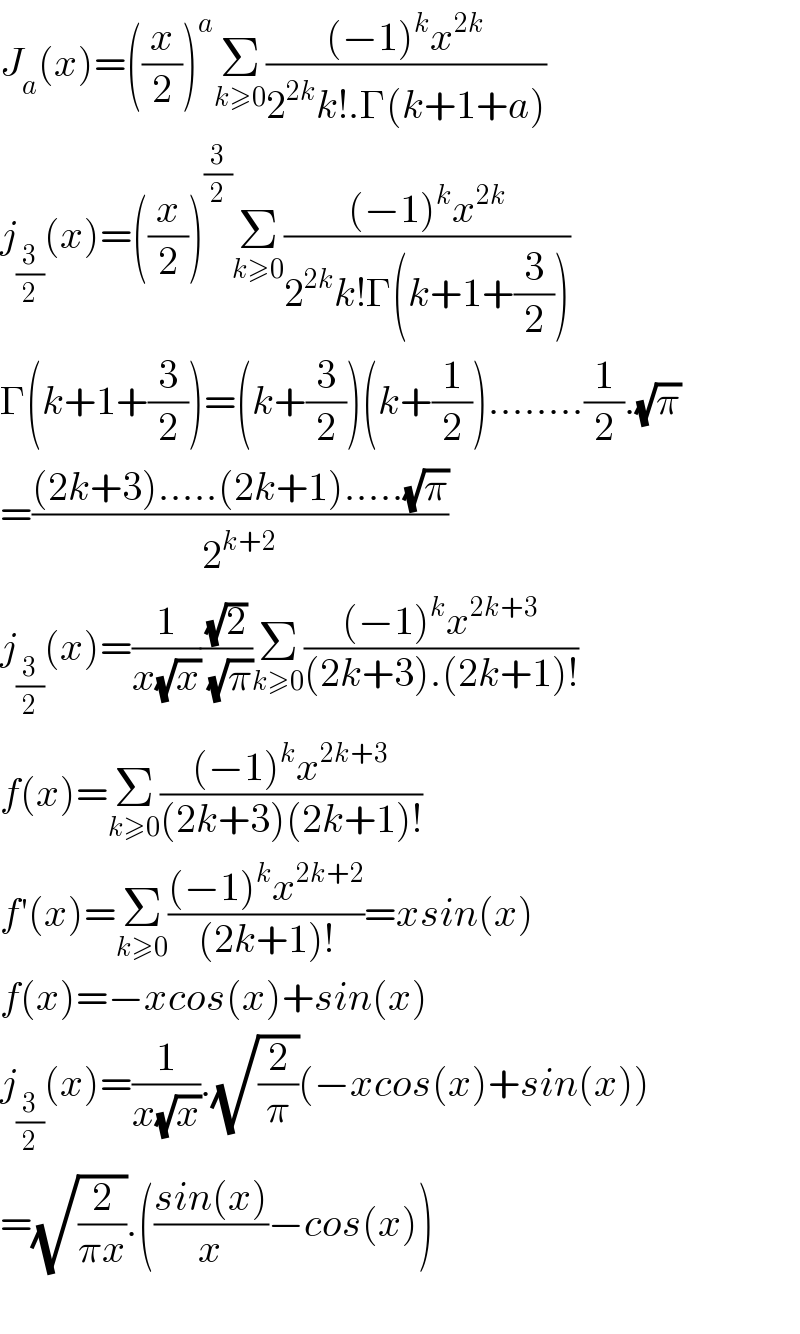
J_a (x)=((x/2))^a Σ_(k≥0) (((−1)^k x^(2k) )/(2^(2k) k!.Γ(k+1+a))) j_(3/2) (x)=((x/2))^(3/2) Σ_(k≥0) (((−1)^k x^(2k) )/(2^(2k) k!Γ(k+1+(3/2)))) Γ(k+1+(3/2))=(k+(3/2))(k+(1/2))........(1/2).(√π) =(((2k+3).....(2k+1).....(√π))/2^(k+2) ) j_(3/2) (x)=(1/(x(√x)))((√2)/( (√π)))Σ_(k≥0) (((−1)^k x^(2k+3) )/((2k+3).(2k+1)!)) f(x)=Σ_(k≥0) (((−1)^k x^(2k+3) )/((2k+3)(2k+1)!)) f′(x)=Σ_(k≥0) (((−1)^k x^(2k+2) )/((2k+1)!))=xsin(x) f(x)=−xcos(x)+sin(x) j_(3/2) (x)=(1/(x(√x))).(√(2/π))(−xcos(x)+sin(x)) =(√(2/(πx))).(((sin(x))/x)−cos(x))
$${J}_{{a}} \left({x}\right)=\left(\frac{{x}}{\mathrm{2}}\right)^{{a}} \underset{{k}\geqslant\mathrm{0}} {\sum}\frac{\left(−\mathrm{1}\right)^{{k}} {x}^{\mathrm{2}{k}} }{\mathrm{2}^{\mathrm{2}{k}} {k}!.\Gamma\left({k}+\mathrm{1}+{a}\right)} \\ $$$${j}_{\frac{\mathrm{3}}{\mathrm{2}}} \left({x}\right)=\left(\frac{{x}}{\mathrm{2}}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} \underset{{k}\geqslant\mathrm{0}} {\sum}\frac{\left(−\mathrm{1}\right)^{{k}} {x}^{\mathrm{2}{k}} }{\mathrm{2}^{\mathrm{2}{k}} {k}!\Gamma\left({k}+\mathrm{1}+\frac{\mathrm{3}}{\mathrm{2}}\right)} \\ $$$$\Gamma\left({k}+\mathrm{1}+\frac{\mathrm{3}}{\mathrm{2}}\right)=\left({k}+\frac{\mathrm{3}}{\mathrm{2}}\right)\left({k}+\frac{\mathrm{1}}{\mathrm{2}}\right)……..\frac{\mathrm{1}}{\mathrm{2}}.\sqrt{\pi} \\ $$$$=\frac{\left(\mathrm{2}{k}+\mathrm{3}\right)…..\left(\mathrm{2}{k}+\mathrm{1}\right)…..\sqrt{\pi}}{\mathrm{2}^{{k}+\mathrm{2}} } \\ $$$${j}_{\frac{\mathrm{3}}{\mathrm{2}}} \left({x}\right)=\frac{\mathrm{1}}{{x}\sqrt{{x}}}\frac{\sqrt{\mathrm{2}}}{\:\sqrt{\pi}}\underset{{k}\geqslant\mathrm{0}} {\sum}\frac{\left(−\mathrm{1}\right)^{{k}} {x}^{\mathrm{2}{k}+\mathrm{3}} }{\left(\mathrm{2}{k}+\mathrm{3}\right).\left(\mathrm{2}{k}+\mathrm{1}\right)!} \\ $$$${f}\left({x}\right)=\underset{{k}\geqslant\mathrm{0}} {\sum}\frac{\left(−\mathrm{1}\right)^{{k}} {x}^{\mathrm{2}{k}+\mathrm{3}} }{\left(\mathrm{2}{k}+\mathrm{3}\right)\left(\mathrm{2}{k}+\mathrm{1}\right)!} \\ $$$${f}'\left({x}\right)=\underset{{k}\geqslant\mathrm{0}} {\sum}\frac{\left(−\mathrm{1}\right)^{{k}} {x}^{\mathrm{2}{k}+\mathrm{2}} }{\left(\mathrm{2}{k}+\mathrm{1}\right)!}={xsin}\left({x}\right) \\ $$$${f}\left({x}\right)=−{xcos}\left({x}\right)+{sin}\left({x}\right) \\ $$$${j}_{\frac{\mathrm{3}}{\mathrm{2}}} \left({x}\right)=\frac{\mathrm{1}}{{x}\sqrt{{x}}}.\sqrt{\frac{\mathrm{2}}{\pi}}\left(−{xcos}\left({x}\right)+{sin}\left({x}\right)\right) \\ $$$$=\sqrt{\frac{\mathrm{2}}{\pi{x}}}.\left(\frac{{sin}\left({x}\right)}{{x}}−{cos}\left({x}\right)\right) \\ $$$$ \\ $$
Commented by TawaTawa last updated on 18/Feb/20
God bless you sir
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Commented by mind is power last updated on 18/Feb/20
thanx miss you too
$${thanx}\:{miss}\:{you}\:{too}\: \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *