Question Number 121052 by mathocean1 last updated on 05/Nov/20
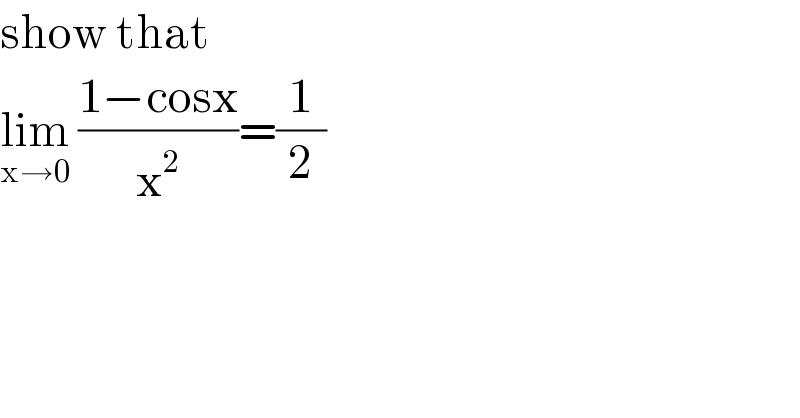
$$\mathrm{show}\:\mathrm{that} \\ $$$$\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{1}−\mathrm{cosx}}{\mathrm{x}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$ \\ $$
Answered by Dwaipayan Shikari last updated on 05/Nov/20
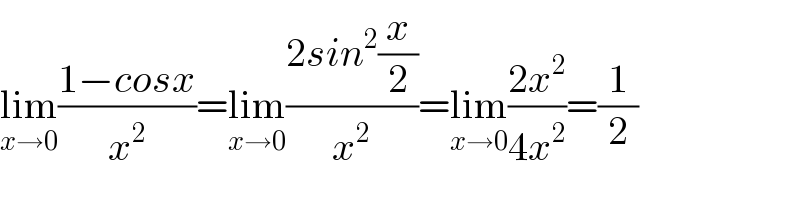
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−{cosx}}{{x}^{\mathrm{2}} }=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{2}{sin}^{\mathrm{2}} \frac{{x}}{\mathrm{2}}}{{x}^{\mathrm{2}} }=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{2}{x}^{\mathrm{2}} }{\mathrm{4}{x}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}} \\ $$
Answered by malwan last updated on 05/Nov/20

$$\underset{{x}\rightarrow\mathrm{0}} {{lim}}\:\frac{\mathrm{2}{sin}^{\mathrm{2}} \frac{{x}}{\mathrm{2}}}{{x}^{\mathrm{2}} }\:=\:\mathrm{2}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} =\:\mathrm{2}×\frac{\mathrm{1}}{\mathrm{4}}\:=\:\frac{\mathrm{1}}{\mathrm{2}} \\ $$
Answered by 675480065 last updated on 05/Nov/20

$$\mathrm{x}\rightarrow\mathrm{0},\:\mathrm{cosx}\rightarrow\mathrm{1}−\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}+\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{24}}+\mathrm{0}\left(\mathrm{x}^{\mathrm{4}} \right) \\ $$$$\Rightarrow\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−\mathrm{cosx}}{\mathrm{x}^{\mathrm{2}} }=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−\mathrm{1}+\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}−\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{24}}}{\mathrm{x}^{\mathrm{2}} }=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{x}^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{24}}\right)}{\mathrm{x}^{\mathrm{2}} } \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{24}}\right)=\frac{\mathrm{1}}{\mathrm{2}} \\ $$
