Show-that-the-angle-between-two-unit-vectors-a-and-b-is-given-by-cos-a-b-Hence-given-that-a-i-cosA-j-sinA-and-b-i-cosB-j-sinB-prove-that-cos-A-B-cos

Question Number 58312 by pete last updated on 21/Apr/19

Show that the angle θ between two unit

vectors \underset{}{\hat{a}} and \underset{}{\hat{b}} is given by \cos θ = \underset{}{\hat{a}} ∙ \underset{}{\hat{b}} .

Hence, given that \underset{}{\hat{a}} = \underset{}{i c o s A} + \underset{}{j s i n A} and

\underset{}{\hat{b}} = \underset{}{i c o s B} - \underset{}{j s i n B}, prove that \cos (A + B) =

cosAcosB - sinAsinB .

Commented by tanmay last updated on 21/Apr/19

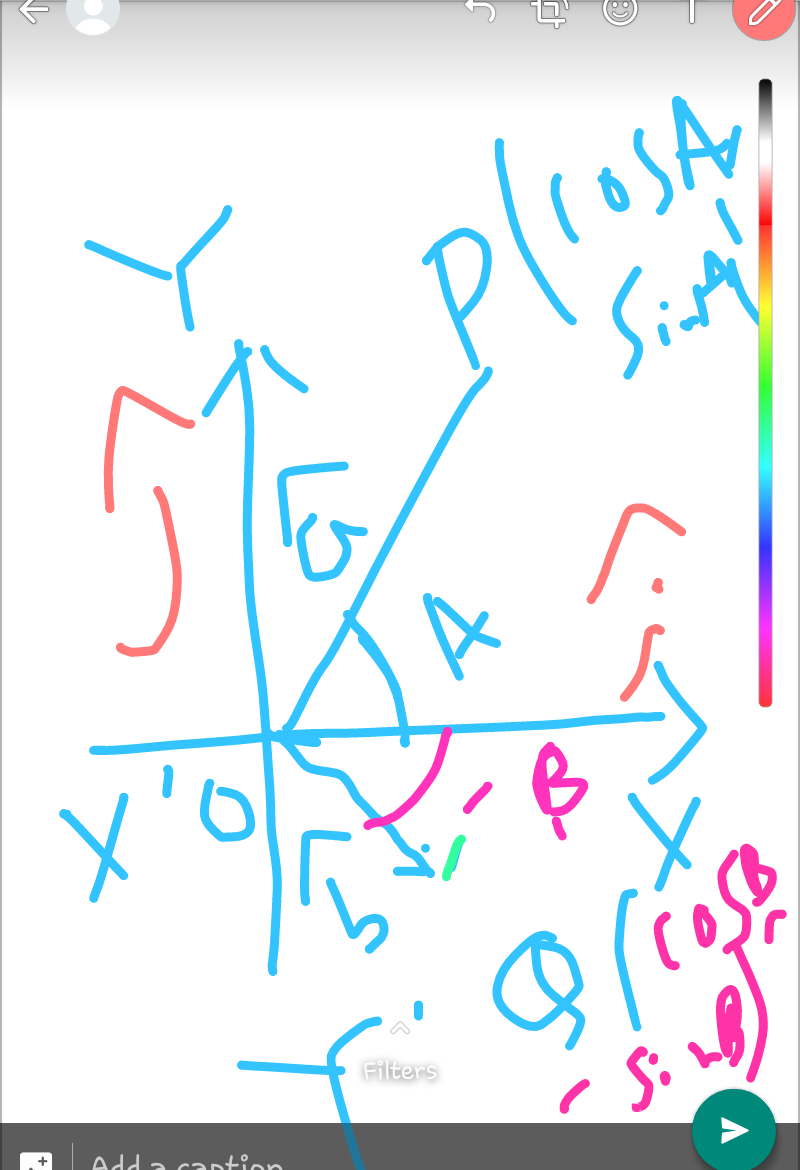
Commented by tanmay last updated on 21/Apr/19

v e c t o r o \vec{p} = \overset{\land}{a} = i c o s A + j s i n A

v e c t o r o \vec{Q} = \overset{\land}{b} = i c o s B - j s i n B

a n g l e b e t w e e n o \vec{p} a n d o \vec{Q} i s < P O Q = A + B

\overset{\land}{a} . \overset{\land}{b} =∣ \overset{\land}{a} ∣ ∣ \overset{\land}{b} ∣ c o s (A + B) = 1 \times 1 \times c o s (A + B) = c o s (A + B)

\overset{\land}{a} . \overset{\land}{b}

= (i c o s A + j s i n A) . (i c o s B - j s i n B)

= c o s A c o s B - s i n A s i n B

h e n c e e q u a t i n g v a l u e o f \overset{\land}{a} . \overset{\land}{b}

c o s (A + B) = c o s A c o s B - s i n B

f o r m u l a \dots

i f \vec{A} = {i x}_{1} + {j y}_{1} + {k z}_{1}

\vec{B} = {i x}_{2} + {j y}_{2} + {k z}_{2}

\vec{A} . \vec{B} =∣ \vec{A} ∣ ∣ \vec{B} ∣ c o s θ

∣ A ∣= \sqrt{x_{1}^{2} + y_{1}^{2} + z_{1}^{2}} a n d ∣ \vec{B} ∣= \sqrt{x_{2}^{2} + y_{2}^{2} + z_{2}^{2}}

\vec{A} . \vec{B} = x_{1} x_{2} + y_{1} y_{2} + z_{1} z_{2}

c o s θ = \frac{\vec{A} . \vec{B}}{∣ \vec{A} ∣ ∣ \vec{B} ∣} = \frac{x_{1} x_{2} + y_{1} y_{2} + z_{1} z_{2}}{\sqrt{x_{1}^{2} + y_{1}^{2} + z_{1}^{2}} \times \sqrt{x_{2}^{2} + y_{2}^{2} + z_{2}^{2}}}

Commented by pete last updated on 21/Apr/19

Thanks very much

Commented by tanmay last updated on 21/Apr/19

m o s t w e l c o m e \dots

Facebook Tweet Pin