Simplify-1-x-x-1-x-x-

Question Number 168054 by Mastermind last updated on 01/Apr/22

$${Simplify}\:\:\frac{\sqrt{\mathrm{1}+{x}}+\sqrt{{x}}}{\:\sqrt{\mathrm{1}−{x}}+\sqrt{{x}}} \\ $$

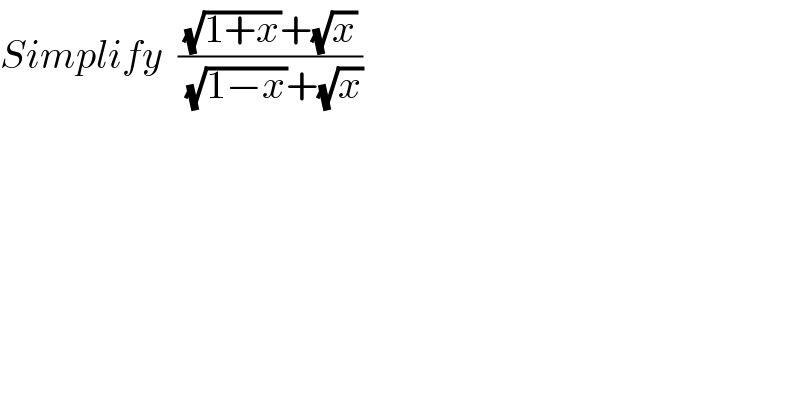
Commented by MJS_new last updated on 01/Apr/22

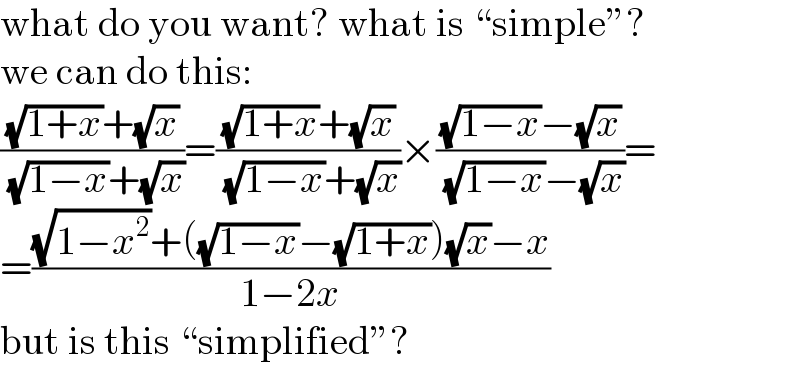
what do you want? what is “simple”? we can do this: (((√(1+x))+(√x))/( (√(1−x))+(√x)))=(((√(1+x))+(√x))/( (√(1−x))+(√x)))×(((√(1−x))−(√x))/( (√(1−x))−(√x)))= =(((√(1−x^2 ))+((√(1−x))−(√(1+x)))(√x)−x)/(1−2x)) but is this “simplified”?

$$\mathrm{what}\:\mathrm{do}\:\mathrm{you}\:\mathrm{want}?\:\mathrm{what}\:\mathrm{is}\:“\mathrm{simple}''? \\ $$$$\mathrm{we}\:\mathrm{can}\:\mathrm{do}\:\mathrm{this}: \\ $$$$\frac{\sqrt{\mathrm{1}+{x}}+\sqrt{{x}}}{\:\sqrt{\mathrm{1}−{x}}+\sqrt{{x}}}=\frac{\sqrt{\mathrm{1}+{x}}+\sqrt{{x}}}{\:\sqrt{\mathrm{1}−{x}}+\sqrt{{x}}}×\frac{\sqrt{\mathrm{1}−{x}}−\sqrt{{x}}}{\:\sqrt{\mathrm{1}−{x}}−\sqrt{{x}}}= \\ $$$$=\frac{\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }+\left(\sqrt{\mathrm{1}−{x}}−\sqrt{\mathrm{1}+{x}}\right)\sqrt{{x}}−{x}}{\mathrm{1}−\mathrm{2}{x}} \\ $$$$\mathrm{but}\:\mathrm{is}\:\mathrm{this}\:“\mathrm{simplified}''? \\ $$

Commented by Mastermind last updated on 02/Apr/22

$${You}\:{did}\:{a}\:{great}\:{job},\:{Thanks}\:{so}\:{much} \\ $$

Commented by Mastermind last updated on 02/Apr/22

$${Thanks}\:{to}\:{everyone}\: \\ $$

Answered by HeferH last updated on 01/Apr/22

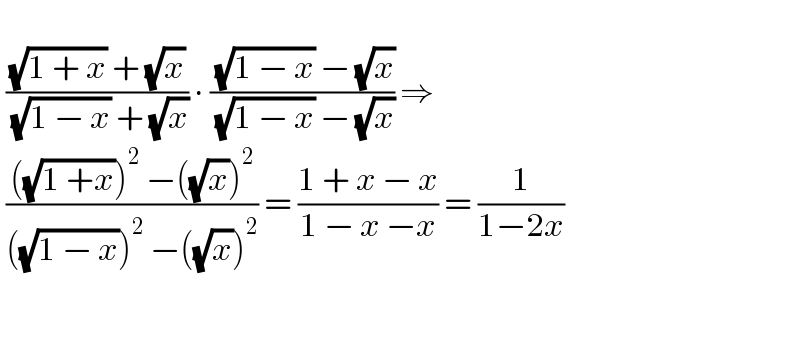
(((√(1 + x)) + (√x))/( (√(1 − x)) + (√x))) ∙ (( (√(1 − x)) − (√x))/( (√(1 − x)) − (√x))) ⇒ ((((√(1 +x)))^2 −((√x))^2 )/(((√(1 − x)))^2 −((√x))^2 )) = ((1 + x − x)/(1 − x −x)) = (1/(1−2x))

$$ \\ $$$$\:\frac{\sqrt{\mathrm{1}\:+\:{x}}\:+\:\sqrt{{x}}}{\:\sqrt{\mathrm{1}\:−\:{x}}\:+\:\sqrt{{x}}}\:\centerdot\:\frac{\:\sqrt{\mathrm{1}\:−\:{x}}\:−\:\sqrt{{x}}}{\:\sqrt{\mathrm{1}\:−\:{x}}\:−\:\sqrt{{x}}}\:\Rightarrow \\ $$$$\:\frac{\left(\sqrt{\mathrm{1}\:+{x}}\right)^{\mathrm{2}} \:−\left(\sqrt{{x}}\right)^{\mathrm{2}} }{\left(\sqrt{\mathrm{1}\:−\:{x}}\right)^{\mathrm{2}} \:−\left(\sqrt{{x}}\right)^{\mathrm{2}} }\:=\:\frac{\mathrm{1}\:+\:{x}\:−\:{x}}{\mathrm{1}\:−\:{x}\:−{x}}\:=\:\frac{\mathrm{1}}{\mathrm{1}−\mathrm{2}{x}} \\ $$$$\: \\ $$$$\: \\ $$

Commented by MJS_new last updated on 01/Apr/22

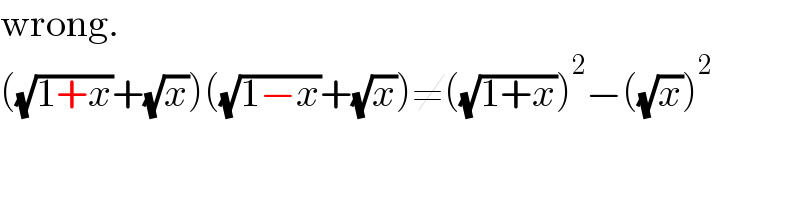
wrong. ((√(1+x))+(√x))((√(1−x))+(√x))≠((√(1+x)))^2 −((√x))^2

$$\mathrm{wrong}. \\ $$$$\left(\sqrt{\mathrm{1}+{x}}+\sqrt{{x}}\right)\left(\sqrt{\mathrm{1}−{x}}+\sqrt{{x}}\right)\neq\left(\sqrt{\mathrm{1}+{x}}\right)^{\mathrm{2}} −\left(\sqrt{{x}}\right)^{\mathrm{2}} \\ $$

Commented by HeferH last updated on 01/Apr/22

yeah, I made a mistake, sorry (didn′t realize)

$$\: \\ $$$$\:{yeah},\:{I}\:{made}\:{a}\:{mistake},\:{sorry} \\ $$$$\:\left({didn}'{t}\:{realize}\right) \\ $$$$\: \\ $$

Facebook Tweet Pin

Simplify-1-x-x-1-x-x-

Leave a Reply Cancel reply