Menu Close

Simplify-3-3-10-3-1-3-i-3-3-10-3-1-3-i-

Tinku Tara 0 Comments
Pin




Question Number 78800 by Tony Lin last updated on 20/Jan/20
Simplify: _3 (√(3+((10)/3)(√(1/3))i))+ _3 (√(3−((10)/3)(√(1/3))i))
$${Simplify}: \\ $$$$\underset{\mathrm{3}} {\:}\sqrt{\mathrm{3}+\frac{\mathrm{10}}{\mathrm{3}}\sqrt{\frac{\mathrm{1}}{\mathrm{3}}}{i}}+\underset{\mathrm{3}} {\:}\sqrt{\mathrm{3}−\frac{\mathrm{10}}{\mathrm{3}}\sqrt{\frac{\mathrm{1}}{\mathrm{3}}}{i}} \\ $$
Commented by mathmax by abdo last updated on 20/Jan/20
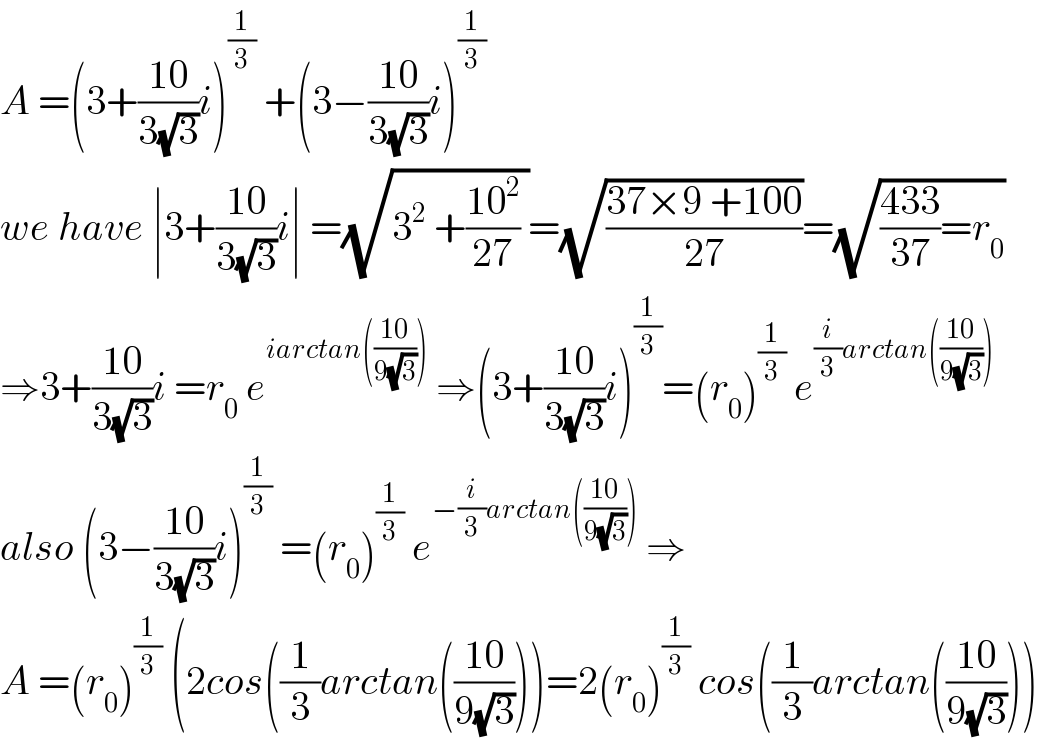
A =(3+((10)/(3(√3)))i)^(1/3) +(3−((10)/(3(√3)))i)^(1/3) we have ∣3+((10)/(3(√3)))i∣ =(√(3^2 +((10^2 )/(27)) ))=(√((37×9 +100)/(27)))=(√(((433)/(37))=r_0 )) ⇒3+((10)/(3(√3)))i =r_0 e^(iarctan(((10)/(9(√3))))) ⇒(3+((10)/(3(√3)))i)^(1/3) =(r_0 )^(1/3) e^((i/3)arctan(((10)/(9(√3))))) also (3−((10)/(3(√3)))i)^(1/3) =(r_0 )^(1/3) e^(−(i/3)arctan(((10)/(9(√3))))) ⇒ A =(r_0 )^(1/3) (2cos((1/3)arctan(((10)/(9(√3)))))=2(r_0 )^(1/3) cos((1/3)arctan(((10)/(9(√3)))))
$${A}\:=\left(\mathrm{3}+\frac{\mathrm{10}}{\mathrm{3}\sqrt{\mathrm{3}}}{i}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:+\left(\mathrm{3}−\frac{\mathrm{10}}{\mathrm{3}\sqrt{\mathrm{3}}}{i}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} \\ $$$${we}\:{have}\:\mid\mathrm{3}+\frac{\mathrm{10}}{\mathrm{3}\sqrt{\mathrm{3}}}{i}\mid\:=\sqrt{\mathrm{3}^{\mathrm{2}} \:+\frac{\mathrm{10}^{\mathrm{2}} }{\mathrm{27}}\:}=\sqrt{\frac{\mathrm{37}×\mathrm{9}\:+\mathrm{100}}{\mathrm{27}}}=\sqrt{\frac{\mathrm{433}}{\mathrm{37}}={r}_{\mathrm{0}} } \\ $$$$\Rightarrow\mathrm{3}+\frac{\mathrm{10}}{\mathrm{3}\sqrt{\mathrm{3}}}{i}\:={r}_{\mathrm{0}} \:{e}^{{iarctan}\left(\frac{\mathrm{10}}{\mathrm{9}\sqrt{\mathrm{3}}}\right)} \:\Rightarrow\left(\mathrm{3}+\frac{\mathrm{10}}{\mathrm{3}\sqrt{\mathrm{3}}}{i}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} =\left({r}_{\mathrm{0}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:{e}^{\frac{{i}}{\mathrm{3}}{arctan}\left(\frac{\mathrm{10}}{\mathrm{9}\sqrt{\mathrm{3}}}\right)} \\ $$$${also}\:\left(\mathrm{3}−\frac{\mathrm{10}}{\mathrm{3}\sqrt{\mathrm{3}}}{i}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:=\left({r}_{\mathrm{0}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:{e}^{−\frac{{i}}{\mathrm{3}}{arctan}\left(\frac{\mathrm{10}}{\mathrm{9}\sqrt{\mathrm{3}}}\right)} \:\Rightarrow \\ $$$${A}\:=\left({r}_{\mathrm{0}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:\left(\mathrm{2}{cos}\left(\frac{\mathrm{1}}{\mathrm{3}}{arctan}\left(\frac{\mathrm{10}}{\mathrm{9}\sqrt{\mathrm{3}}}\right)\right)=\mathrm{2}\left({r}_{\mathrm{0}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:{cos}\left(\frac{\mathrm{1}}{\mathrm{3}}{arctan}\left(\frac{\mathrm{10}}{\mathrm{9}\sqrt{\mathrm{3}}}\right)\right)\right. \\ $$
Commented by Tony Lin last updated on 21/Jan/20
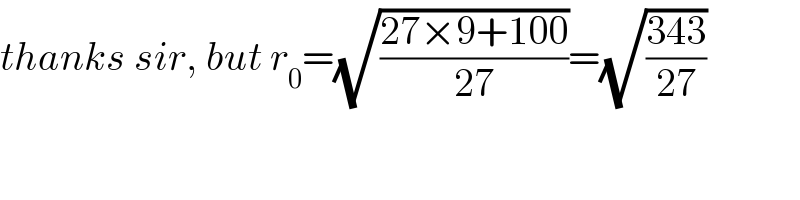
thanks sir, but r_0 =(√((27×9+100)/(27)))=(√((343)/(27)))
$${thanks}\:{sir},\:{but}\:{r}_{\mathrm{0}} =\sqrt{\frac{\mathrm{27}×\mathrm{9}+\mathrm{100}}{\mathrm{27}}}=\sqrt{\frac{\mathrm{343}}{\mathrm{27}}} \\ $$
Commented by mathmax by abdo last updated on 21/Jan/20
yes
$${yes} \\ $$
Answered by MJS last updated on 20/Jan/20
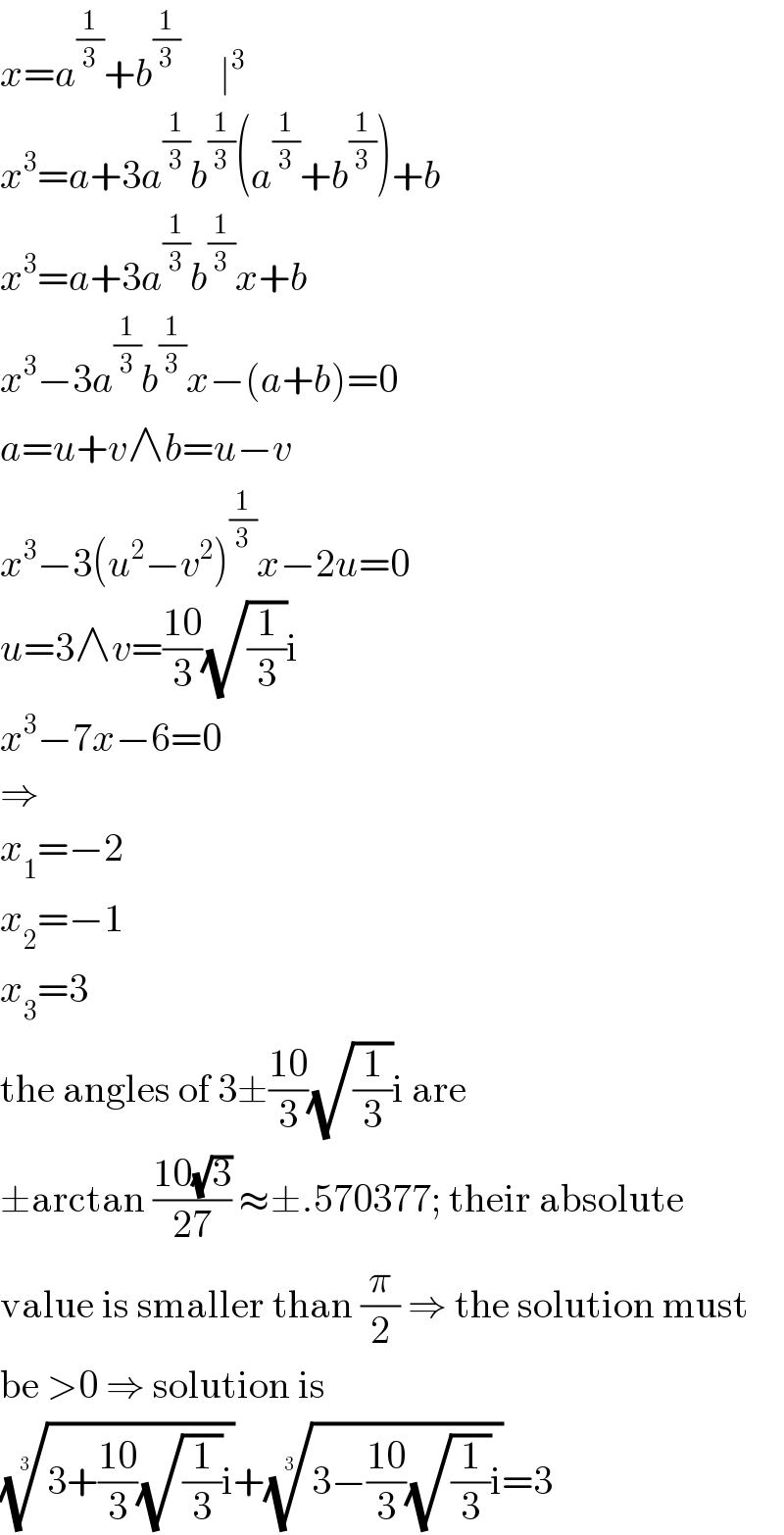
x=a^(1/3) +b^(1/3) ∣^3 x^3 =a+3a^(1/3) b^(1/3) (a^(1/3) +b^(1/3) )+b x^3 =a+3a^(1/3) b^(1/3) x+b x^3 −3a^(1/3) b^(1/3) x−(a+b)=0 a=u+v∧b=u−v x^3 −3(u^2 −v^2 )^(1/3) x−2u=0 u=3∧v=((10)/3)(√(1/3))i x^3 −7x−6=0 ⇒ x_1 =−2 x_2 =−1 x_3 =3 the angles of 3±((10)/3)(√(1/3))i are ±arctan ((10(√3))/(27)) ≈±.570377; their absolute value is smaller than (π/2) ⇒ the solution must be >0 ⇒ solution is ((3+((10)/3)(√(1/3))i))^(1/3) +((3−((10)/3)(√(1/3))i))^(1/3) =3
$${x}={a}^{\frac{\mathrm{1}}{\mathrm{3}}} +{b}^{\frac{\mathrm{1}}{\mathrm{3}}} \:\:\:\:\:\mid^{\mathrm{3}} \\ $$$${x}^{\mathrm{3}} ={a}+\mathrm{3}{a}^{\frac{\mathrm{1}}{\mathrm{3}}} {b}^{\frac{\mathrm{1}}{\mathrm{3}}} \left({a}^{\frac{\mathrm{1}}{\mathrm{3}}} +{b}^{\frac{\mathrm{1}}{\mathrm{3}}} \right)+{b} \\ $$$${x}^{\mathrm{3}} ={a}+\mathrm{3}{a}^{\frac{\mathrm{1}}{\mathrm{3}}} {b}^{\frac{\mathrm{1}}{\mathrm{3}}} {x}+{b} \\ $$$${x}^{\mathrm{3}} −\mathrm{3}{a}^{\frac{\mathrm{1}}{\mathrm{3}}} {b}^{\frac{\mathrm{1}}{\mathrm{3}}} {x}−\left({a}+{b}\right)=\mathrm{0} \\ $$$${a}={u}+{v}\wedge{b}={u}−{v} \\ $$$${x}^{\mathrm{3}} −\mathrm{3}\left({u}^{\mathrm{2}} −{v}^{\mathrm{2}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} {x}−\mathrm{2}{u}=\mathrm{0} \\ $$$${u}=\mathrm{3}\wedge{v}=\frac{\mathrm{10}}{\mathrm{3}}\sqrt{\frac{\mathrm{1}}{\mathrm{3}}}\mathrm{i} \\ $$$${x}^{\mathrm{3}} −\mathrm{7}{x}−\mathrm{6}=\mathrm{0} \\ $$$$\Rightarrow \\ $$$${x}_{\mathrm{1}} =−\mathrm{2} \\ $$$${x}_{\mathrm{2}} =−\mathrm{1} \\ $$$${x}_{\mathrm{3}} =\mathrm{3} \\ $$$$\mathrm{the}\:\mathrm{angles}\:\mathrm{of}\:\mathrm{3}\pm\frac{\mathrm{10}}{\mathrm{3}}\sqrt{\frac{\mathrm{1}}{\mathrm{3}}}\mathrm{i}\:\mathrm{are} \\ $$$$\pm\mathrm{arctan}\:\frac{\mathrm{10}\sqrt{\mathrm{3}}}{\mathrm{27}}\:\approx\pm.\mathrm{570377};\:\mathrm{their}\:\mathrm{absolute} \\ $$$$\mathrm{value}\:\mathrm{is}\:\mathrm{smaller}\:\mathrm{than}\:\frac{\pi}{\mathrm{2}}\:\Rightarrow\:\mathrm{the}\:\mathrm{solution}\:\mathrm{must} \\ $$$$\mathrm{be}\:>\mathrm{0}\:\Rightarrow\:\mathrm{solution}\:\mathrm{is} \\ $$$$\sqrt[{\mathrm{3}}]{\mathrm{3}+\frac{\mathrm{10}}{\mathrm{3}}\sqrt{\frac{\mathrm{1}}{\mathrm{3}}}\mathrm{i}}+\sqrt[{\mathrm{3}}]{\mathrm{3}−\frac{\mathrm{10}}{\mathrm{3}}\sqrt{\frac{\mathrm{1}}{\mathrm{3}}}\mathrm{i}}=\mathrm{3} \\ $$
Commented by Tony Lin last updated on 21/Jan/20
thanks sir, but if we originally use Cardano′s method to solve x^3 +px+q=0 how can we simplify the answer to become more beautiful without using factorization or what we can do is only cube it and solve it in a complicated and slow way
$${thanks}\:{sir},\:{but}\:{if}\:{we}\:{originally}\:{use}\: \\ $$$${Cardano}'{s}\:{method}\:{to}\:{solve}\: \\ $$$${x}^{\mathrm{3}} +{px}+{q}=\mathrm{0} \\ $$$${how}\:{can}\:{we}\:{simplify}\:{the}\:{answer}\:{to} \\ $$$${become}\:{more}\:{beautiful}\:{without}\:{using} \\ $$$${factorization} \\ $$$${or}\:{what}\:{we}\:{can}\:{do}\:{is}\:{only}\:{cube}\:{it} \\ $$$${and}\:{solve}\:{it}\:{in}\:{a}\:{complicated}\:{and} \\ $$$${slow}\:{way} \\ $$
Commented by MJS last updated on 21/Jan/20
Cardano doesn′t work when the equation has 3 real roots. x^3 +px+q=0 first, always check all factors of ±q (x−α)(x−β)(x−γ)=x^3 +...−αβγ ⇒ q=−αβγ if we have solutions ∈Z or ∈Q we can simply find them second, calculate D=(p^3 /(27))+(q^2 /4) D≥0 ⇒ Cardano D<0 ⇒ trigonometric solution x_(k+1) =(2/3)(√(−3p))sin ((1/3)(2kπ+arcsin ((9q)/(2(√(−3p^3 )))))) with k=0, 1, 2 the problem with both methods is that the solutions “hide” easier ones like x=(√3)+(√2) but there′s no other way
$$\mathrm{Cardano}\:\mathrm{doesn}'\mathrm{t}\:\mathrm{work}\:\mathrm{when}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{has} \\ $$$$\mathrm{3}\:\mathrm{real}\:\mathrm{roots}. \\ $$$${x}^{\mathrm{3}} +{px}+{q}=\mathrm{0} \\ $$$$\mathrm{first},\:\mathrm{always}\:\mathrm{check}\:\mathrm{all}\:\mathrm{factors}\:\mathrm{of}\:\pm{q} \\ $$$$\left({x}−\alpha\right)\left({x}−\beta\right)\left({x}−\gamma\right)={x}^{\mathrm{3}} +…−\alpha\beta\gamma \\ $$$$\Rightarrow\:{q}=−\alpha\beta\gamma \\ $$$$\mathrm{if}\:\mathrm{we}\:\mathrm{have}\:\mathrm{solutions}\:\in\mathbb{Z}\:\mathrm{or}\:\in\mathbb{Q}\:\mathrm{we}\:\mathrm{can}\:\mathrm{simply} \\ $$$$\mathrm{find}\:\mathrm{them} \\ $$$$\mathrm{second},\:\mathrm{calculate}\:{D}=\frac{{p}^{\mathrm{3}} }{\mathrm{27}}+\frac{{q}^{\mathrm{2}} }{\mathrm{4}} \\ $$$${D}\geqslant\mathrm{0}\:\Rightarrow\:\mathrm{Cardano} \\ $$$${D}<\mathrm{0}\:\Rightarrow\:\mathrm{trigonometric}\:\mathrm{solution} \\ $$$${x}_{{k}+\mathrm{1}} =\frac{\mathrm{2}}{\mathrm{3}}\sqrt{−\mathrm{3}{p}}\mathrm{sin}\:\left(\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{2}{k}\pi+\mathrm{arcsin}\:\frac{\mathrm{9}{q}}{\mathrm{2}\sqrt{−\mathrm{3}{p}^{\mathrm{3}} }}\right)\right) \\ $$$$\mathrm{with}\:{k}=\mathrm{0},\:\mathrm{1},\:\mathrm{2} \\ $$$$\mathrm{the}\:\mathrm{problem}\:\mathrm{with}\:\mathrm{both}\:\mathrm{methods}\:\mathrm{is}\:\mathrm{that}\:\mathrm{the} \\ $$$$\mathrm{solutions}\:“\mathrm{hide}''\:\mathrm{easier}\:\mathrm{ones}\:\mathrm{like}\:{x}=\sqrt{\mathrm{3}}+\sqrt{\mathrm{2}} \\ $$$$\mathrm{but}\:\mathrm{there}'\mathrm{s}\:\mathrm{no}\:\mathrm{other}\:\mathrm{way} \\ $$
Commented by MJS last updated on 21/Jan/20
x^3 −7x−6=0 in this case, first try factors of ±6: {−6, −3, −2, −1, 1, 2, 3, 6} and you′re done Cardano doesn′t work because D=(((−7)^3 )/(27))+(((−6)^2 )/4)=−((100)/(27))<0 trigonometric solution gives x_1 =−((√(21))/3)sin ((1/3)arcsin ((9(√(21)))/(49))) x_2 =((2(√(21)))/3)sin ((π/3)+arcsin ((9(√(21)))/(49))) x_3 =−((2(√(21)))/3)cos ((π/6)+arcsin ((9(√(21)))/(49))) and it′s not easy to show x_1 =−1 x_2 =3 x_3 =−2
$${x}^{\mathrm{3}} −\mathrm{7}{x}−\mathrm{6}=\mathrm{0} \\ $$$$\mathrm{in}\:\mathrm{this}\:\mathrm{case},\:\mathrm{first}\:\mathrm{try}\:\mathrm{factors}\:\mathrm{of}\:\pm\mathrm{6}: \\ $$$$\left\{−\mathrm{6},\:−\mathrm{3},\:−\mathrm{2},\:−\mathrm{1},\:\mathrm{1},\:\mathrm{2},\:\mathrm{3},\:\mathrm{6}\right\} \\ $$$$\mathrm{and}\:\mathrm{you}'\mathrm{re}\:\mathrm{done} \\ $$$$\mathrm{Cardano}\:\mathrm{doesn}'\mathrm{t}\:\mathrm{work}\:\mathrm{because} \\ $$$${D}=\frac{\left(−\mathrm{7}\right)^{\mathrm{3}} }{\mathrm{27}}+\frac{\left(−\mathrm{6}\right)^{\mathrm{2}} }{\mathrm{4}}=−\frac{\mathrm{100}}{\mathrm{27}}<\mathrm{0} \\ $$$$\mathrm{trigonometric}\:\mathrm{solution}\:\mathrm{gives} \\ $$$${x}_{\mathrm{1}} =−\frac{\sqrt{\mathrm{21}}}{\mathrm{3}}\mathrm{sin}\:\left(\frac{\mathrm{1}}{\mathrm{3}}\mathrm{arcsin}\:\frac{\mathrm{9}\sqrt{\mathrm{21}}}{\mathrm{49}}\right) \\ $$$${x}_{\mathrm{2}} =\frac{\mathrm{2}\sqrt{\mathrm{21}}}{\mathrm{3}}\mathrm{sin}\:\left(\frac{\pi}{\mathrm{3}}+\mathrm{arcsin}\:\frac{\mathrm{9}\sqrt{\mathrm{21}}}{\mathrm{49}}\right) \\ $$$${x}_{\mathrm{3}} =−\frac{\mathrm{2}\sqrt{\mathrm{21}}}{\mathrm{3}}\mathrm{cos}\:\left(\frac{\pi}{\mathrm{6}}+\mathrm{arcsin}\:\frac{\mathrm{9}\sqrt{\mathrm{21}}}{\mathrm{49}}\right) \\ $$$$\mathrm{and}\:\mathrm{it}'\mathrm{s}\:\mathrm{not}\:\mathrm{easy}\:\mathrm{to}\:\mathrm{show} \\ $$$${x}_{\mathrm{1}} =−\mathrm{1} \\ $$$${x}_{\mathrm{2}} =\mathrm{3} \\ $$$${x}_{\mathrm{3}} =−\mathrm{2} \\ $$
Commented by jagoll last updated on 21/Jan/20
waw..i understand sir. thanks sir
$$\mathrm{waw}..\mathrm{i}\:\mathrm{understand}\:\mathrm{sir}.\:\mathrm{thanks}\:\mathrm{sir} \\ $$
Commented by Tony Lin last updated on 21/Jan/20
thanks sir,and I found that if I use the result from mathmax ((3+((10)/3)(√(1/3))i))^(1/3) +((3−((10)/3)(√(1/3))i))^(1/3) =2(√(7/3))cos[(1/3)arctan(((10)/(9(√3))))] let arctan(((10)/(9(√3))))=θ⇒tanθ=((10)/(9(√3))) ∴cosθ=((9(√3))/(7(√7)))=4cos^3 (θ/3)−3cos(θ/3) let cos(θ/3)=t⇒4t^3 −3t=((9(√3))/(7(√7))) ⇒28(√7)t^3 −21(√7)t−9(√3)=0 ⇒(2(√7)t−3(√3))(14t^2 +3(√(21))t+3)=0 ∴t=(3/2)(√(3/7)) ∴2(√(7/3))cos[(1/3)arctan(((10)/(9(√3))))] =((3+((10)/3)(√(1/3))i))^(1/3) +((3−((10)/3)(√(1/3))i))^(1/3) =3
$${thanks}\:{sir},{and}\:{I}\:{found}\:{that} \\ $$$${if}\:{I}\:{use}\:{the}\:{result}\:{from}\:{mathmax} \\ $$$$\sqrt[{\mathrm{3}}]{\mathrm{3}+\frac{\mathrm{10}}{\mathrm{3}}\sqrt{\frac{\mathrm{1}}{\mathrm{3}}}{i}}+\sqrt[{\mathrm{3}}]{\mathrm{3}−\frac{\mathrm{10}}{\mathrm{3}}\sqrt{\frac{\mathrm{1}}{\mathrm{3}}}{i}} \\ $$$$=\mathrm{2}\sqrt{\frac{\mathrm{7}}{\mathrm{3}}}{cos}\left[\frac{\mathrm{1}}{\mathrm{3}}{arctan}\left(\frac{\mathrm{10}}{\mathrm{9}\sqrt{\mathrm{3}}}\right)\right] \\ $$$${let}\:{arctan}\left(\frac{\mathrm{10}}{\mathrm{9}\sqrt{\mathrm{3}}}\right)=\theta\Rightarrow{tan}\theta=\frac{\mathrm{10}}{\mathrm{9}\sqrt{\mathrm{3}}} \\ $$$$\therefore{cos}\theta=\frac{\mathrm{9}\sqrt{\mathrm{3}}}{\mathrm{7}\sqrt{\mathrm{7}}}=\mathrm{4}{cos}^{\mathrm{3}} \frac{\theta}{\mathrm{3}}−\mathrm{3}{cos}\frac{\theta}{\mathrm{3}} \\ $$$${let}\:{cos}\frac{\theta}{\mathrm{3}}={t}\Rightarrow\mathrm{4}{t}^{\mathrm{3}} −\mathrm{3}{t}=\frac{\mathrm{9}\sqrt{\mathrm{3}}}{\mathrm{7}\sqrt{\mathrm{7}}} \\ $$$$\Rightarrow\mathrm{28}\sqrt{\mathrm{7}}{t}^{\mathrm{3}} −\mathrm{21}\sqrt{\mathrm{7}}{t}−\mathrm{9}\sqrt{\mathrm{3}}=\mathrm{0} \\ $$$$\Rightarrow\left(\mathrm{2}\sqrt{\mathrm{7}}{t}−\mathrm{3}\sqrt{\mathrm{3}}\right)\left(\mathrm{14}{t}^{\mathrm{2}} +\mathrm{3}\sqrt{\mathrm{21}}{t}+\mathrm{3}\right)=\mathrm{0} \\ $$$$\therefore{t}=\frac{\mathrm{3}}{\mathrm{2}}\sqrt{\frac{\mathrm{3}}{\mathrm{7}}} \\ $$$$\therefore\mathrm{2}\sqrt{\frac{\mathrm{7}}{\mathrm{3}}}{cos}\left[\frac{\mathrm{1}}{\mathrm{3}}{arctan}\left(\frac{\mathrm{10}}{\mathrm{9}\sqrt{\mathrm{3}}}\right)\right] \\ $$$$=\sqrt[{\mathrm{3}}]{\mathrm{3}+\frac{\mathrm{10}}{\mathrm{3}}\sqrt{\frac{\mathrm{1}}{\mathrm{3}}}{i}}+\sqrt[{\mathrm{3}}]{\mathrm{3}−\frac{\mathrm{10}}{\mathrm{3}}\sqrt{\frac{\mathrm{1}}{\mathrm{3}}}{i}} \\ $$$$=\mathrm{3} \\ $$
Commented by msup trace by abdo last updated on 21/Jan/20
thank you for completing the answer
$${thank}\:{you}\:{for}\:{completing}\:{the}\:{answer} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *