simplify-sin-1-cot-cos-1-tan-

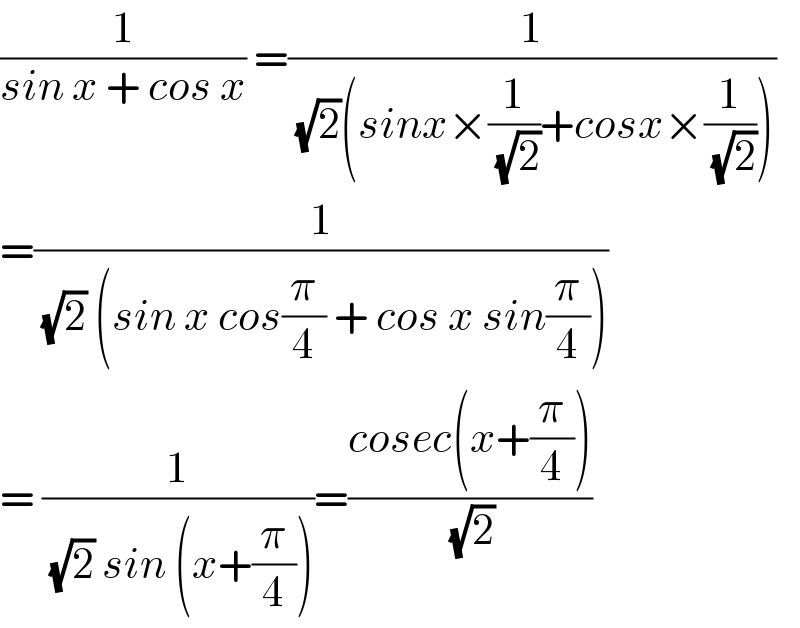
Question Number 105285 by bemath last updated on 27/Jul/20

s i m p l i f y \frac{\sin θ}{1 + \cot θ} + \frac{\cos θ}{1 + \tan θ} ?

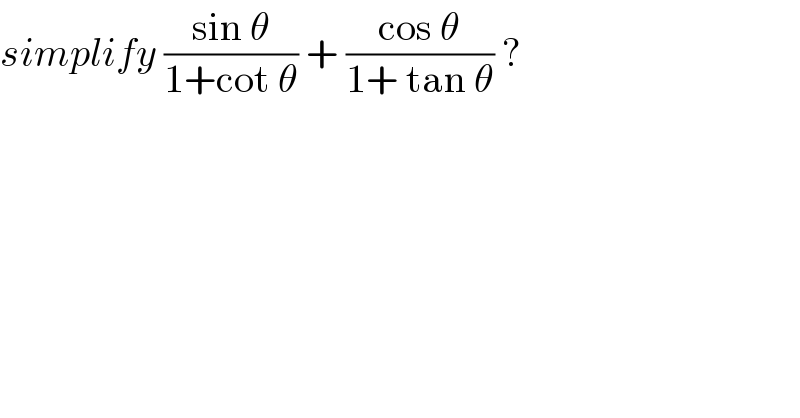
Commented by bemath last updated on 27/Jul/20

t h a n k y o u

Commented by som(math1967) last updated on 27/Jul/20

\frac{\sin θ}{1 + \frac{\cos θ}{\sin θ}} + \frac{\cos θ}{1 + \frac{\sin θ}{\cos θ}}

= \frac{\sin^{2} θ}{\sin θ + \cos θ} + \frac{\cos^{2} θ}{\cos θ + \sin θ}

= \frac{1}{\sin θ + \cos θ} [∵ \sin^{2} θ + \cos^{2} θ = 1]

Answered by bobhans last updated on 27/Jul/20

\Leftrightarrow \frac{\sin^{2} x}{\sin x + \cos x} + \frac{\cos^{2}}{\cos x + \sin x} = \frac{1}{\sin x + \cos x}

= \frac{1}{\sqrt{2} \cos (x - \frac{π}{4})} = \frac{\sec (x - \frac{π}{4})}{\sqrt{2}} ▹

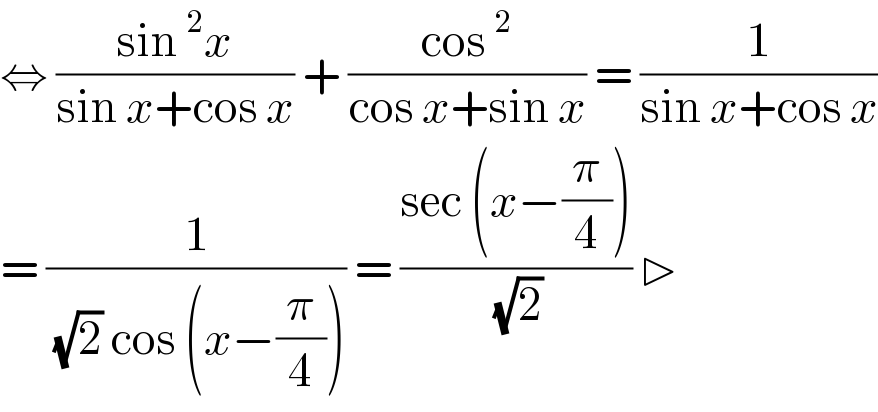
Commented by malwaan last updated on 27/Jul/20

\frac{1}{s i n x + c o s x} = \frac{1}{\sqrt{2} (s i n x \times \frac{1}{\sqrt{2}} + c o s x \times \frac{1}{\sqrt{2}})}

= \frac{1}{\sqrt{2} (s i n x c o s \frac{π}{4} + c o s x s i n \frac{π}{4})}

= \frac{1}{\sqrt{2} s i n (x + \frac{π}{4})} = \frac{c o s e c (x + \frac{π}{4})}{\sqrt{2}}

Facebook Tweet Pin