Question Number 92585 by john santu last updated on 08/May/20
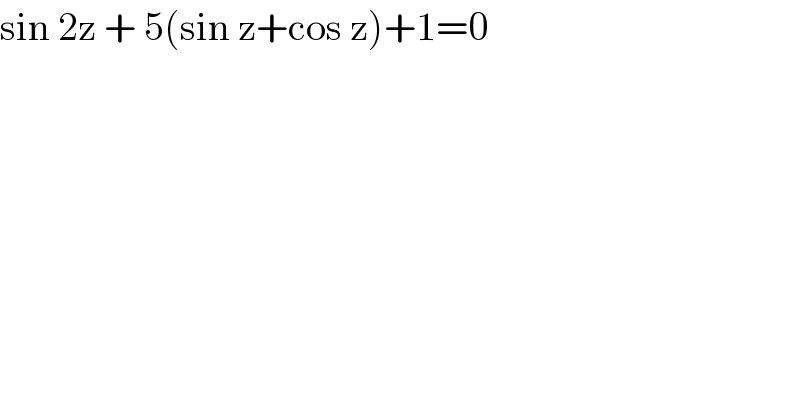
$$\mathrm{sin}\:\mathrm{2z}\:+\:\mathrm{5}\left(\mathrm{sin}\:\mathrm{z}+\mathrm{cos}\:\mathrm{z}\right)+\mathrm{1}=\mathrm{0} \\ $$
Commented by john santu last updated on 08/May/20
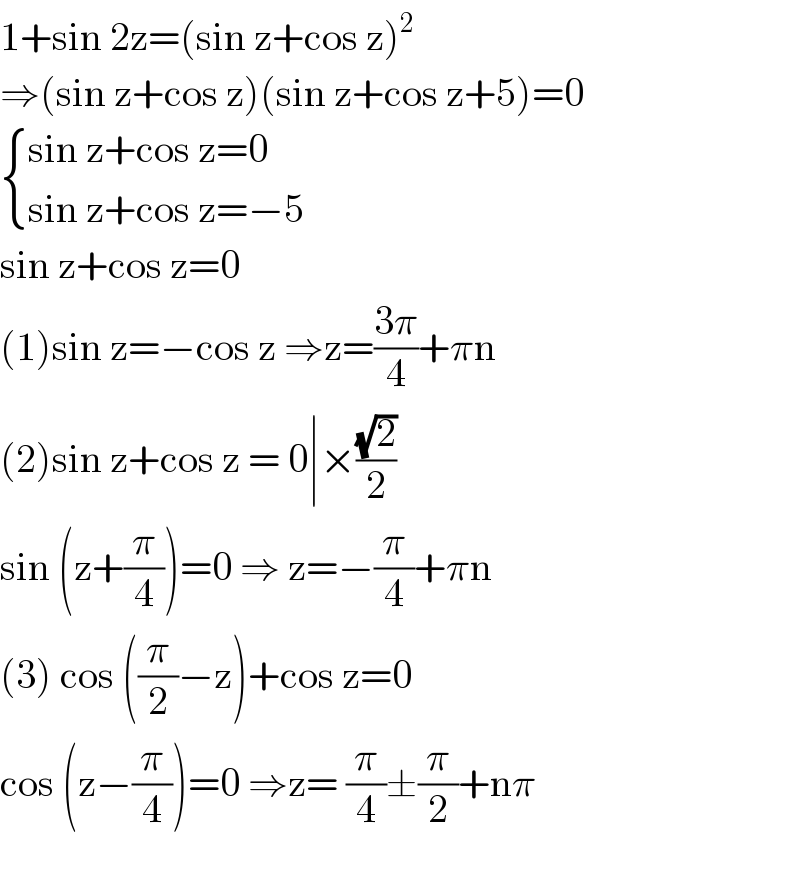
$$\mathrm{1}+\mathrm{sin}\:\mathrm{2z}=\left(\mathrm{sin}\:\mathrm{z}+\mathrm{cos}\:\mathrm{z}\right)^{\mathrm{2}} \\ $$$$\Rightarrow\left(\mathrm{sin}\:\mathrm{z}+\mathrm{cos}\:\mathrm{z}\right)\left(\mathrm{sin}\:\mathrm{z}+\mathrm{cos}\:\mathrm{z}+\mathrm{5}\right)=\mathrm{0} \\ $$$$\begin{cases}{\mathrm{sin}\:\mathrm{z}+\mathrm{cos}\:\mathrm{z}=\mathrm{0}}\\{\mathrm{sin}\:\mathrm{z}+\mathrm{cos}\:\mathrm{z}=−\mathrm{5}}\end{cases} \\ $$$$\mathrm{sin}\:\mathrm{z}+\mathrm{cos}\:\mathrm{z}=\mathrm{0} \\ $$$$\left(\mathrm{1}\right)\mathrm{sin}\:\mathrm{z}=−\mathrm{cos}\:\mathrm{z}\:\Rightarrow\mathrm{z}=\frac{\mathrm{3}\pi}{\mathrm{4}}+\pi\mathrm{n} \\ $$$$\left(\mathrm{2}\right)\mathrm{sin}\:\mathrm{z}+\mathrm{cos}\:\mathrm{z}\:=\:\mathrm{0}\mid×\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$$\mathrm{sin}\:\left(\mathrm{z}+\frac{\pi}{\mathrm{4}}\right)=\mathrm{0}\:\Rightarrow\:\mathrm{z}=−\frac{\pi}{\mathrm{4}}+\pi\mathrm{n} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{cos}\:\left(\frac{\pi}{\mathrm{2}}−\mathrm{z}\right)+\mathrm{cos}\:\mathrm{z}=\mathrm{0} \\ $$$$\mathrm{cos}\:\left(\mathrm{z}−\frac{\pi}{\mathrm{4}}\right)=\mathrm{0}\:\Rightarrow\mathrm{z}=\:\frac{\pi}{\mathrm{4}}\pm\frac{\pi}{\mathrm{2}}+\mathrm{n}\pi \\ $$$$ \\ $$
