solve-1-x-1-y-1-z-3-4-with-x-y-z-N-

Question Number 118322 by mr W last updated on 16/Oct/20

s o l v e

\frac{1}{x} + \frac{1}{y} + \frac{1}{z} = \frac{3}{4}

w i t h x, y, z \in N

Commented by malwaan last updated on 17/Oct/20

\frac{y z + x z + x y}{x y z} = \frac{3}{4} (x \neq 0; y \neq 0; z \neq 0)

1) x = y = z

\Rightarrow \frac{3 x^{2}}{x^{3}} = \frac{3}{4} \Rightarrow \frac{3}{x} = \frac{3}{4}

\Rightarrow x = y = z = 4

2) i f x = 1 \Rightarrow \frac{1}{y} + \frac{1}{z} = - \frac{1}{4} {w r o n g}

s o x > 1; y > 1; z > 1

3) x = 2 \Rightarrow \frac{1}{y} + \frac{1}{z} = \frac{1}{4}

\Rightarrow y = 8; z = 8

4) x = 3 \Rightarrow \frac{1}{y} + \frac{1}{z} = \frac{5}{12}

\frac{y + z}{y z} = \frac{5}{12} {n o s o l u t i o n s}

s o x \neq 3; y \neq 3; z \neq 3

Commented by MJS_new last updated on 17/Oct/20

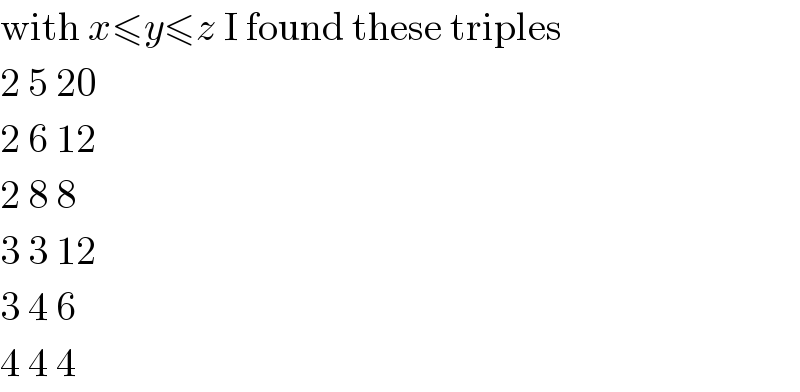
with x ⩽ y ⩽ z I found these triples

2 5 20

2 6 12

2 8 8

3 3 12

3 4 6

4 4 4

Answered by TANMAY PANACEA last updated on 16/Oct/20

x = y = z = 4

x = 2 y = z = 8

i t i s n o t s o l u t i o n . . b y t r i a l

Answered by 1549442205PVT last updated on 17/Oct/20

\frac{1}{x} + \frac{1}{y} + \frac{1}{z} = \frac{3}{4} (1)

Since x, y, z have equal role in the equality

, WLOG we can assume that x ⩾ y ⩾ z > 1

Then \frac{1}{x} ⩽ \frac{1}{y} ⩽ \frac{1}{z} < 1 . Hence

\frac{3}{4} = \frac{1}{x} + \frac{1}{y} + \frac{1}{z} ⩽ \frac{3}{z} \Rightarrow z ⩽ 4

i) For z = 4 substituting into (1) we get

\frac{2}{y} ⩾ \frac{1}{x} + \frac{1}{y} = \frac{1}{2} (2) \Rightarrow y ⩽ 4 \Rightarrow y \in {1, 2, 3, 4}

∙ For y = 4 substituting into (2) we get

\frac{1}{x} = \frac{1}{2} - \frac{1}{4} = \frac{1}{4} \Rightarrow x = 4

∙ For y = 3 substituting into (2) we get

\frac{1}{x} = \frac{1}{2} - \frac{1}{3} = \frac{1}{6} \Rightarrow x = 6

∙ For y \in {1, 2} substituting into (2) we

get L . H . S (2) > \frac{1}{2} \Rightarrow has no roots

ii) For z = 3 substituting into (1) we get

\frac{2}{y} ⩾ \frac{1}{x} + \frac{1}{y} = \frac{3}{4} - \frac{1}{3} = \frac{5}{12} (3) \Rightarrow 5 y ⩽ 24 .

Since y \in N \Rightarrow y \in {1, 2, 3, 4}

∙ For y = 4 substituting into (3) we get

\frac{1}{x} = \frac{5}{12} - \frac{1}{4} = \frac{2}{12} = \frac{1}{6} \Rightarrow x = 6

∙ For y = 3 substituting into (3) we get

\frac{1}{x} = \frac{5}{12} - \frac{1}{3} = \frac{1}{12} \Rightarrow x = 12

∙ For y \in {1, 2} substituting into (3) we

get L . H . S (3) > \frac{5}{12} \Rightarrow has no roots

iii) For z = 2 substituting into (1) we get

\frac{2}{y} ⩾ \frac{1}{x} + \frac{1}{y} = \frac{3}{4} - \frac{1}{2} = \frac{1}{4} (4) \Rightarrow y ⩽ 8

∙ For y = 8 substituting into (4) we get

\frac{1}{x} = \frac{1}{4} - \frac{1}{8} = \frac{1}{8} \Rightarrow x = 8

∙ For y = 7 substituting into (4) we get

\frac{1}{x} = \frac{1}{4} - \frac{1}{7} = \frac{3}{28} \Rightarrow 3 x = 28 \Rightarrow x \notin N

∙ For y = 6 substituting into (4) we get

\frac{1}{x} = \frac{1}{4} - \frac{1}{6} = \frac{1}{12} \Rightarrow x = 12

∙ For y = 5 substituting into (4) we get

\frac{1}{x} = \frac{1}{4} - \frac{1}{5} = \frac{1}{20} \Rightarrow x = 20

∙ For y \in {1, 2, 3, 4} substituting into (4)

we get L . H . S (4) > \frac{1}{4} \Rightarrow has no roots

Combining three above cases we obtain

6 roots are :

(x, y, z) \in {(4, 4, 4), (6, 4, 3), (12, 3, 3)

(8, 8, 2), (12, 6, 2), (20, 5, 2)} (*)

Consequently, the equation

\frac{1}{x} + \frac{1}{y} + \frac{1}{z} = \frac{3}{4} has 3.3! + 2 . \frac{3!}{2} + 1 = 25

roots (x, y, z) . They are elements of (*)

and all their permutation

Commented by mr W last updated on 17/Oct/20

t h a n k y o u a l l s i r s!

Facebook Tweet Pin