Question Number 171837 by Mikenice last updated on 21/Jun/22

$${solve}: \\ $$$$\mathrm{2}{x}+\mathrm{3}{y}=\mathrm{2} \\ $$$${x}+{y}=\mathrm{5}{xy} \\ $$
Answered by Rasheed.Sindhi last updated on 21/Jun/22
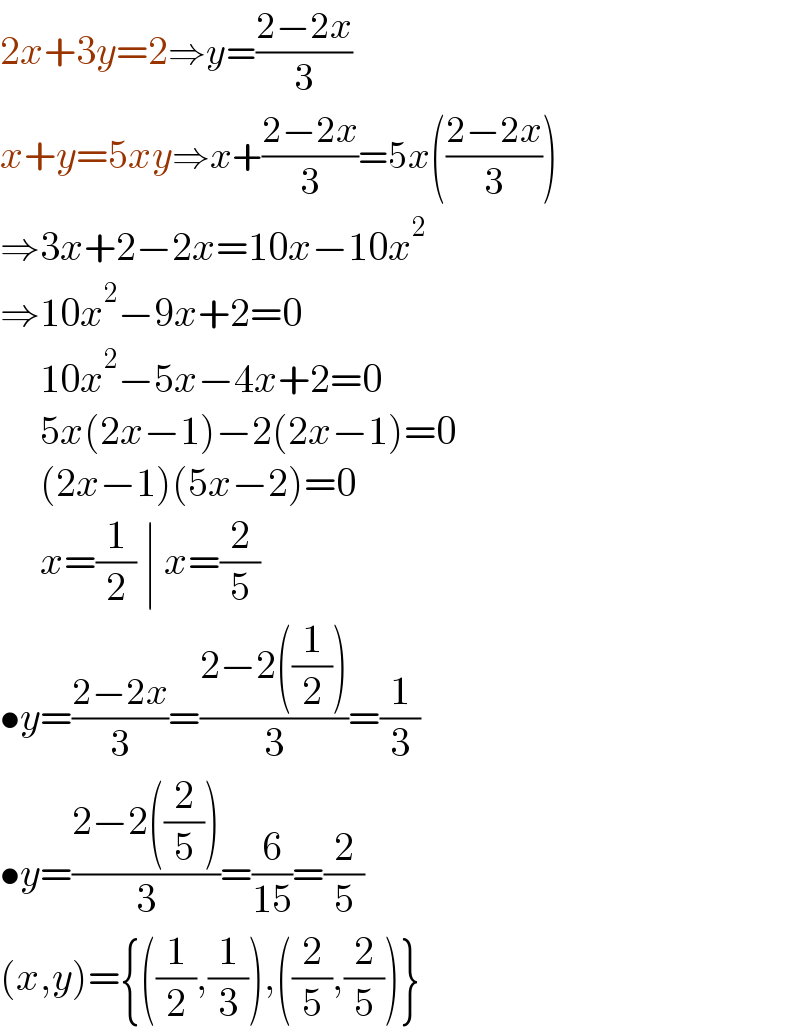
$$\mathrm{2}{x}+\mathrm{3}{y}=\mathrm{2}\Rightarrow{y}=\frac{\mathrm{2}−\mathrm{2}{x}}{\mathrm{3}} \\ $$$${x}+{y}=\mathrm{5}{xy}\Rightarrow{x}+\frac{\mathrm{2}−\mathrm{2}{x}}{\mathrm{3}}=\mathrm{5}{x}\left(\frac{\mathrm{2}−\mathrm{2}{x}}{\mathrm{3}}\right) \\ $$$$\Rightarrow\mathrm{3}{x}+\mathrm{2}−\mathrm{2}{x}=\mathrm{10}{x}−\mathrm{10}{x}^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{10}{x}^{\mathrm{2}} −\mathrm{9}{x}+\mathrm{2}=\mathrm{0} \\ $$$$\:\:\:\:\:\mathrm{10}{x}^{\mathrm{2}} −\mathrm{5}{x}−\mathrm{4}{x}+\mathrm{2}=\mathrm{0} \\ $$$$\:\:\:\:\:\mathrm{5}{x}\left(\mathrm{2}{x}−\mathrm{1}\right)−\mathrm{2}\left(\mathrm{2}{x}−\mathrm{1}\right)=\mathrm{0} \\ $$$$\:\:\:\:\:\left(\mathrm{2}{x}−\mathrm{1}\right)\left(\mathrm{5}{x}−\mathrm{2}\right)=\mathrm{0} \\ $$$$\:\:\:\:\:{x}=\frac{\mathrm{1}}{\mathrm{2}}\:\mid\:{x}=\frac{\mathrm{2}}{\mathrm{5}} \\ $$$$\bullet{y}=\frac{\mathrm{2}−\mathrm{2}{x}}{\mathrm{3}}=\frac{\mathrm{2}−\mathrm{2}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)}{\mathrm{3}}=\frac{\mathrm{1}}{\mathrm{3}} \\ $$$$\bullet{y}=\frac{\mathrm{2}−\mathrm{2}\left(\frac{\mathrm{2}}{\mathrm{5}}\right)}{\mathrm{3}}=\frac{\mathrm{6}}{\mathrm{15}}=\frac{\mathrm{2}}{\mathrm{5}} \\ $$$$\left({x},{y}\right)=\left\{\left(\frac{\mathrm{1}}{\mathrm{2}},\frac{\mathrm{1}}{\mathrm{3}}\right),\left(\frac{\mathrm{2}}{\mathrm{5}},\frac{\mathrm{2}}{\mathrm{5}}\right)\right\} \\ $$
