Question Number 152626 by mathdanisur last updated on 30/Aug/21

$$\mathrm{Solve}\:\mathrm{for}\:\mathrm{real}\:\mathrm{numbers}: \\ $$$$\frac{\mathrm{1}}{\mathrm{x}-\mathrm{1}}\:+\:\frac{\mathrm{2}}{\mathrm{x}-\mathrm{2}}\:+\:\frac{\mathrm{3}}{\mathrm{x}-\mathrm{3}}\:+\:\frac{\mathrm{4}}{\mathrm{x}-\mathrm{4}}\:=\:\mathrm{2x}^{\mathrm{2}} -\mathrm{5x}-\mathrm{4} \\ $$
Answered by MJS_new last updated on 30/Aug/21

$${x}=\mathrm{0} \\ $$$${x}=\frac{\mathrm{5}}{\mathrm{2}} \\ $$$${x}=\mathrm{2}\pm\sqrt{\mathrm{2}} \\ $$$${x}=\mathrm{3}\pm\sqrt{\mathrm{2}} \\ $$
Commented by MJS_new last updated on 30/Aug/21
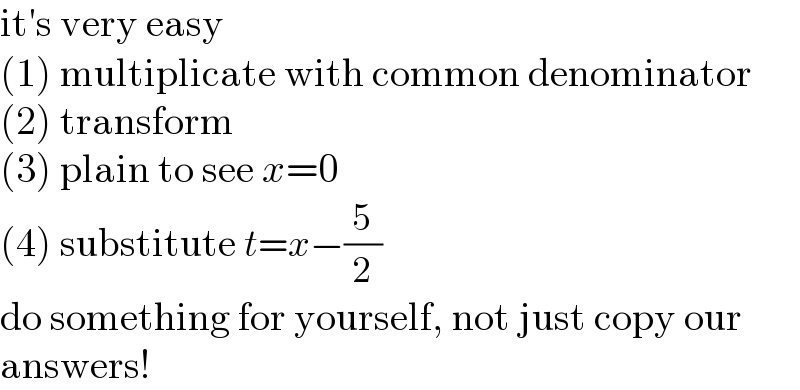
$$\mathrm{it}'\mathrm{s}\:\mathrm{very}\:\mathrm{easy} \\ $$$$\left(\mathrm{1}\right)\:\mathrm{multiplicate}\:\mathrm{with}\:\mathrm{common}\:\mathrm{denominator} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{transform} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{plain}\:\mathrm{to}\:\mathrm{see}\:{x}=\mathrm{0} \\ $$$$\left(\mathrm{4}\right)\:\mathrm{substitute}\:{t}={x}−\frac{\mathrm{5}}{\mathrm{2}} \\ $$$$\mathrm{do}\:\mathrm{something}\:\mathrm{for}\:\mathrm{yourself},\:\mathrm{not}\:\mathrm{just}\:\mathrm{copy}\:\mathrm{our} \\ $$$$\mathrm{answers}! \\ $$
