Solve-for-x-10-4-x-x-4-10-8-

Question Number 24443 by Tinkutara last updated on 18/Nov/17

S o l v e f o r x :

{(10^{- 4} x)}^{x} = 4 \times 10^{- 8}

Answered by ajfour last updated on 18/Nov/17

\Rightarrow x \ln (10^{- 4} x) = \ln 4 - 8

o r \ln x - 4 = \frac{\ln 4}{x} - \frac{8}{x}

\Rightarrow \frac{1}{2} (2 \ln x - 8) = \frac{1}{x} (2 \ln 2 - 8)

\Rightarrow x = 2 (a t l e a s t) .

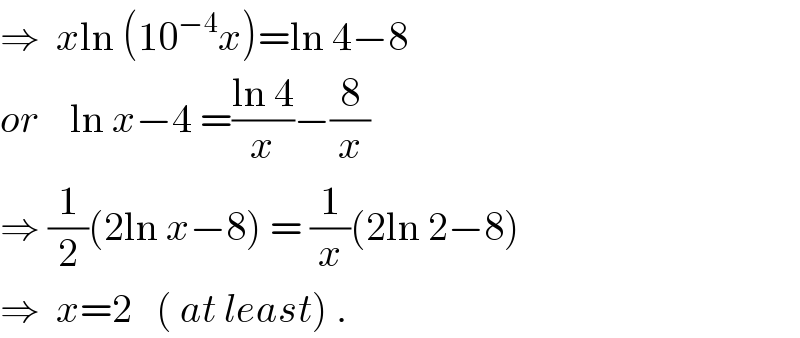
Commented by Tinkutara last updated on 19/Nov/17

Thank you very much Sir!

Answered by mrW1 last updated on 19/Nov/17

T o s i m p l y t h e w r i t i n g, {l e t}^{'} s s a y

t h e e q u a t i o n i s {(a x)}^{x} = b .

[\ln (a x)] x = \ln b

[\ln (a x)] (a x) = a \ln b

[\ln (a x)] e^{\ln (a x)} = a \ln b

\Rightarrow \ln (a x) = W (a \ln b)

\Rightarrow a x = e^{W (a \ln b)} = \frac{a \ln b}{W (a \ln b)}

\Rightarrow x = \frac{\ln b}{W (a \ln b)}

w i t h a = 10^{- 4} a n d b = 4 \times 10^{- 8}

\Rightarrow x = \frac{\ln 4 - 8 \ln 10}{W [10^{- 4} (\ln 4 - 8 \ln 10)]}

= \frac{- 17.0344}{W [- 1.70344 \times 10^{- 3}]} = {\begin{cases} \frac{- 17.0344}{- 8.5172} = 2 \\ \frac{- 17.0344}{- 0.0017064} = 9983 \end{cases}

i . e . t h e r e a r e t w o s o l u t i o n s :

x = 2 a n d 9983 .

Commented by Tinkutara last updated on 19/Nov/17

A n d h o w t o f i n d W [- 1.703 \times 10^{- 5}]

u s i n g G e o g e b r a ?

Commented by mrW1 last updated on 19/Nov/17

I f y o u {d o n}^{'} t h a v e a c a l c u l a t o r f o r

W - f u n c t i o n, y o u c a n c a l c u l a t e t h e

v a l u e (s) o f W (p) u s i n g G e o g e b r a .

T h e v a l u e (s) o f W (p) i s (a r e) t h e

r o o t (s) o f e q u a t i o n

f (x) = {x e}^{x} - p = 0

F o r p < 0 t h e r e a r e u s u a l l y t w o r o o t s .

Commented by mrW1 last updated on 19/Nov/17

Commented by mrW1 last updated on 19/Nov/17

T h e e x a m p l e a b o v e s h o w s

W (- 0.2) = - 2.54264 o r - 0.25917

Commented by Tinkutara last updated on 19/Nov/17

Thank you very much Sir!