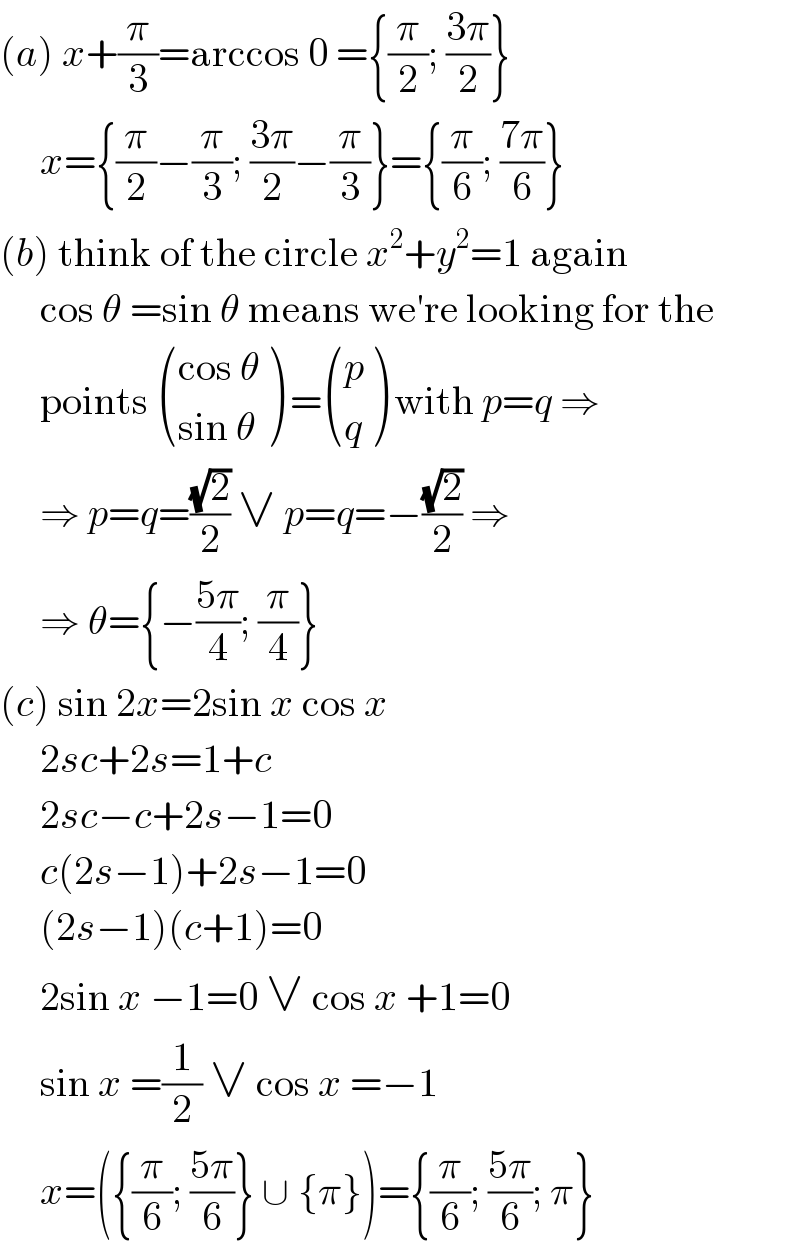Question Number 39587 by Rio Mike last updated on 08/Jul/18
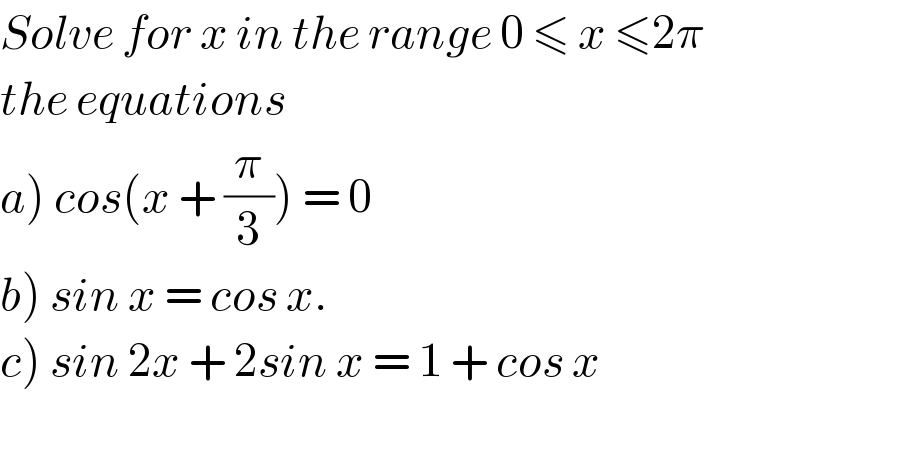
Answered by Joel579 last updated on 08/Jul/18

Answered by Joel579 last updated on 08/Jul/18

Answered by Joel579 last updated on 08/Jul/18

Answered by MJS last updated on 08/Jul/18