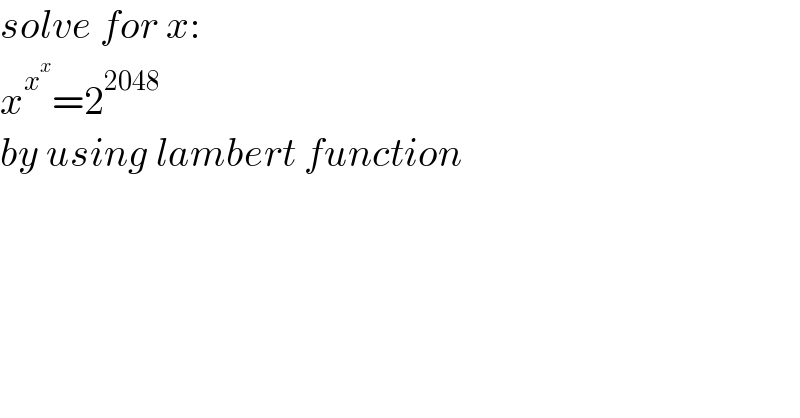
solve-for-x-x-x-x-2-2048-by-using-lambert-function-

Question Number 183769 by Michaelfaraday last updated on 29/Dec/22

s o l v e f o r x :

x^{x^{x}} = 2^{2048}

b y u s i n g l a m b e r t f u n c t i o n

Commented by mr W last updated on 30/Dec/22

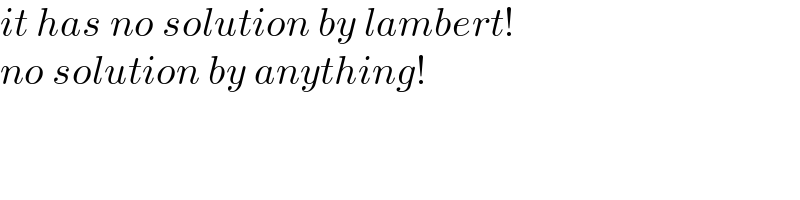
i t h a s n o s o l u t i o n b y l a m b e r t!

n o s o l u t i o n b y a n y t h i n g!

Commented by mr W last updated on 30/Dec/22

4^{4^{4}} = 2^{512} < 2^{2048}

5^{5^{5}} = 5^{3125} > 2^{2048}

\Rightarrow t h e r o o t o f x^{x^{x}} = 2^{2048} l i e s b e t w e e n

4 a n d 5 .

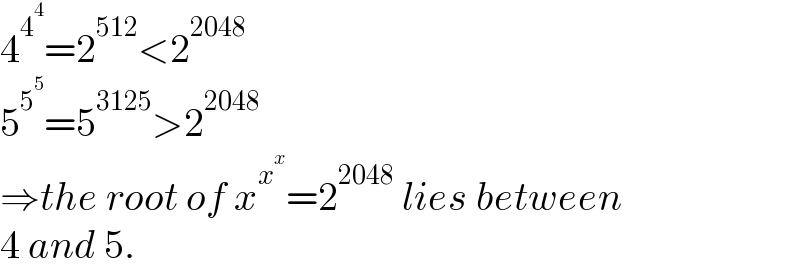
Commented by Michaelfaraday last updated on 30/Dec/22

o k a y t h a n k s s i r

Commented by paul2222 last updated on 03/Jan/23

we can use newton raphson iteration