Solve-in-R-59-x-2-1-3-12-x-11-1-3-6-

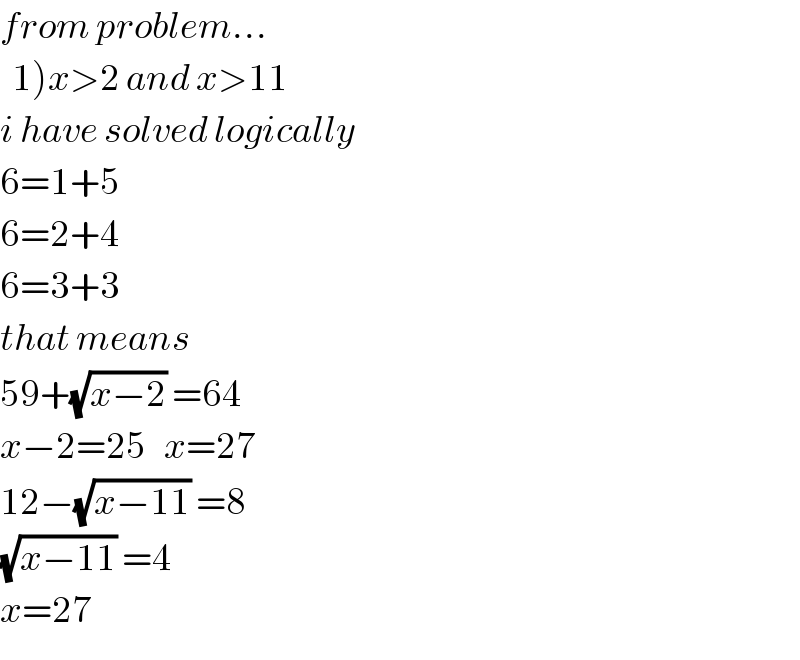
Question Number 47993 by F_Nongue last updated on 18/Nov/18

S o l v e i n R

\sqrt[3]{59 + \sqrt{x - 2}} + \sqrt[3]{12 - \sqrt{x - 11}} = 6

Commented by tanmay.chaudhury50@gmail.com last updated on 18/Nov/18

x = 27

Commented by ajfour last updated on 18/Nov/18

s t e p s p l e a s e . .

Commented by MJS last updated on 18/Nov/18

we can try

or

we can eliminate all roots which leads to

a polynome of 5^{th} degree \Rightarrow we can only try

again . is there an easier way ?

[putting x = t + \frac{13}{2} helps while transforming]

Commented by MJS last updated on 18/Nov/18

trying to find x with both x - 2 and x - 11

are square numbers we get x = 11 or x = 27

but only x = 27 fits

f (x) = \sqrt[3]{59 + \sqrt{x - 2}} + \sqrt[3]{12 - \sqrt{x - 11}} - 6 is decreasing

for x ⩾ 11 \Rightarrow {there}^{'} s only 1 solution

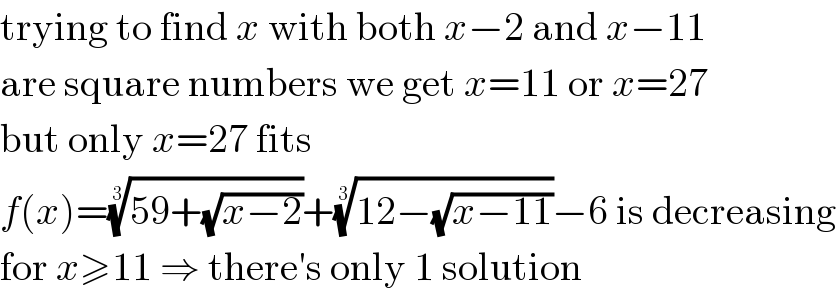
Commented by tanmay.chaudhury50@gmail.com last updated on 18/Nov/18

f r o m p r o b l e m \dots

1) x > 2 a n d x > 11

i h a v e s o l v e d l o g i c a l l y

6 = 1 + 5

6 = 2 + 4

6 = 3 + 3

t h a t m e a n s

59 + \sqrt{x - 2} = 64

x - 2 = 25 x = 27

12 - \sqrt{x - 11} = 8

\sqrt{x - 11} = 4

x = 27