Question Number 15840 by tawa tawa last updated on 14/Jun/17

$$\mathrm{Solve}\:\mathrm{the}\:\:\mathrm{ODE} \\ $$$$\frac{\mathrm{dy}}{\mathrm{dx}}\:+\:\mathrm{x}^{\mathrm{2}} \mathrm{y}\:=\:\mathrm{x}^{\mathrm{4}} \\ $$$$ \\ $$
Commented by chux last updated on 14/Jun/17
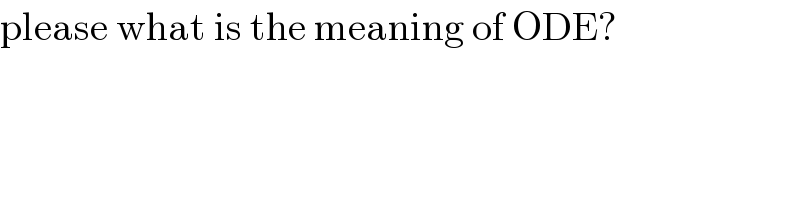
$$\mathrm{please}\:\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{meaning}\:\mathrm{of}\:\mathrm{ODE}? \\ $$
Commented by tawa tawa last updated on 14/Jun/17

$$\mathrm{Ordinary}\:\mathrm{differential}\:\mathrm{equation} \\ $$
Answered by mrW1 last updated on 15/Jun/17
![(dy/dx) + x^2 y = x^4 (dy/dx) + p(x)y =q(x) p(x)=x^2 q(x)=x^4 P(x)=∫p(x)dx=∫x^2 dx=(x^3 /3) let y=c(x)e^(−P(x)) (dy/dx)=c′(x)e^(−P(x)) −p(x)c(x)e^(−P(x)) ⇒c′(x)e^(−P(x)) −p(x)c(x)e^(−P(x)) +p(x)c(x)e^(−P(x)) =q(x) ⇒c′(x)e^(−P(x)) =q(x) ⇒c′(x)=q(x)e^(P(x)) =x^4 e^(x^3 /3) ⇒c(x)=∫x^4 e^(x^3 /3) dx=3^(2/3) Γ((5/3),−(x^3 /3))+C y=c(x)e^(−P(x)) ⇒y=3^(2/3) e^(−(x^3 /3)) [Γ((5/3),−(x^3 /3))+C] I don′t know if this is really the expected result.](https://www.tinkutara.com/question/Q15920.png)
$$\frac{\mathrm{dy}}{\mathrm{dx}}\:+\:\mathrm{x}^{\mathrm{2}} \mathrm{y}\:=\:\mathrm{x}^{\mathrm{4}} \\ $$$$\frac{\mathrm{dy}}{\mathrm{dx}}\:+\:\mathrm{p}\left(\mathrm{x}\right)\mathrm{y}\:=\mathrm{q}\left(\mathrm{x}\right) \\ $$$$ \\ $$$$\mathrm{p}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{2}} \\ $$$$\mathrm{q}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{4}} \\ $$$$ \\ $$$$\mathrm{P}\left(\mathrm{x}\right)=\int\mathrm{p}\left(\mathrm{x}\right)\mathrm{dx}=\int\mathrm{x}^{\mathrm{2}} \mathrm{dx}=\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}} \\ $$$$ \\ $$$$\mathrm{let}\:\mathrm{y}=\mathrm{c}\left(\mathrm{x}\right)\mathrm{e}^{−\mathrm{P}\left(\mathrm{x}\right)} \\ $$$$\frac{\mathrm{dy}}{\mathrm{dx}}=\mathrm{c}'\left(\mathrm{x}\right)\mathrm{e}^{−\mathrm{P}\left(\mathrm{x}\right)} −\mathrm{p}\left(\mathrm{x}\right)\mathrm{c}\left(\mathrm{x}\right)\mathrm{e}^{−\mathrm{P}\left(\mathrm{x}\right)} \\ $$$$\Rightarrow\mathrm{c}'\left(\mathrm{x}\right)\mathrm{e}^{−\mathrm{P}\left(\mathrm{x}\right)} −\mathrm{p}\left(\mathrm{x}\right)\mathrm{c}\left(\mathrm{x}\right)\mathrm{e}^{−\mathrm{P}\left(\mathrm{x}\right)} +\mathrm{p}\left(\mathrm{x}\right)\mathrm{c}\left(\mathrm{x}\right)\mathrm{e}^{−\mathrm{P}\left(\mathrm{x}\right)} =\mathrm{q}\left(\mathrm{x}\right) \\ $$$$\Rightarrow\mathrm{c}'\left(\mathrm{x}\right)\mathrm{e}^{−\mathrm{P}\left(\mathrm{x}\right)} =\mathrm{q}\left(\mathrm{x}\right) \\ $$$$\Rightarrow\mathrm{c}'\left(\mathrm{x}\right)=\mathrm{q}\left(\mathrm{x}\right)\mathrm{e}^{\mathrm{P}\left(\mathrm{x}\right)} =\mathrm{x}^{\mathrm{4}} \mathrm{e}^{\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}}} \\ $$$$\Rightarrow\mathrm{c}\left(\mathrm{x}\right)=\int\mathrm{x}^{\mathrm{4}} \mathrm{e}^{\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}}} \mathrm{dx}=\mathrm{3}^{\frac{\mathrm{2}}{\mathrm{3}}} \Gamma\left(\frac{\mathrm{5}}{\mathrm{3}},−\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}}\right)+\mathrm{C} \\ $$$$\mathrm{y}=\mathrm{c}\left(\mathrm{x}\right)\mathrm{e}^{−\mathrm{P}\left(\mathrm{x}\right)} \\ $$$$\Rightarrow\mathrm{y}=\mathrm{3}^{\frac{\mathrm{2}}{\mathrm{3}}} \mathrm{e}^{−\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}}} \left[\Gamma\left(\frac{\mathrm{5}}{\mathrm{3}},−\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}}\right)+\mathrm{C}\right] \\ $$$$ \\ $$$$\mathrm{I}\:\mathrm{don}'\mathrm{t}\:\mathrm{know}\:\mathrm{if}\:\mathrm{this}\:\mathrm{is}\:\mathrm{really}\:\mathrm{the}\:\mathrm{expected} \\ $$$$\mathrm{result}. \\ $$
Commented by tawa tawa last updated on 15/Jun/17

$$\mathrm{I}\:\mathrm{will}\:\mathrm{check}\:\mathrm{sir}.\:\mathrm{Thanks}\:\mathrm{for}\:\mathrm{your}\:\mathrm{help}.\:\mathrm{i}\:\mathrm{really}\:\mathrm{appreciate}\:\mathrm{sir}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you} \\ $$
