Menu Close

Solve-the-system-x-3-x-2-y-4xy-2-4y-3-0-x-2-2xy-3y-2-x-y-0-

Tinku Tara 0 Comments
Pin




Question Number 48055 by F_Nongue last updated on 21/Nov/18
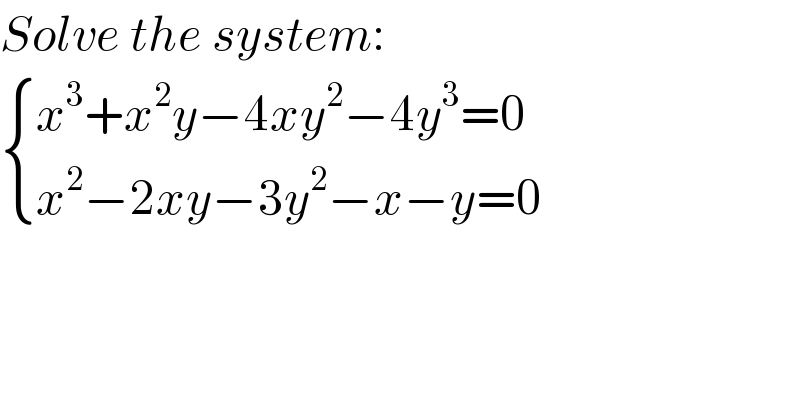
Solve the system: { ((x^3 +x^2 y−4xy^2 −4y^3 =0)),((x^2 −2xy−3y^2 −x−y=0)) :}
$${Solve}\:{the}\:{system}: \\ $$$$\begin{cases}{{x}^{\mathrm{3}} +{x}^{\mathrm{2}} {y}−\mathrm{4}{xy}^{\mathrm{2}} −\mathrm{4}{y}^{\mathrm{3}} =\mathrm{0}}\\{{x}^{\mathrm{2}} −\mathrm{2}{xy}−\mathrm{3}{y}^{\mathrm{2}} −{x}−{y}=\mathrm{0}}\end{cases} \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 19/Nov/18
x=0 y=0
$${x}=\mathrm{0}\:\:\:{y}=\mathrm{0}\: \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 19/Nov/18
Commented by tanmay.chaudhury50@gmail.com last updated on 19/Nov/18
(x,y)=(−0.869,−0.171) (x,y)=(1,−1) (x,y)=(2.628,1.459) obtained from graph...
$$\left({x},{y}\right)=\left(−\mathrm{0}.\mathrm{869},−\mathrm{0}.\mathrm{171}\right) \\ $$$$\left({x},{y}\right)=\left(\mathrm{1},−\mathrm{1}\right) \\ $$$$\left({x},{y}\right)=\left(\mathrm{2}.\mathrm{628},\mathrm{1}.\mathrm{459}\right)\:\:{obtained}\:{from}\:{graph}… \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 19/Nov/18
Commented by F_Nongue last updated on 19/Nov/18
excuse me people, it was missing some power. Now it is correct
Commented by F_Nongue last updated on 19/Nov/18
I get x= 1/4 , y=-1/4
Answered by ajfour last updated on 21/Nov/18
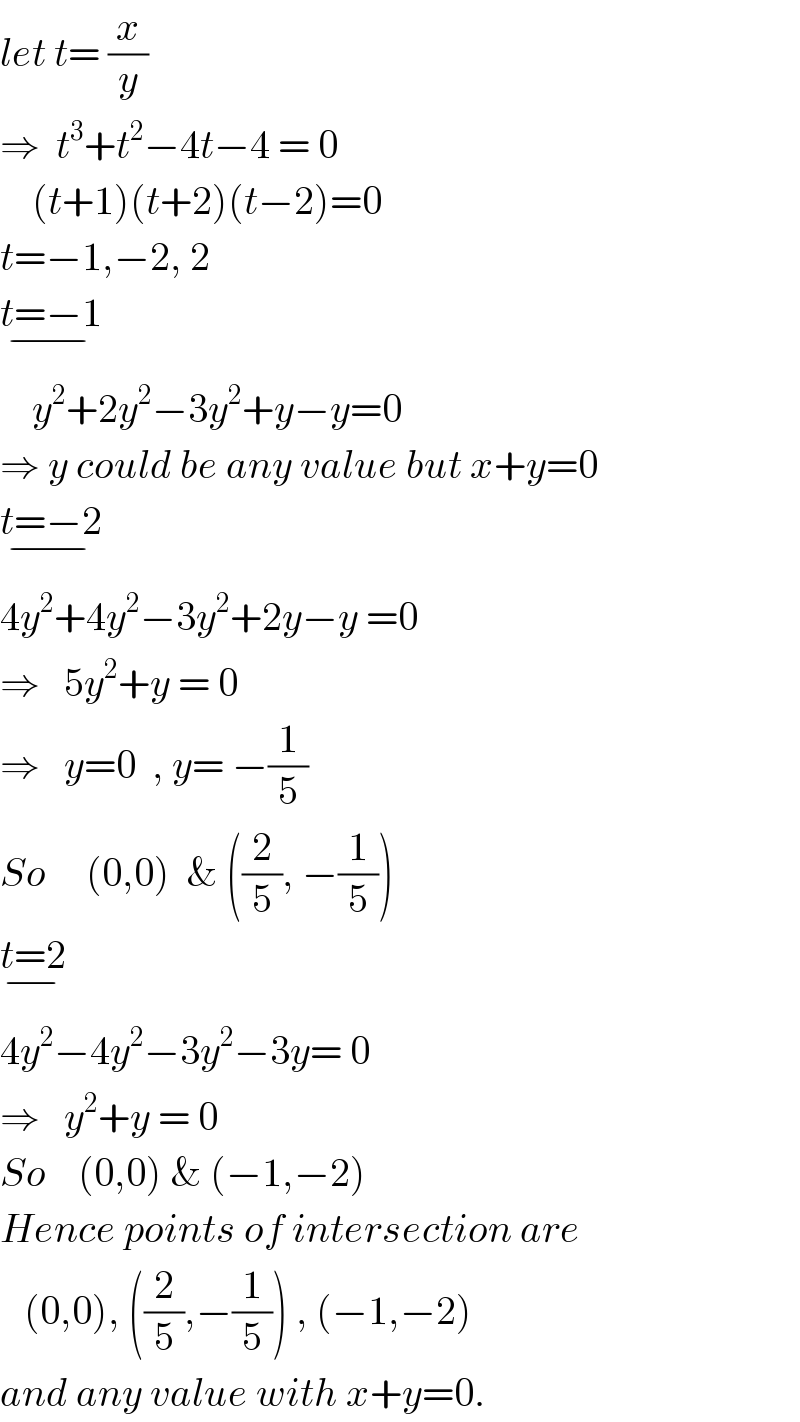
let t= (x/y) ⇒ t^3 +t^2 −4t−4 = 0 (t+1)(t+2)(t−2)=0 t=−1,−2, 2 t=−1_(−) y^2 +2y^2 −3y^2 +y−y=0 ⇒ y could be any value but x+y=0 t=−2_(−) 4y^2 +4y^2 −3y^2 +2y−y =0 ⇒ 5y^2 +y = 0 ⇒ y=0 , y= −(1/5) So (0,0) & ((2/5), −(1/5)) t=2_(−) 4y^2 −4y^2 −3y^2 −3y= 0 ⇒ y^2 +y = 0 So (0,0) & (−1,−2) Hence points of intersection are (0,0), ((2/5),−(1/5)) , (−1,−2) and any value with x+y=0.
$${let}\:{t}=\:\frac{{x}}{{y}} \\ $$$$\Rightarrow\:\:{t}^{\mathrm{3}} +{t}^{\mathrm{2}} −\mathrm{4}{t}−\mathrm{4}\:=\:\mathrm{0} \\ $$$$\:\:\:\:\left({t}+\mathrm{1}\right)\left({t}+\mathrm{2}\right)\left({t}−\mathrm{2}\right)=\mathrm{0} \\ $$$${t}=−\mathrm{1},−\mathrm{2},\:\mathrm{2} \\ $$$$\underset{−} {{t}=−\mathrm{1}} \\ $$$$\:\:\:\:{y}^{\mathrm{2}} +\mathrm{2}{y}^{\mathrm{2}} −\mathrm{3}{y}^{\mathrm{2}} +{y}−{y}=\mathrm{0} \\ $$$$\Rightarrow\:{y}\:{could}\:{be}\:{any}\:{value}\:{but}\:{x}+{y}=\mathrm{0} \\ $$$$\underset{−} {{t}=−\mathrm{2}} \\ $$$$\mathrm{4}{y}^{\mathrm{2}} +\mathrm{4}{y}^{\mathrm{2}} −\mathrm{3}{y}^{\mathrm{2}} +\mathrm{2}{y}−{y}\:=\mathrm{0} \\ $$$$\Rightarrow\:\:\:\mathrm{5}{y}^{\mathrm{2}} +{y}\:=\:\mathrm{0} \\ $$$$\Rightarrow\:\:\:{y}=\mathrm{0}\:\:,\:{y}=\:−\frac{\mathrm{1}}{\mathrm{5}} \\ $$$${So}\:\:\:\:\:\left(\mathrm{0},\mathrm{0}\right)\:\:\&\:\left(\frac{\mathrm{2}}{\mathrm{5}},\:−\frac{\mathrm{1}}{\mathrm{5}}\right) \\ $$$$\underset{−} {{t}=\mathrm{2}} \\ $$$$\mathrm{4}{y}^{\mathrm{2}} −\mathrm{4}{y}^{\mathrm{2}} −\mathrm{3}{y}^{\mathrm{2}} −\mathrm{3}{y}=\:\mathrm{0} \\ $$$$\Rightarrow\:\:\:{y}^{\mathrm{2}} +{y}\:=\:\mathrm{0} \\ $$$${So}\:\:\:\:\left(\mathrm{0},\mathrm{0}\right)\:\&\:\left(−\mathrm{1},−\mathrm{2}\right) \\ $$$${Hence}\:{points}\:{of}\:{intersection}\:{are} \\ $$$$\:\:\:\left(\mathrm{0},\mathrm{0}\right),\:\left(\frac{\mathrm{2}}{\mathrm{5}},−\frac{\mathrm{1}}{\mathrm{5}}\right)\:,\:\left(−\mathrm{1},−\mathrm{2}\right) \\ $$$${and}\:{any}\:{value}\:{with}\:{x}+{y}=\mathrm{0}. \\ $$
Answered by Riya Chauhan last updated on 21/Nov/18

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *