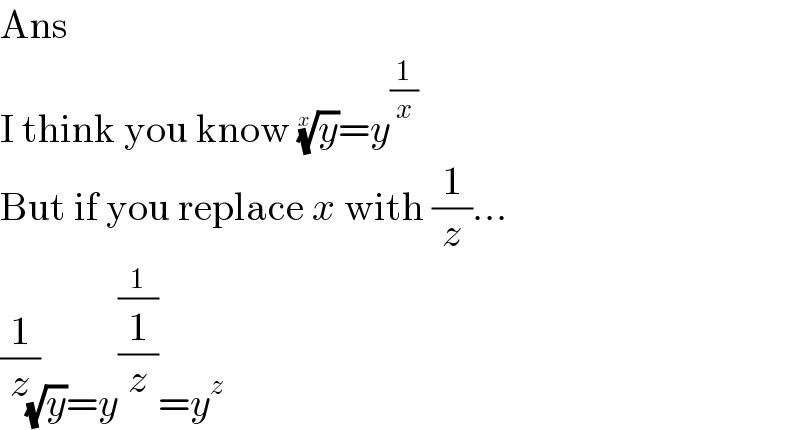Question Number 156709 by aliyn last updated on 14/Oct/21
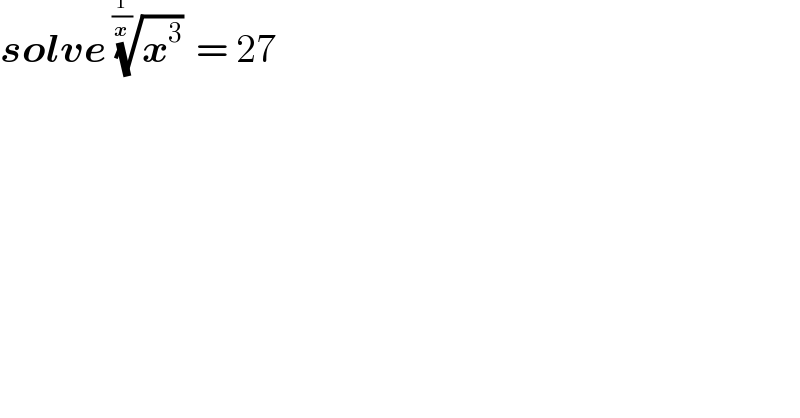
Answered by mr W last updated on 14/Oct/21
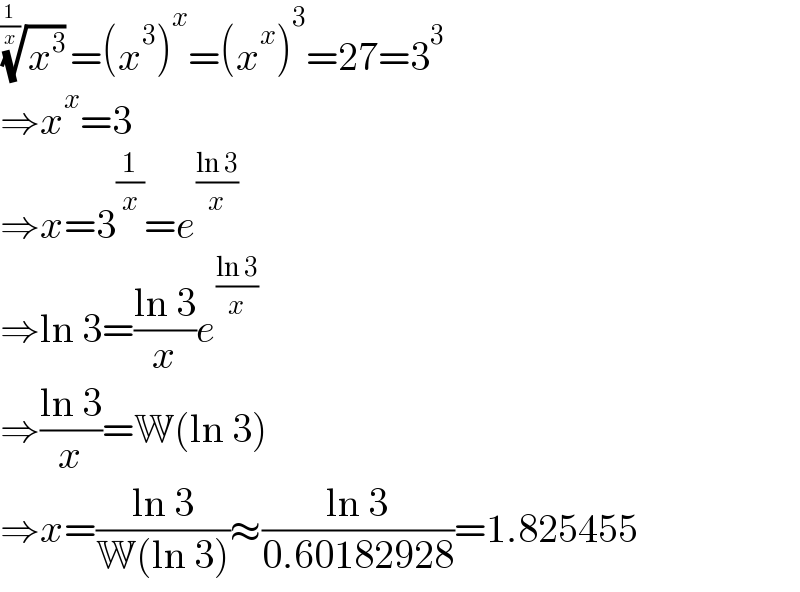
Commented by tabata last updated on 14/Oct/21
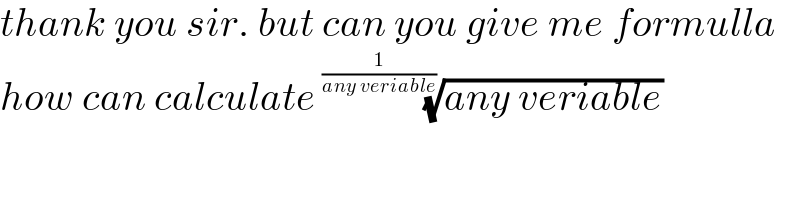
Commented by mr W last updated on 14/Oct/21
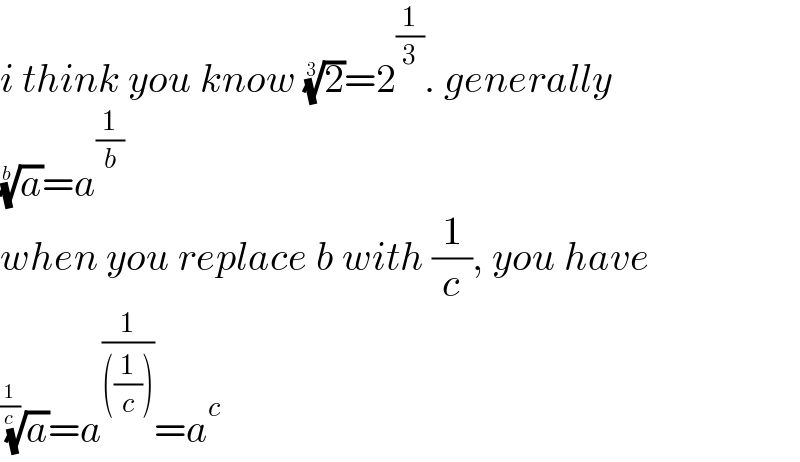
Commented by tabata last updated on 14/Oct/21

Commented by mr W last updated on 15/Oct/21

Commented by Sky_b last updated on 15/Oct/21
Commented by Ruuudiy last updated on 16/Oct/21