solve-y-2xy-xe-x-2-

Question Number 102163 by mathmax by abdo last updated on 07/Jul/20

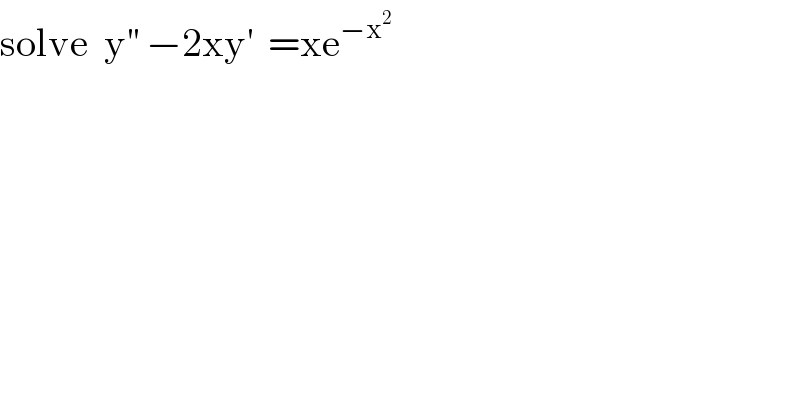
$$\mathrm{solve}\:\:\mathrm{y}^{''} \:−\mathrm{2xy}^{'} \:\:=\mathrm{xe}^{−\mathrm{x}^{\mathrm{2}} } \\ $$

Answered by mathmax by abdo last updated on 08/Jul/20

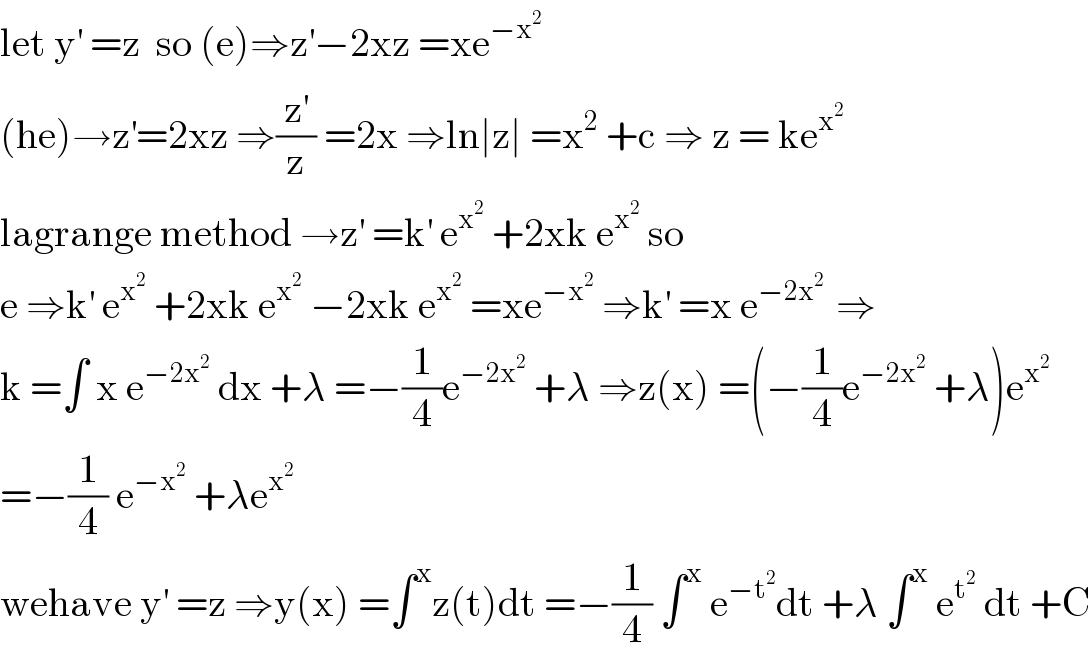
let y^′ =z so (e)⇒z^′ −2xz =xe^(−x^2 ) (he)→z^′ =2xz ⇒(z^′ /z) =2x ⇒ln∣z∣ =x^2 +c ⇒ z = ke^x^2 lagrange method →z^′ =k^′ e^x^2 +2xk e^x^2 so e ⇒k^′ e^x^2 +2xk e^x^2 −2xk e^x^2 =xe^(−x^2 ) ⇒k^′ =x e^(−2x^2 ) ⇒ k =∫ x e^(−2x^2 ) dx +λ =−(1/4)e^(−2x^2 ) +λ ⇒z(x) =(−(1/4)e^(−2x^2 ) +λ)e^x^2 =−(1/4) e^(−x^2 ) +λe^x^2 wehave y^′ =z ⇒y(x) =∫^x z(t)dt =−(1/4) ∫^x e^(−t^2 ) dt +λ ∫^x e^t^2 dt +C

$$\mathrm{let}\:\mathrm{y}^{'} \:=\mathrm{z}\:\:\mathrm{so}\:\left(\mathrm{e}\right)\Rightarrow\mathrm{z}^{'} −\mathrm{2xz}\:=\mathrm{xe}^{−\mathrm{x}^{\mathrm{2}} } \\ $$$$\left(\mathrm{he}\right)\rightarrow\mathrm{z}^{'} =\mathrm{2xz}\:\Rightarrow\frac{\mathrm{z}^{'} }{\mathrm{z}}\:=\mathrm{2x}\:\Rightarrow\mathrm{ln}\mid\mathrm{z}\mid\:=\mathrm{x}^{\mathrm{2}} \:+\mathrm{c}\:\Rightarrow\:\mathrm{z}\:=\:\mathrm{ke}^{\mathrm{x}^{\mathrm{2}} } \\ $$$$\mathrm{lagrange}\:\mathrm{method}\:\rightarrow\mathrm{z}^{'} \:=\mathrm{k}^{'} \:\mathrm{e}^{\mathrm{x}^{\mathrm{2}} } \:+\mathrm{2xk}\:\mathrm{e}^{\mathrm{x}^{\mathrm{2}} } \:\mathrm{so} \\ $$$$\mathrm{e}\:\Rightarrow\mathrm{k}^{'} \:\mathrm{e}^{\mathrm{x}^{\mathrm{2}} } \:+\mathrm{2xk}\:\mathrm{e}^{\mathrm{x}^{\mathrm{2}} } \:−\mathrm{2xk}\:\mathrm{e}^{\mathrm{x}^{\mathrm{2}} } \:=\mathrm{xe}^{−\mathrm{x}^{\mathrm{2}} } \:\Rightarrow\mathrm{k}^{'} \:=\mathrm{x}\:\mathrm{e}^{−\mathrm{2x}^{\mathrm{2}} \:} \:\Rightarrow \\ $$$$\mathrm{k}\:=\int\:\mathrm{x}\:\mathrm{e}^{−\mathrm{2x}^{\mathrm{2}} } \:\mathrm{dx}\:+\lambda\:=−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{e}^{−\mathrm{2x}^{\mathrm{2}} } \:+\lambda\:\Rightarrow\mathrm{z}\left(\mathrm{x}\right)\:=\left(−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{e}^{−\mathrm{2x}^{\mathrm{2}} } \:+\lambda\right)\mathrm{e}^{\mathrm{x}^{\mathrm{2}} } \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}\:\mathrm{e}^{−\mathrm{x}^{\mathrm{2}} } \:+\lambda\mathrm{e}^{\mathrm{x}^{\mathrm{2}} } \\ $$$$\mathrm{wehave}\:\mathrm{y}^{'} \:=\mathrm{z}\:\Rightarrow\mathrm{y}\left(\mathrm{x}\right)\:=\int^{\mathrm{x}} \mathrm{z}\left(\mathrm{t}\right)\mathrm{dt}\:=−\frac{\mathrm{1}}{\mathrm{4}}\:\int^{\mathrm{x}} \:\mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \mathrm{dt}\:+\lambda\:\int^{\mathrm{x}} \:\mathrm{e}^{\mathrm{t}^{\mathrm{2}} } \:\mathrm{dt}\:+\mathrm{C} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply