Square-planar-complex-is-formed-by-hybridisation-of-which-atomic-orbitals-1-s-p-x-p-y-p-z-2-s-p-x-p-y-d-z-2-3-s-p-x-p-y-d-x-2-y-2-4-s-p-x-p-y-d-z-3-

Question Number 23146 by Tinkutara last updated on 26/Oct/17

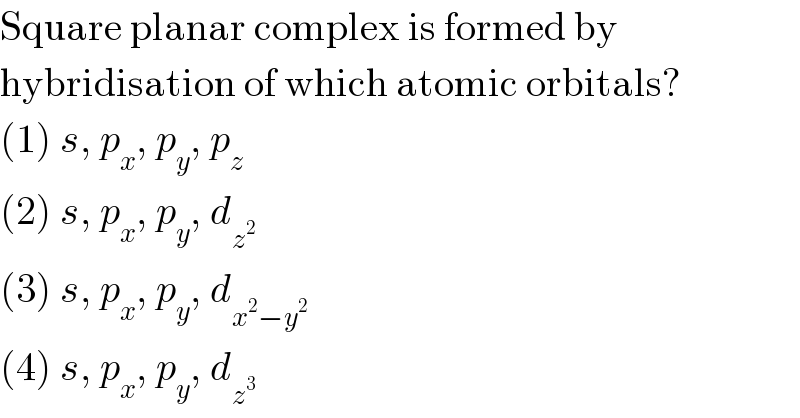
Square planar complex is formed by

hybridisation of which atomic orbitals ?

(1) s, p_{x}, p_{y}, p_{z}

(2) s, p_{x}, p_{y}, d_{z^{2}}

(3) s, p_{x}, p_{y}, d_{x^{2} - y^{2}}

(4) s, p_{x}, p_{y}, d_{z^{3}}

Commented by math solver last updated on 26/Oct/17

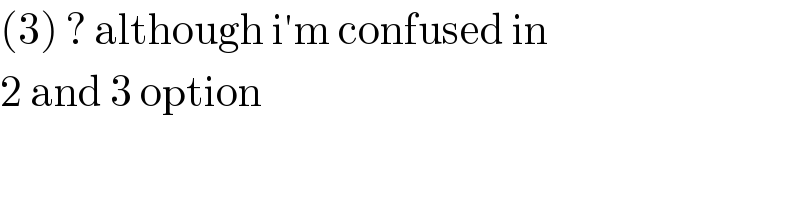
(3) ? although i^{'} m confused in

2 and 3 option

Commented by math solver last updated on 26/Oct/17

actually square planar compex

has hybridisation {dsp}^{2} .

so going by option only 2 and 3

satisfy and d_{z_{}^{3}} is nothing \dots .

Commented by Tinkutara last updated on 26/Oct/17

{Doesn}^{'} t square planar has {s p}^{3} ? This

means 4 σ bonds and 0 lone pairs . And

answer is only (3) .

Commented by math solver last updated on 26/Oct/17

square planar and square planar

complex are different .

square planar has hybridization

{sp}^{3} d^{2} . eg : {XeF}_{4} .

Commented by Tinkutara last updated on 27/Oct/17

{XeF}_{4} has 2 axial lone pairs also . So why

this is square planar ? It should be

bipyramidal .