Question Number 13732 by prakash jain last updated on 22/May/17

$$\mathrm{Sum}\:\mathrm{the}\:\mathrm{following}: \\ $$$$\mathrm{tan}\:{x}+\mathrm{2tan}\:\mathrm{2}{x}+\mathrm{2}^{\mathrm{2}} \mathrm{tan}\:\mathrm{2}^{\mathrm{2}} {x}+…+\mathrm{2}^{{n}} \mathrm{tan}\:\mathrm{2}^{{n}} {x} \\ $$
Commented by ajfour last updated on 23/May/17

$${a}\:{genuine}\:{solution}\:{please}.. \\ $$
Commented by prakash jain last updated on 23/May/17
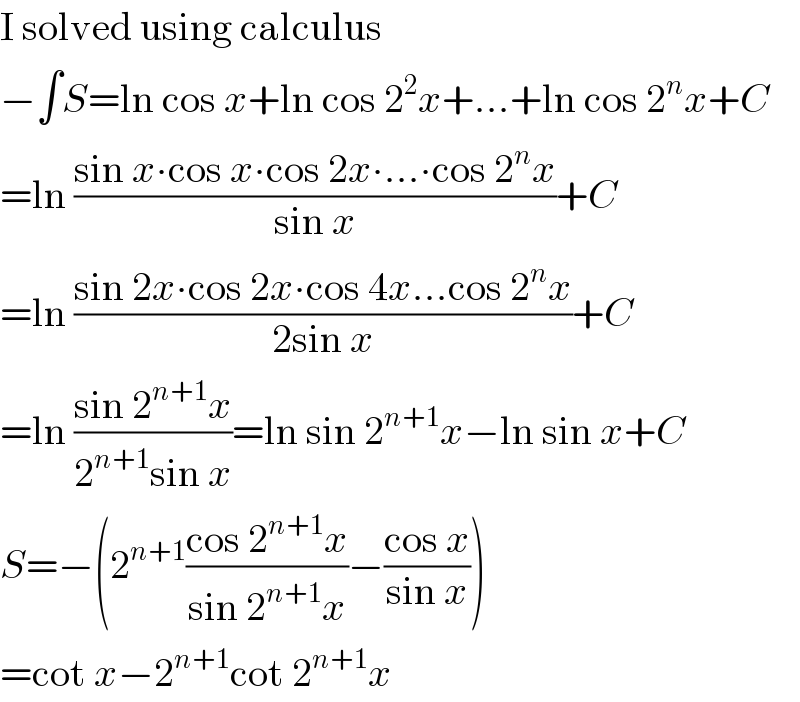
$$\mathrm{I}\:\mathrm{solved}\:\mathrm{using}\:\mathrm{calculus} \\ $$$$−\int{S}=\mathrm{ln}\:\mathrm{cos}\:{x}+\mathrm{ln}\:\mathrm{cos}\:\mathrm{2}^{\mathrm{2}} {x}+…+\mathrm{ln}\:\mathrm{cos}\:\mathrm{2}^{{n}} {x}+{C} \\ $$$$=\mathrm{ln}\:\frac{\mathrm{sin}\:{x}\centerdot\mathrm{cos}\:{x}\centerdot\mathrm{cos}\:\mathrm{2}{x}\centerdot…\centerdot\mathrm{cos}\:\mathrm{2}^{{n}} {x}}{\mathrm{sin}\:{x}}+{C} \\ $$$$=\mathrm{ln}\:\frac{\mathrm{sin}\:\mathrm{2}{x}\centerdot\mathrm{cos}\:\mathrm{2}{x}\centerdot\mathrm{cos}\:\mathrm{4}{x}…\mathrm{cos}\:\mathrm{2}^{{n}} {x}}{\mathrm{2sin}\:{x}}+{C} \\ $$$$=\mathrm{ln}\:\frac{\mathrm{sin}\:\mathrm{2}^{{n}+\mathrm{1}} {x}}{\mathrm{2}^{{n}+\mathrm{1}} \mathrm{sin}\:{x}}=\mathrm{ln}\:\mathrm{sin}\:\mathrm{2}^{{n}+\mathrm{1}} {x}−\mathrm{ln}\:\mathrm{sin}\:{x}+{C} \\ $$$${S}=−\left(\mathrm{2}^{{n}+\mathrm{1}} \frac{\mathrm{cos}\:\mathrm{2}^{{n}+\mathrm{1}} {x}}{\mathrm{sin}\:\mathrm{2}^{{n}+\mathrm{1}} {x}}−\frac{\mathrm{cos}\:{x}}{\mathrm{sin}\:{x}}\right) \\ $$$$=\mathrm{cot}\:{x}−\mathrm{2}^{{n}+\mathrm{1}} \mathrm{cot}\:\mathrm{2}^{{n}+\mathrm{1}} {x} \\ $$
Commented by ajfour last updated on 23/May/17

$$\mathscr{A}{mazing}\:! \\ $$
Answered by Tinkutara last updated on 23/May/17
![= tan x+2tan 2x+2^2 tan 2^2 x+...+2^n tan 2^n x + 2^(n+1) cot2^(n+1) x − 2^(n+1) cot2^(n+1) x = cot x − 2^(n+1) cot2^(n+1) x [∵ tanx + 2tan2x + 2^2 tan2^2 x + ... + 2^n cot2^n x = cotx]](https://www.tinkutara.com/question/Q13742.png)
$$=\:\mathrm{tan}\:{x}+\mathrm{2tan}\:\mathrm{2}{x}+\mathrm{2}^{\mathrm{2}} \mathrm{tan}\:\mathrm{2}^{\mathrm{2}} {x}+…+\mathrm{2}^{{n}} \mathrm{tan}\:\mathrm{2}^{{n}} {x} \\ $$$$+\:\mathrm{2}^{{n}+\mathrm{1}} \mathrm{cot2}^{{n}+\mathrm{1}} {x}\:−\:\mathrm{2}^{{n}+\mathrm{1}} \mathrm{cot2}^{{n}+\mathrm{1}} {x} \\ $$$$=\:\boldsymbol{\mathrm{cot}}\:\boldsymbol{{x}}\:−\:\mathrm{2}^{\boldsymbol{{n}}+\mathrm{1}} \boldsymbol{\mathrm{cot}}\mathrm{2}^{\boldsymbol{{n}}+\mathrm{1}} \boldsymbol{{x}} \\ $$$$\left[\because\:\mathrm{tan}{x}\:+\:\mathrm{2tan2}{x}\:+\:\mathrm{2}^{\mathrm{2}} \mathrm{tan2}^{\mathrm{2}} {x}\:+\:…\:+\right. \\ $$$$\left.\mathrm{2}^{{n}} \mathrm{cot2}^{{n}} {x}\:=\:\mathrm{cot}{x}\right] \\ $$
