Menu Close

tan-1-sec-x-tan-x-dx-

Tinku Tara 0 Comments
Pin




Question Number 152111 by peter frank last updated on 25/Aug/21
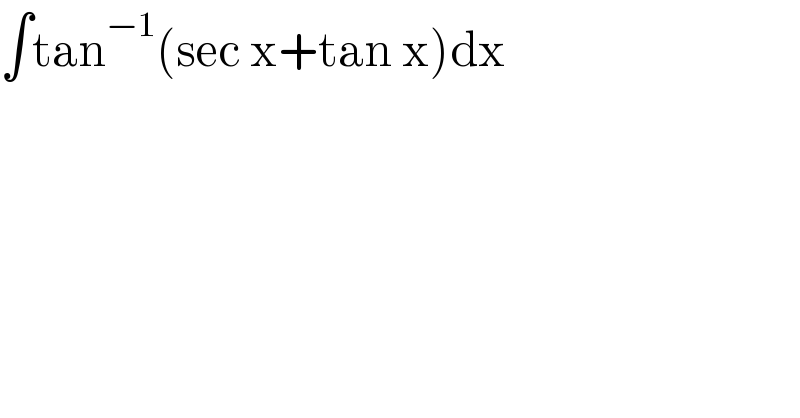
∫tan^(−1) (sec x+tan x)dx
$$\int\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{sec}\:\mathrm{x}+\mathrm{tan}\:\mathrm{x}\right)\mathrm{dx} \\ $$
Answered by peter frank last updated on 26/Aug/21
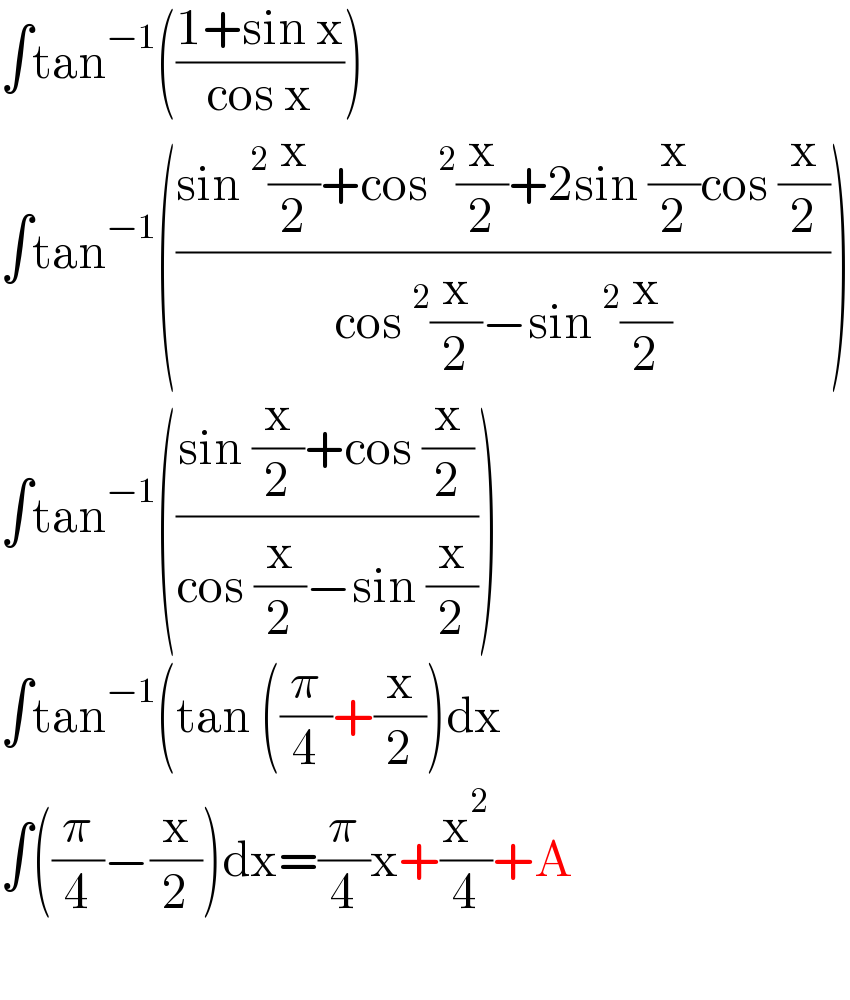
∫tan^(−1) (((1+sin x)/(cos x))) ∫tan^(−1) (((sin^2 (x/2)+cos^2 (x/2)+2sin (x/2)cos (x/2))/(cos^2 (x/2)−sin^2 (x/2)))) ∫tan^(−1) (((sin (x/2)+cos (x/2))/(cos (x/2)−sin (x/2)))) ∫tan^(−1) (tan ((π/4)+(x/2))dx ∫((π/4)−(x/2))dx=(π/4)x+(x^2 /4)+A
$$\int\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{1}+\mathrm{sin}\:\mathrm{x}}{\mathrm{cos}\:\mathrm{x}}\right) \\ $$$$\int\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{sin}\:^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}+\mathrm{cos}\:^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}+\mathrm{2sin}\:\frac{\mathrm{x}}{\mathrm{2}}\mathrm{cos}\:\frac{\mathrm{x}}{\mathrm{2}}}{\mathrm{cos}\:^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}−\mathrm{sin}\:^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}}\right) \\ $$$$\int\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{sin}\:\frac{\mathrm{x}}{\mathrm{2}}+\mathrm{cos}\:\frac{\mathrm{x}}{\mathrm{2}}}{\mathrm{cos}\:\frac{\mathrm{x}}{\mathrm{2}}−\mathrm{sin}\:\frac{\mathrm{x}}{\mathrm{2}}}\right) \\ $$$$\int\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{tan}\:\left(\frac{\pi}{\mathrm{4}}+\frac{\mathrm{x}}{\mathrm{2}}\right)\mathrm{dx}\right. \\ $$$$\int\left(\frac{\pi}{\mathrm{4}}−\frac{\mathrm{x}}{\mathrm{2}}\right)\mathrm{dx}=\frac{\pi}{\mathrm{4}}\mathrm{x}+\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{4}}+\mathrm{A} \\ $$$$ \\ $$
Answered by Olaf_Thorendsen last updated on 26/Aug/21
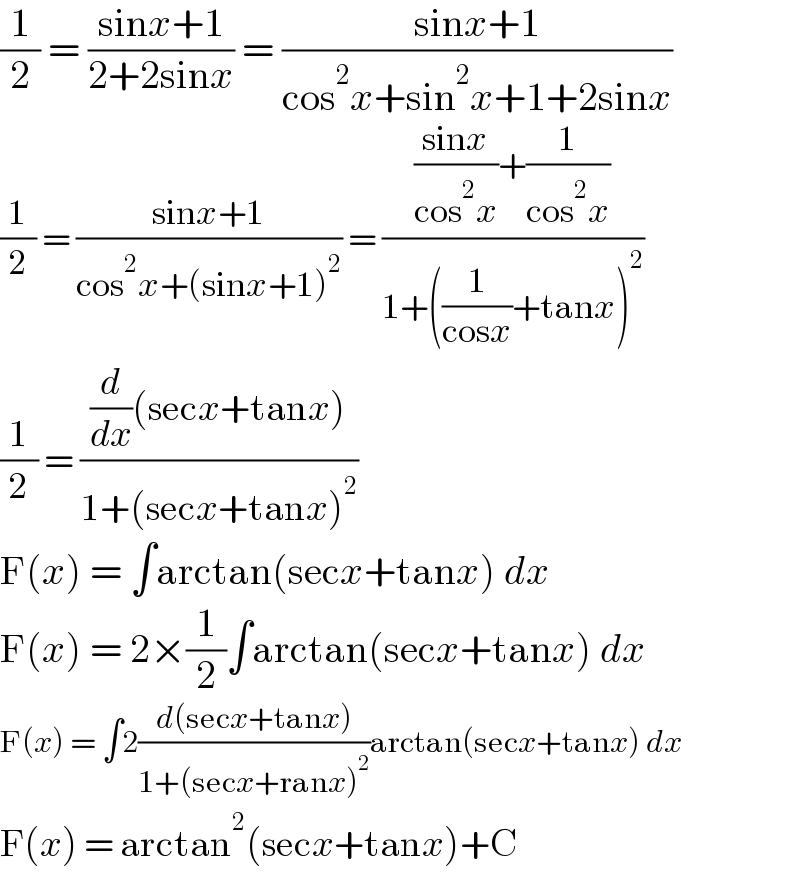
(1/2) = ((sinx+1)/(2+2sinx)) = ((sinx+1)/(cos^2 x+sin^2 x+1+2sinx)) (1/2) = ((sinx+1)/(cos^2 x+(sinx+1)^2 )) = ((((sinx)/(cos^2 x))+(1/(cos^2 x)))/(1+((1/(cosx))+tanx)^2 )) (1/2) = (((d/dx)(secx+tanx))/(1+(secx+tanx)^2 )) F(x) = ∫arctan(secx+tanx) dx F(x) = 2×(1/2)∫arctan(secx+tanx) dx F(x) = ∫2((d(secx+tanx))/(1+(secx+ranx)^2 ))arctan(secx+tanx) dx F(x) = arctan^2 (secx+tanx)+C
$$\frac{\mathrm{1}}{\mathrm{2}}\:=\:\frac{\mathrm{sin}{x}+\mathrm{1}}{\mathrm{2}+\mathrm{2sin}{x}}\:=\:\frac{\mathrm{sin}{x}+\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} {x}+\mathrm{sin}^{\mathrm{2}} {x}+\mathrm{1}+\mathrm{2sin}{x}} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\:=\:\frac{\mathrm{sin}{x}+\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} {x}+\left(\mathrm{sin}{x}+\mathrm{1}\right)^{\mathrm{2}} }\:=\:\frac{\frac{\mathrm{sin}{x}}{\mathrm{cos}^{\mathrm{2}} {x}}+\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} {x}}}{\mathrm{1}+\left(\frac{\mathrm{1}}{\mathrm{cos}{x}}+\mathrm{tan}{x}\right)^{\mathrm{2}} } \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\:=\:\frac{\frac{{d}}{{dx}}\left(\mathrm{sec}{x}+\mathrm{tan}{x}\right)}{\mathrm{1}+\left(\mathrm{sec}{x}+\mathrm{tan}{x}\right)^{\mathrm{2}} } \\ $$$$\mathrm{F}\left({x}\right)\:=\:\int\mathrm{arctan}\left(\mathrm{sec}{x}+\mathrm{tan}{x}\right)\:{dx} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\mathrm{2}×\frac{\mathrm{1}}{\mathrm{2}}\int\mathrm{arctan}\left(\mathrm{sec}{x}+\mathrm{tan}{x}\right)\:{dx} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\int\mathrm{2}\frac{{d}\left(\mathrm{sec}{x}+\mathrm{tan}{x}\right)}{\mathrm{1}+\left(\mathrm{sec}{x}+\mathrm{ran}{x}\right)^{\mathrm{2}} }\mathrm{arctan}\left(\mathrm{sec}{x}+\mathrm{tan}{x}\right)\:{dx} \\ $$$$\mathrm{F}\left({x}\right)\:=\:\mathrm{arctan}^{\mathrm{2}} \left(\mathrm{sec}{x}+\mathrm{tan}{x}\right)+\mathrm{C} \\ $$
Commented by peter frank last updated on 26/Aug/21
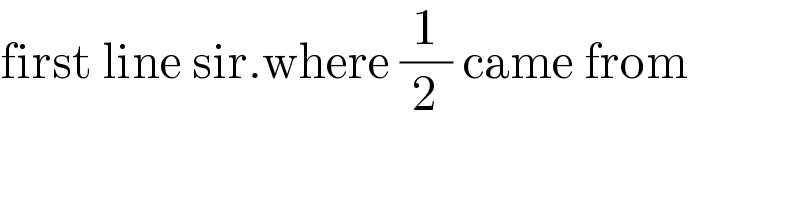
first line sir.where (1/2) came from
$$\mathrm{first}\:\mathrm{line}\:\mathrm{sir}.\mathrm{where}\:\frac{\mathrm{1}}{\mathrm{2}}\:\mathrm{came}\:\mathrm{from} \\ $$
Commented by peter frank last updated on 26/Aug/21
it clear now.i got you
$$\mathrm{it}\:\mathrm{clear}\:\mathrm{now}.\mathrm{i}\:\mathrm{got}\:\mathrm{you} \\ $$
Answered by puissant last updated on 26/Aug/21
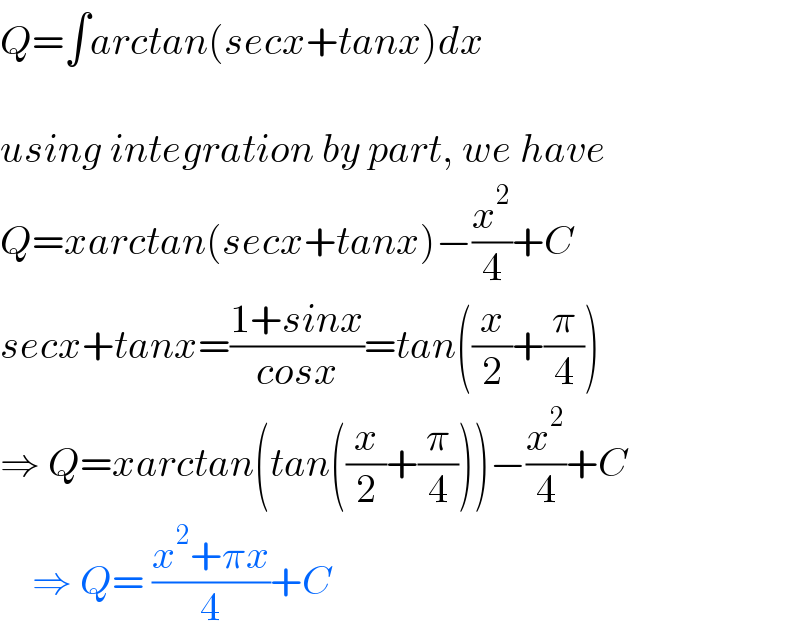
Q=∫arctan(secx+tanx)dx using integration by part, we have Q=xarctan(secx+tanx)−(x^2 /4)+C secx+tanx=((1+sinx)/(cosx))=tan((x/2)+(π/4)) ⇒ Q=xarctan(tan((x/2)+(π/4)))−(x^2 /4)+C ⇒ Q= ((x^2 +πx)/4)+C
$${Q}=\int{arctan}\left({secx}+{tanx}\right){dx} \\ $$$$ \\ $$$${using}\:{integration}\:{by}\:{part},\:{we}\:{have} \\ $$$${Q}={xarctan}\left({secx}+{tanx}\right)−\frac{{x}^{\mathrm{2}} }{\mathrm{4}}+{C} \\ $$$${secx}+{tanx}=\frac{\mathrm{1}+{sinx}}{{cosx}}={tan}\left(\frac{{x}}{\mathrm{2}}+\frac{\pi}{\mathrm{4}}\right) \\ $$$$\Rightarrow\:{Q}={xarctan}\left({tan}\left(\frac{{x}}{\mathrm{2}}+\frac{\pi}{\mathrm{4}}\right)\right)−\frac{{x}^{\mathrm{2}} }{\mathrm{4}}+{C} \\ $$$$\:\:\:\:\Rightarrow\:{Q}=\:\frac{{x}^{\mathrm{2}} +\pi{x}}{\mathrm{4}}+{C} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *