Question Number 23539 by tapan das last updated on 01/Nov/17

$$\int\mathrm{tan}\:^{\mathrm{6}} \mathrm{x}\:\mathrm{dx} \\ $$
Answered by $@ty@m last updated on 01/Nov/17
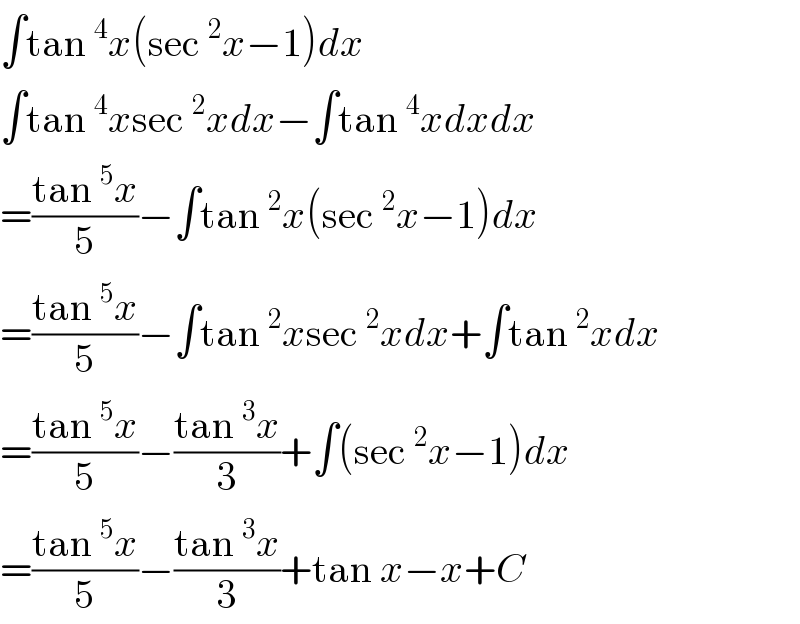
$$\int\mathrm{tan}\:^{\mathrm{4}} {x}\left(\mathrm{sec}\:^{\mathrm{2}} {x}−\mathrm{1}\right){dx} \\ $$$$\int\mathrm{tan}\:^{\mathrm{4}} {x}\mathrm{sec}\:^{\mathrm{2}} {xdx}−\int\mathrm{tan}\:^{\mathrm{4}} {xdxdx} \\ $$$$=\frac{\mathrm{tan}\:^{\mathrm{5}} {x}}{\mathrm{5}}−\int\mathrm{tan}\:^{\mathrm{2}} {x}\left(\mathrm{sec}\:^{\mathrm{2}} {x}−\mathrm{1}\right){dx} \\ $$$$=\frac{\mathrm{tan}\:^{\mathrm{5}} {x}}{\mathrm{5}}−\int\mathrm{tan}\:^{\mathrm{2}} {x}\mathrm{sec}\:^{\mathrm{2}} {xdx}+\int\mathrm{tan}\:^{\mathrm{2}} {xdx} \\ $$$$=\frac{\mathrm{tan}\:^{\mathrm{5}} {x}}{\mathrm{5}}−\frac{\mathrm{tan}\:^{\mathrm{3}} {x}}{\mathrm{3}}+\int\left(\mathrm{sec}\:^{\mathrm{2}} {x}−\mathrm{1}\right){dx} \\ $$$$=\frac{\mathrm{tan}\:^{\mathrm{5}} {x}}{\mathrm{5}}−\frac{\mathrm{tan}\:^{\mathrm{3}} {x}}{\mathrm{3}}+\mathrm{tan}\:{x}−{x}+{C} \\ $$
