Question Number 98382 by bobhans last updated on 13/Jun/20
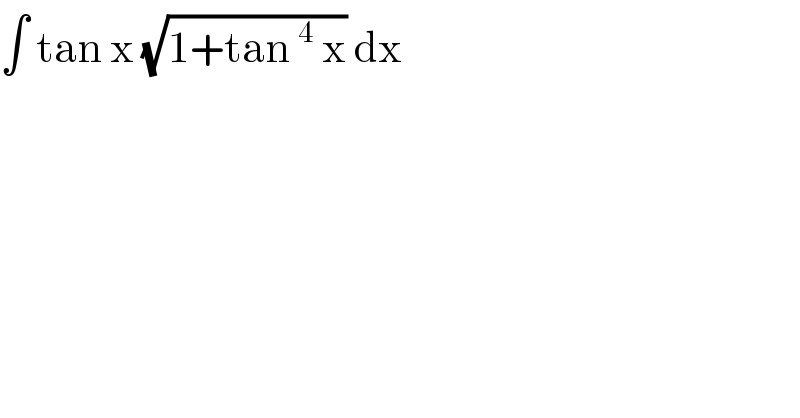
$$\int\:\mathrm{tan}\:\mathrm{x}\:\sqrt{\mathrm{1}+\mathrm{tan}\:^{\mathrm{4}} \:\mathrm{x}}\:\mathrm{dx}\: \\ $$
Commented by john santu last updated on 13/Jun/20
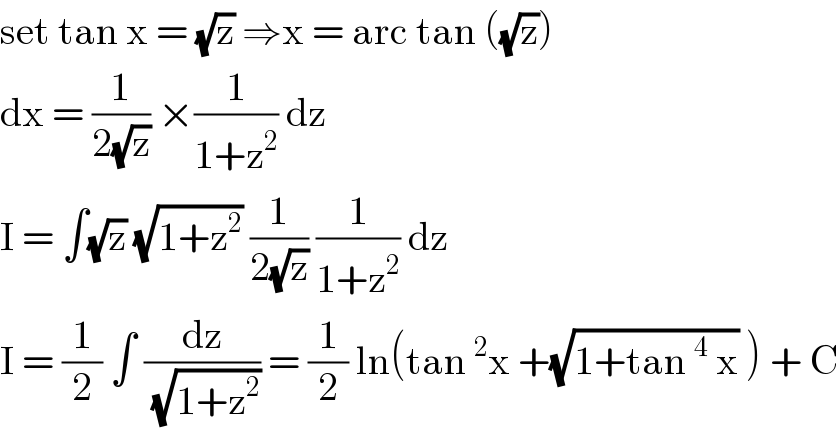
$$\mathrm{set}\:\mathrm{tan}\:\mathrm{x}\:=\:\sqrt{\mathrm{z}}\:\Rightarrow\mathrm{x}\:=\:\mathrm{arc}\:\mathrm{tan}\:\left(\sqrt{\mathrm{z}}\right) \\ $$$$\mathrm{dx}\:=\:\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{z}}}\:×\frac{\mathrm{1}}{\mathrm{1}+\mathrm{z}^{\mathrm{2}} }\:\mathrm{dz}\: \\ $$$$\mathrm{I}\:=\:\int\sqrt{\mathrm{z}}\:\sqrt{\mathrm{1}+\mathrm{z}^{\mathrm{2}} }\:\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{z}}}\:\frac{\mathrm{1}}{\mathrm{1}+\mathrm{z}^{\mathrm{2}} }\:\mathrm{dz}\: \\ $$$$\mathrm{I}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\:\int\:\frac{\mathrm{dz}}{\:\sqrt{\mathrm{1}+\mathrm{z}^{\mathrm{2}} }}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\:\mathrm{ln}\left(\mathrm{tan}\:^{\mathrm{2}} \mathrm{x}\:+\sqrt{\mathrm{1}+\mathrm{tan}\:^{\mathrm{4}} \:\mathrm{x}}\:\right)\:+\:\mathrm{C} \\ $$
Commented by Aziztisffola last updated on 14/Jun/20
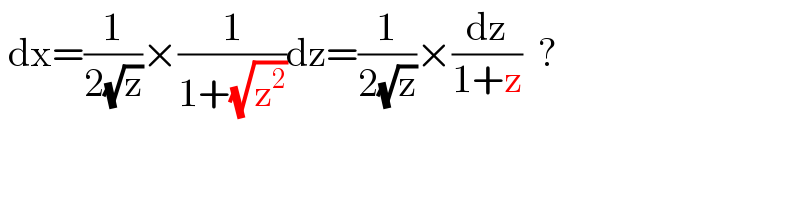
$$\:\mathrm{dx}=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{z}}}×\frac{\mathrm{1}}{\mathrm{1}+\sqrt{\mathrm{z}^{\mathrm{2}} }}\mathrm{dz}=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{z}}}×\frac{\mathrm{dz}}{\mathrm{1}+\mathrm{z}}\:\:? \\ $$
