Question Number 64994 by aliesam last updated on 23/Jul/19
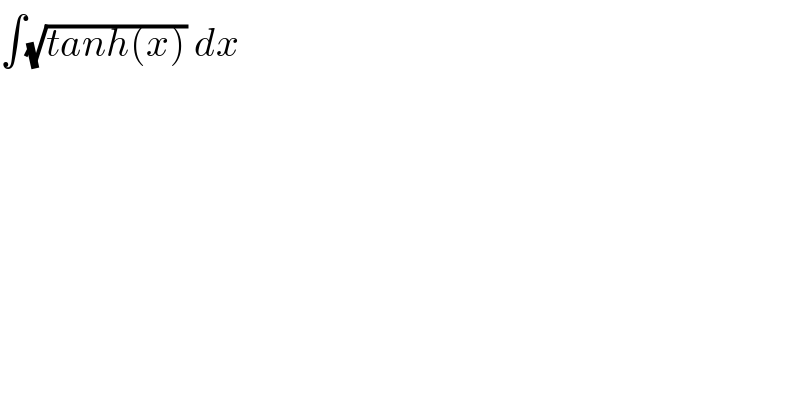
$$\int\sqrt{{tanh}\left({x}\right)}\:{dx} \\ $$
Commented by zakaria elghaouti last updated on 24/Jul/19
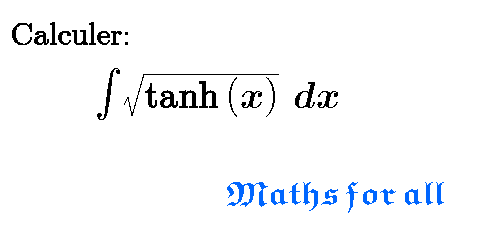
Answered by zakaria elghaouti last updated on 24/Jul/19

Commented by AnjanDey last updated on 24/Jul/19
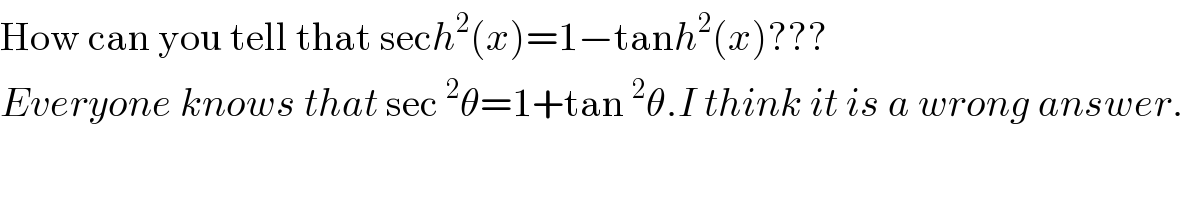
$$\mathrm{How}\:\mathrm{can}\:\mathrm{you}\:\mathrm{tell}\:\mathrm{that}\:\mathrm{sec}{h}^{\mathrm{2}} \left({x}\right)=\mathrm{1}−\mathrm{tan}{h}^{\mathrm{2}} \left({x}\right)??? \\ $$$${Everyone}\:{knows}\:{that}\:\mathrm{sec}\:^{\mathrm{2}} \theta=\mathrm{1}+\mathrm{tan}\:^{\mathrm{2}} \theta.{I}\:{think}\:{it}\:{is}\:{a}\:{wrong}\:{answer}. \\ $$
Commented by Rio Michael last updated on 24/Jul/19
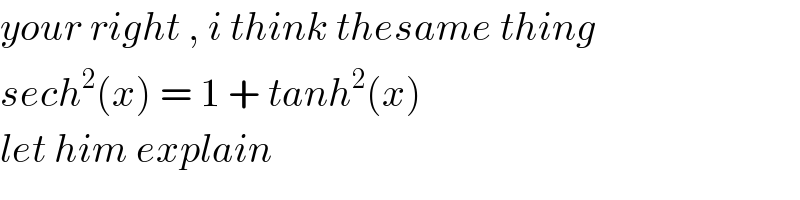
$${your}\:{right}\:,\:{i}\:{think}\:{thesame}\:{thing} \\ $$$${sech}^{\mathrm{2}} \left({x}\right)\:=\:\mathrm{1}\:+\:{tanh}^{\mathrm{2}} \left({x}\right) \\ $$$${let}\:{him}\:{explain} \\ $$
Commented by Tanmay chaudhury last updated on 24/Jul/19

Commented by Tanmay chaudhury last updated on 24/Jul/19
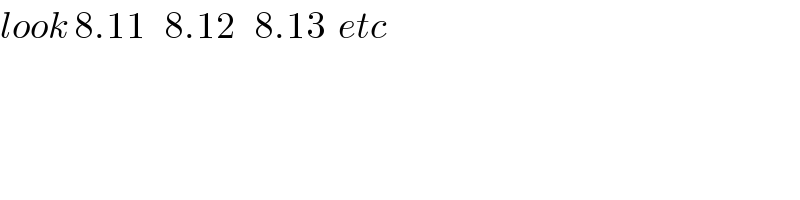
$${look}\:\mathrm{8}.\mathrm{11}\:\:\:\mathrm{8}.\mathrm{12}\:\:\:\mathrm{8}.\mathrm{13}\:\:{etc}\:\:\: \\ $$
Commented by zakaria elghaouti last updated on 24/Jul/19

Commented by AnjanDey last updated on 24/Jul/19

$${Thank}\:{u}\:{for}\:{this}\:{information}… \\ $$$$ \\ $$
Commented by AnjanDey last updated on 24/Jul/19
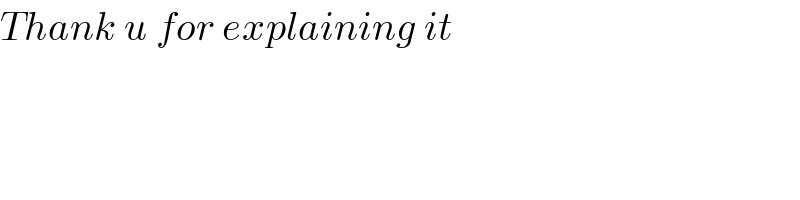
$${Thank}\:{u}\:{for}\:{explaining}\:{it} \\ $$
