Menu Close

tanx-dx-

Tinku Tara 0 Comments
Pin




Question Number 113706 by Rasikh last updated on 15/Sep/20
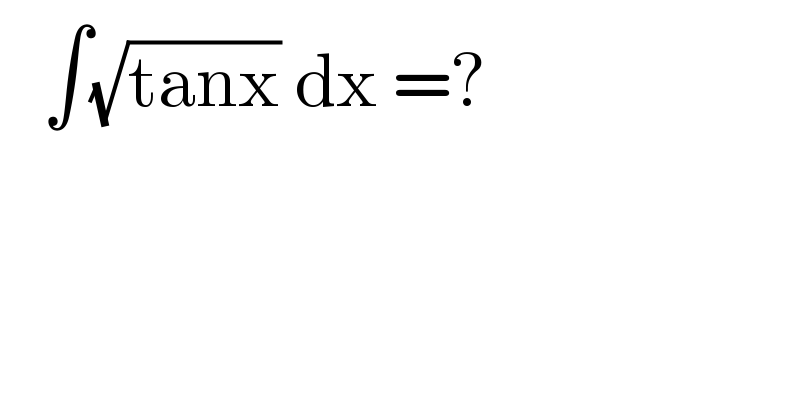
∫(√(tanx)) dx =?
$$\:\:\:\int\sqrt{\mathrm{tanx}}\:\mathrm{dx}\:=?\:\:\:\: \\ $$
Answered by MJS_new last updated on 15/Sep/20
∫(√(tan x)) dx= [t=(√(tan x)) → dx=2cos^2 x (√(tan x)) dt] =2∫(t^2 /(t^4 +1))dt=((√2)/2)∫((t/(t^2 −(√2)t+1))−(t/(t^2 +(√2)t+1)))dt= =((√2)/4)ln ((t^2 −(√2)t+1)/(t^2 +(√2)t+1)) +((√2)/2)(arctan ((√2)t−1) +arctan ((√2)t+1)) with t=(√(tan x))
$$\int\sqrt{\mathrm{tan}\:{x}}\:{dx}= \\ $$$$\:\:\:\:\:\left[{t}=\sqrt{\mathrm{tan}\:{x}}\:\rightarrow\:{dx}=\mathrm{2cos}^{\mathrm{2}} \:{x}\:\sqrt{\mathrm{tan}\:{x}}\:{dt}\right] \\ $$$$=\mathrm{2}\int\frac{{t}^{\mathrm{2}} }{{t}^{\mathrm{4}} +\mathrm{1}}{dt}=\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\int\left(\frac{{t}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}}−\frac{{t}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}}\right){dt}= \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{4}}\mathrm{ln}\:\frac{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}}\:+\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\left(\mathrm{arctan}\:\left(\sqrt{\mathrm{2}}{t}−\mathrm{1}\right)\:+\mathrm{arctan}\:\left(\sqrt{\mathrm{2}}{t}+\mathrm{1}\right)\right) \\ $$$$\mathrm{with}\:{t}=\sqrt{\mathrm{tan}\:{x}} \\ $$
Commented by malwan last updated on 16/Sep/20
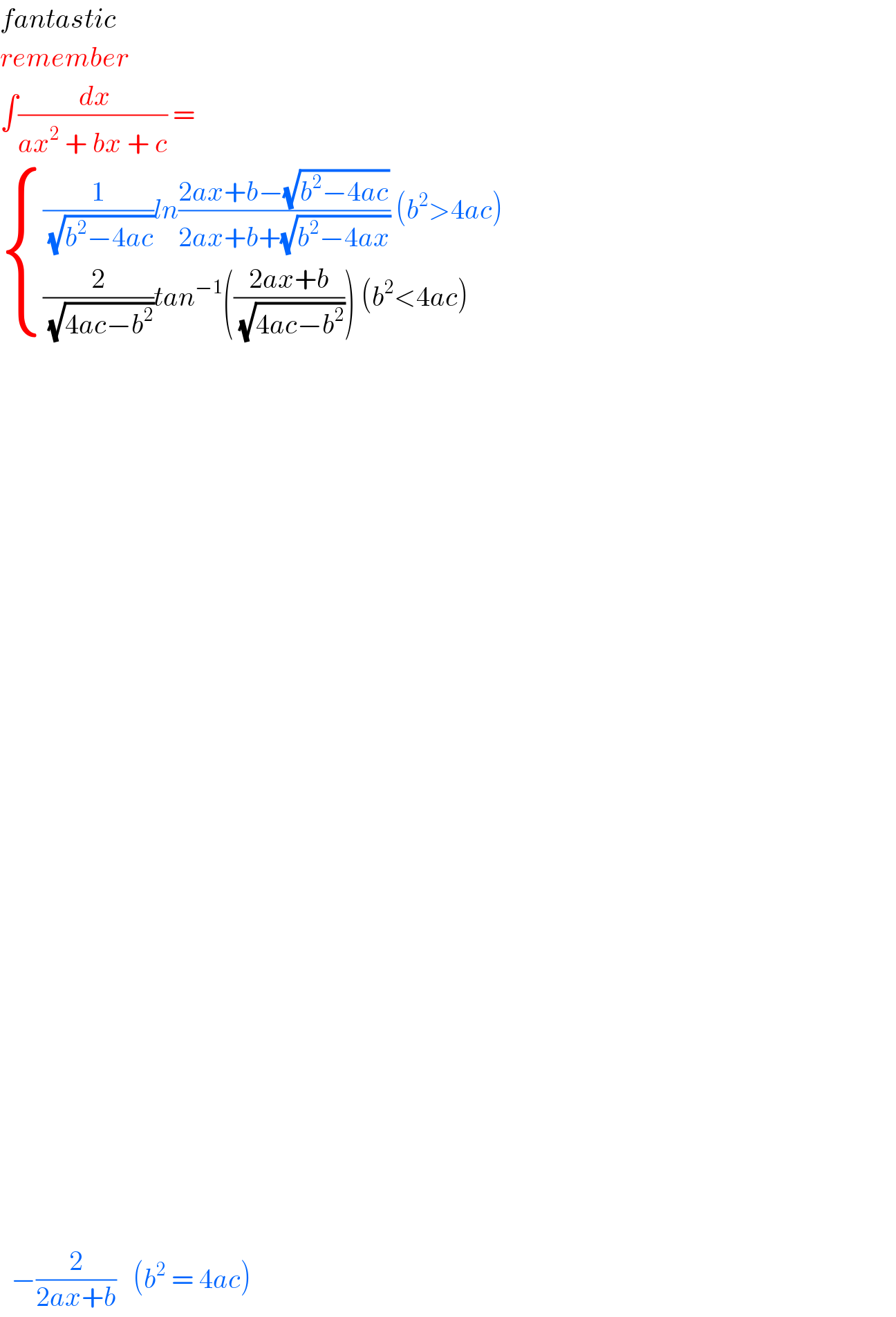
fantastic remember ∫(( dx)/(ax^2 + bx + c)) = { (((1/( (√(b^2 −4ac))))ln((2ax+b−(√(b^2 −4ac)))/(2ax+b+(√(b^2 −4ax)))) (b^2 >4ac))),(((2/( (√(4ac−b^2 ))))tan^(−1) (((2ax+b)/( (√(4ac−b^2 ))))) (b^2 <4ac))) :} −(2/(2ax+b)) (b^2 = 4ac)
$${fantastic}\: \\ $$$${remember} \\ $$$$\int\frac{\:{dx}}{{ax}^{\mathrm{2}} \:+\:{bx}\:+\:{c}}\:= \\ $$$$\begin{cases}{\frac{\mathrm{1}}{\:\sqrt{{b}^{\mathrm{2}} −\mathrm{4}{ac}}}{ln}\frac{\mathrm{2}{ax}+{b}−\sqrt{{b}^{\mathrm{2}} −\mathrm{4}{ac}}}{\mathrm{2}{ax}+{b}+\sqrt{{b}^{\mathrm{2}} −\mathrm{4}{ax}}}\:\left({b}^{\mathrm{2}} >\mathrm{4}{ac}\right)}\\{\frac{\mathrm{2}}{\:\sqrt{\mathrm{4}{ac}−{b}^{\mathrm{2}} }}{tan}^{−\mathrm{1}} \left(\frac{\mathrm{2}{ax}+{b}}{\:\sqrt{\mathrm{4}{ac}−{b}^{\mathrm{2}} }}\right)\:\left({b}^{\mathrm{2}} <\mathrm{4}{ac}\right)}\end{cases} \\ $$$$ \\ $$$$\:\:−\frac{\mathrm{2}}{\mathrm{2}{ax}+{b}}\:\:\:\left({b}^{\mathrm{2}} \:=\:\mathrm{4}{ac}\right) \\ $$
Answered by mathdave last updated on 15/Sep/20
solution let t=(√(tanx)) ,t^2 =tanx,dx=((2t)/(1+t^4 ))dt I=∫(√(tanx))=∫t×((2t)/(1+t^4 ))dt=∫((2t^2 )/(1+t^4 ))dt but 2t^2 =(t^2 −1)+(1+t^2 ) I=∫((t^2 −1)/(1+t^4 ))dt+∫((t^2 +1)/(1+t^4 ))dt=A+B let A=∫((t^2 −1)/(1+t^4 ))dt=∫((1−1/t^2 )/(t^2 +1/t^2 ))dt let t+1/t=p.......(x) (dp/dt)=1−1/t^2 ,dp=(1−1/t^2 )dt....(1) and from (x) (t+1/t)^2 =p^2 , t^2 +1/t^2 =p^2 −((√2))^2 .......(2) ∵A=∫(dp/(p^2 −((√2))^2 )) but ∫(dz/(z^2 −a^2 ))=(1/(2a))ln[((z−a)/(z+a))] A=∫(dp/(p^2 −((√2))^2 ))=(1/(2(√2)))ln[((p−(√2))/(p+(√2)))]=(1/(2(√2)))ln[((t+1/t−(√2))/(t+1/t+(√2)))] ∵∫((t^2 −1)/(1+t^4 ))dt=(1/(2(√2)))ln[((t^2 −t(√2)+1)/(t^2 +t(√2)+1))]........xx and B=∫((t^2 +1)/(1+t^4 ))dt=∫((1+1/t^2 )/(t^2 +1/t^2 ))dt let t−1/t=p,dp=(1+1/t^2 )dt....(1) and (t−1/t)^2 =p^2 ,t^2 +1/t^2 =p^2 +((√2))^2 .....(2) ∫((1+1/t^2 )/(t^2 +1/t^2 ))dt=∫(dp/(p^2 +((√2))^2 )) but ∫(dz/(z^2 +a^2 ))=(1/a)tan^(−1) [(p/( (√2)))] ∫((t^2 +1)/(1+t^4 ))dt=(1/( (√2)))tan^(−1) [((t^2 −1)/(t(√2)))]......xxx ∵∫(√(tanx))dx=(1/(2(√2)))ln[((t^2 −(√2)t+1)/(t^2 +(√2)t+1))]+(1/( (√2)))tan^(−1) [((t^2 −1)/( (√2)t))] ∫(√(tanx))dx=((√2)/4)ln[((tanx−(√(2tanx))+1)/(tanx+(√(2tanx))+1))]+((√2)/2)tan^(−1) [((tanx−1)/( (√(2tanx))))]+k mathdave
$${solution} \\ $$$${let}\:{t}=\sqrt{\mathrm{tan}{x}}\:,{t}^{\mathrm{2}} =\mathrm{tan}{x},{dx}=\frac{\mathrm{2}{t}}{\mathrm{1}+{t}^{\mathrm{4}} }{dt} \\ $$$${I}=\int\sqrt{\mathrm{tan}{x}}=\int{t}×\frac{\mathrm{2}{t}}{\mathrm{1}+{t}^{\mathrm{4}} }{dt}=\int\frac{\mathrm{2}{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{4}} }{dt} \\ $$$${but}\:\mathrm{2}{t}^{\mathrm{2}} =\left({t}^{\mathrm{2}} −\mathrm{1}\right)+\left(\mathrm{1}+{t}^{\mathrm{2}} \right) \\ $$$${I}=\int\frac{{t}^{\mathrm{2}} −\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{4}} }{dt}+\int\frac{{t}^{\mathrm{2}} +\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{4}} }{dt}={A}+{B} \\ $$$${let}\:{A}=\int\frac{{t}^{\mathrm{2}} −\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{4}} }{dt}=\int\frac{\mathrm{1}−\mathrm{1}/{t}^{\mathrm{2}} }{{t}^{\mathrm{2}} +\mathrm{1}/{t}^{\mathrm{2}} }{dt} \\ $$$${let}\:{t}+\mathrm{1}/{t}={p}…….\left({x}\right) \\ $$$$\frac{{dp}}{{dt}}=\mathrm{1}−\mathrm{1}/{t}^{\mathrm{2}} \:,{dp}=\left(\mathrm{1}−\mathrm{1}/{t}^{\mathrm{2}} \right){dt}….\left(\mathrm{1}\right)\:\:{and}\:\:{from}\:\:\left({x}\right) \\ $$$$\left({t}+\mathrm{1}/{t}\right)^{\mathrm{2}} ={p}^{\mathrm{2}} ,\:\:\:\:{t}^{\mathrm{2}} +\mathrm{1}/{t}^{\mathrm{2}} ={p}^{\mathrm{2}} −\left(\sqrt{\mathrm{2}}\right)^{\mathrm{2}} …….\left(\mathrm{2}\right) \\ $$$$\because{A}=\int\frac{{dp}}{{p}^{\mathrm{2}} −\left(\sqrt{\mathrm{2}}\right)^{\mathrm{2}} } \\ $$$${but}\:\int\frac{{dz}}{{z}^{\mathrm{2}} −{a}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}{a}}\mathrm{ln}\left[\frac{{z}−{a}}{{z}+{a}}\right] \\ $$$${A}=\int\frac{{dp}}{{p}^{\mathrm{2}} −\left(\sqrt{\mathrm{2}}\right)^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\mathrm{ln}\left[\frac{{p}−\sqrt{\mathrm{2}}}{{p}+\sqrt{\mathrm{2}}}\right]=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\mathrm{ln}\left[\frac{{t}+\mathrm{1}/{t}−\sqrt{\mathrm{2}}}{{t}+\mathrm{1}/{t}+\sqrt{\mathrm{2}}}\right] \\ $$$$\because\int\frac{{t}^{\mathrm{2}} −\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{4}} }{dt}=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\mathrm{ln}\left[\frac{{t}^{\mathrm{2}} −{t}\sqrt{\mathrm{2}}+\mathrm{1}}{{t}^{\mathrm{2}} +{t}\sqrt{\mathrm{2}}+\mathrm{1}}\right]……..{xx} \\ $$$${and}\:{B}=\int\frac{{t}^{\mathrm{2}} +\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{4}} }{dt}=\int\frac{\mathrm{1}+\mathrm{1}/{t}^{\mathrm{2}} }{{t}^{\mathrm{2}} +\mathrm{1}/{t}^{\mathrm{2}} }{dt} \\ $$$${let}\:{t}−\mathrm{1}/{t}={p},{dp}=\left(\mathrm{1}+\mathrm{1}/{t}^{\mathrm{2}} \right){dt}….\left(\mathrm{1}\right)\:{and}\: \\ $$$$\left({t}−\mathrm{1}/{t}\right)^{\mathrm{2}} ={p}^{\mathrm{2}} ,{t}^{\mathrm{2}} +\mathrm{1}/{t}^{\mathrm{2}} ={p}^{\mathrm{2}} +\left(\sqrt{\mathrm{2}}\right)^{\mathrm{2}} …..\left(\mathrm{2}\right) \\ $$$$\int\frac{\mathrm{1}+\mathrm{1}/{t}^{\mathrm{2}} }{{t}^{\mathrm{2}} +\mathrm{1}/{t}^{\mathrm{2}} }{dt}=\int\frac{{dp}}{{p}^{\mathrm{2}} +\left(\sqrt{\mathrm{2}}\right)^{\mathrm{2}} } \\ $$$${but}\:\int\frac{{dz}}{{z}^{\mathrm{2}} +{a}^{\mathrm{2}} }=\frac{\mathrm{1}}{{a}}\mathrm{tan}^{−\mathrm{1}} \left[\frac{{p}}{\:\sqrt{\mathrm{2}}}\right] \\ $$$$\int\frac{{t}^{\mathrm{2}} +\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{4}} }{dt}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\mathrm{tan}^{−\mathrm{1}} \left[\frac{{t}^{\mathrm{2}} −\mathrm{1}}{{t}\sqrt{\mathrm{2}}}\right]……{xxx} \\ $$$$\because\int\sqrt{\mathrm{tan}{x}}{dx}=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\mathrm{ln}\left[\frac{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}}\right]+\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\mathrm{tan}^{−\mathrm{1}} \left[\frac{{t}^{\mathrm{2}} −\mathrm{1}}{\:\sqrt{\mathrm{2}}{t}}\right] \\ $$$$\int\sqrt{\mathrm{tan}{x}}{dx}=\frac{\sqrt{\mathrm{2}}}{\mathrm{4}}\mathrm{ln}\left[\frac{\mathrm{tan}{x}−\sqrt{\mathrm{2tan}{x}}+\mathrm{1}}{\mathrm{tan}{x}+\sqrt{\mathrm{2tan}{x}}+\mathrm{1}}\right]+\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} \left[\frac{\mathrm{tan}{x}−\mathrm{1}}{\:\sqrt{\mathrm{2tan}{x}}}\right]+{k} \\ $$$${mathdave} \\ $$$$ \\ $$
Commented by mathdave last updated on 15/Sep/20
Commented by Tawa11 last updated on 06/Sep/21
great sir
$$\mathrm{great}\:\mathrm{sir} \\ $$
Answered by mathmax by abdo last updated on 14/Sep/20
I =∫(√(tanx))dx changement (√(tanx))=t give x=arctan(t^2 ) ⇒ I =∫ ((t.(2t))/(1+t^4 )) dt =2 ∫ (t^2 /(1+t^4 )) dt =2 ∫ (1/((1/t^2 )+t^2 )) dt =∫ ((1−(1/t^2 )+1+(1/t^2 ))/((1/t^2 )+t^2 )) dt =∫ ((1−(1/t^2 ))/((t+(1/t))^2 −2))dt(→ t+(1/t)=u)+∫ ((1+(1/t^2 ))/((t−(1/t))^2 +2))dt(→t−(1/t)=v) =∫ (du/(u^2 −2)) +∫ (dv/(v^2 +2)) we have ∫ (du/(u^2 −2)) =(1/(2(√2)))∫((1/(u−(√2)))−(1/(u+(√2))))du =(1/(2(√2)))ln∣((u−(√2))/(u+(√2)))∣ +c_1 =(1/(2(√2)))ln∣((t+(1/t)−(√2))/(t+(1/t)+(√2)))∣ +c_1 =(1/(2(√2)))ln∣((t^2 −(√2)t+1)/(t^2 +(√2)t +1))∣ +c_1 ∫ (dv/(v^2 +2)) =_(v =(√2)α) ∫ (((√2)dα)/(2(1+α^2 ))) =(1/( (√2))) arctan(α)+c2 =(1/( (√2))) arctan((1/( (√2)))(t−(1/t)))+c_2 ⇒ I =(1/(2(√2)))ln∣((t^2 −(√2)t +1)/(t^2 +(√2)t+1))∣ +(1/( (√2))) arctan(((t^2 −1)/(t(√2)))) +C but t=(√(tanx)) ⇒ I =(1/(2(√2)))ln∣((tanx−(√(2tanx))+1)/(tanx +(√(2tanx)) +1))∣ +(1/( (√2))) arctan(((tanx−1)/( (√(2tanx))))) +C
$$\mathrm{I}\:=\int\sqrt{\mathrm{tanx}}\mathrm{dx}\:\mathrm{changement}\:\sqrt{\mathrm{tanx}}=\mathrm{t}\:\mathrm{give}\:\mathrm{x}=\mathrm{arctan}\left(\mathrm{t}^{\mathrm{2}} \right)\:\Rightarrow \\ $$$$\mathrm{I}\:=\int\:\frac{\mathrm{t}.\left(\mathrm{2t}\right)}{\mathrm{1}+\mathrm{t}^{\mathrm{4}} }\:\mathrm{dt}\:=\mathrm{2}\:\int\:\:\frac{\mathrm{t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{t}^{\mathrm{4}} }\:\mathrm{dt}\:=\mathrm{2}\:\int\:\:\frac{\mathrm{1}}{\frac{\mathrm{1}}{\mathrm{t}^{\mathrm{2}} }+\mathrm{t}^{\mathrm{2}} }\:\mathrm{dt} \\ $$$$=\int\:\frac{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{t}^{\mathrm{2}} }+\mathrm{1}+\frac{\mathrm{1}}{\mathrm{t}^{\mathrm{2}} }}{\frac{\mathrm{1}}{\mathrm{t}^{\mathrm{2}} }+\mathrm{t}^{\mathrm{2}} }\:\mathrm{dt}\:=\int\:\:\frac{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{t}^{\mathrm{2}} }}{\left(\mathrm{t}+\frac{\mathrm{1}}{\mathrm{t}}\right)^{\mathrm{2}} −\mathrm{2}}\mathrm{dt}\left(\rightarrow\:\mathrm{t}+\frac{\mathrm{1}}{\mathrm{t}}=\mathrm{u}\right)+\int\:\frac{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{t}^{\mathrm{2}} }}{\left(\mathrm{t}−\frac{\mathrm{1}}{\mathrm{t}}\right)^{\mathrm{2}} \:+\mathrm{2}}\mathrm{dt}\left(\rightarrow\mathrm{t}−\frac{\mathrm{1}}{\mathrm{t}}=\mathrm{v}\right) \\ $$$$=\int\:\frac{\mathrm{du}}{\mathrm{u}^{\mathrm{2}} −\mathrm{2}}\:+\int\:\frac{\mathrm{dv}}{\mathrm{v}^{\mathrm{2}} \:+\mathrm{2}}\:\:\mathrm{we}\:\mathrm{have}\:\int\:\frac{\mathrm{du}}{\mathrm{u}^{\mathrm{2}} −\mathrm{2}}\:=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\int\left(\frac{\mathrm{1}}{\mathrm{u}−\sqrt{\mathrm{2}}}−\frac{\mathrm{1}}{\mathrm{u}+\sqrt{\mathrm{2}}}\right)\mathrm{du} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\mathrm{ln}\mid\frac{\mathrm{u}−\sqrt{\mathrm{2}}}{\mathrm{u}+\sqrt{\mathrm{2}}}\mid\:+\mathrm{c}_{\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\mathrm{ln}\mid\frac{\mathrm{t}+\frac{\mathrm{1}}{\mathrm{t}}−\sqrt{\mathrm{2}}}{\mathrm{t}+\frac{\mathrm{1}}{\mathrm{t}}+\sqrt{\mathrm{2}}}\mid\:+\mathrm{c}_{\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\mathrm{ln}\mid\frac{\mathrm{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}\mathrm{t}+\mathrm{1}}{\mathrm{t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}\mathrm{t}\:+\mathrm{1}}\mid\:+\mathrm{c}_{\mathrm{1}} \\ $$$$\int\:\frac{\mathrm{dv}}{\mathrm{v}^{\mathrm{2}} \:+\mathrm{2}}\:=_{\mathrm{v}\:=\sqrt{\mathrm{2}}\alpha} \:\:\:\int\:\:\frac{\sqrt{\mathrm{2}}\mathrm{d}\alpha}{\mathrm{2}\left(\mathrm{1}+\alpha^{\mathrm{2}} \right)}\:=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:\mathrm{arctan}\left(\alpha\right)+\mathrm{c2} \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:\mathrm{arctan}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\left(\mathrm{t}−\frac{\mathrm{1}}{\mathrm{t}}\right)\right)+\mathrm{c}_{\mathrm{2}} \:\Rightarrow \\ $$$$\mathrm{I}\:=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\mathrm{ln}\mid\frac{\mathrm{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}\mathrm{t}\:+\mathrm{1}}{\mathrm{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}\mathrm{t}+\mathrm{1}}\mid\:+\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:\mathrm{arctan}\left(\frac{\mathrm{t}^{\mathrm{2}} −\mathrm{1}}{\mathrm{t}\sqrt{\mathrm{2}}}\right)\:+\mathrm{C}\:\:\mathrm{but}\:\mathrm{t}=\sqrt{\mathrm{tanx}}\:\Rightarrow \\ $$$$\mathrm{I}\:=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\mathrm{ln}\mid\frac{\mathrm{tanx}−\sqrt{\mathrm{2tanx}}+\mathrm{1}}{\mathrm{tanx}\:+\sqrt{\mathrm{2tanx}}\:+\mathrm{1}}\mid\:+\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:\mathrm{arctan}\left(\frac{\mathrm{tanx}−\mathrm{1}}{\:\sqrt{\mathrm{2tanx}}}\right)\:+\mathrm{C} \\ $$
Answered by john santu last updated on 15/Sep/20
∫ (√(tan x)) dx = ∫ (((√(tan x))+(√(cot x)))/2) dx+∫(((√(tan x))−(√(cot x)))/2) dx = (1/2)∫ ((√(sin x))/( (√(cos x))))+((√(cos x))/( (√(sin x)))) dx+(1/2)∫((√(sin x))/( (√(cos x))))−((√(cos x))/( (√(sin x)))) dx = (1/2)∫((sin x+cos x)/( (√(sin 2x))))dx+(1/2)∫((sin x−cos x)/( (√(sin 2x))))dx = (1/( (√2)))∫ ((sin x+cos x)/( (√(1−(sin x−cos x)^2 ))))dx+(1/( (√2)))∫((sin x−cos x)/( (√((sin x+cos x)^2 −1))))dx = (1/( (√2))) ∫ (dt/( (√(1−t^2 ))))−(1/( (√2)))∫(du/( (√(u^2 −1)))) = (1/( (√2)))sin^(−1) (t)−(1/( (√2)))ln (u+(√(u^2 −1)))+c = (1/( (√2))) sin^(−1) (sin x−cos x)−(1/( (√2)))ln (sin x+cos x+(√(sin 2x)))+c [ note t = sin x−cos x , u=sin x+cos x ]
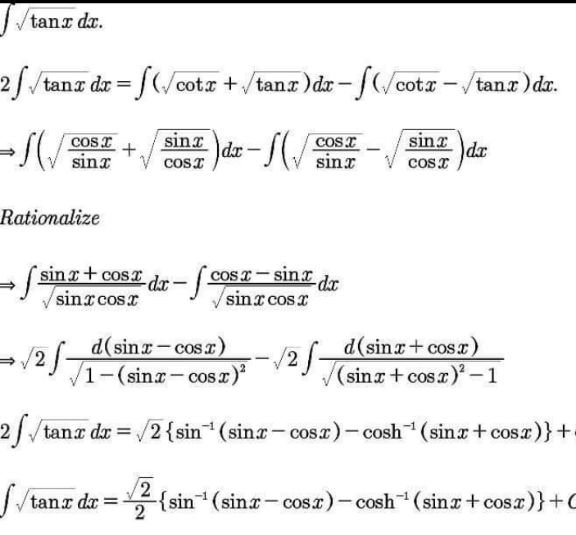
$$\int\:\sqrt{\mathrm{tan}\:{x}}\:{dx}\:=\:\int\:\frac{\sqrt{\mathrm{tan}\:{x}}+\sqrt{\mathrm{cot}\:{x}}}{\mathrm{2}}\:{dx}+\int\frac{\sqrt{\mathrm{tan}\:{x}}−\sqrt{\mathrm{cot}\:{x}}}{\mathrm{2}}\:{dx} \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}}\int\:\frac{\sqrt{\mathrm{sin}\:{x}}}{\:\sqrt{\mathrm{cos}\:{x}}}+\frac{\sqrt{\mathrm{cos}\:{x}}}{\:\sqrt{\mathrm{sin}\:{x}}}\:{dx}+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\sqrt{\mathrm{sin}\:{x}}}{\:\sqrt{\mathrm{cos}\:{x}}}−\frac{\sqrt{\mathrm{cos}\:{x}}}{\:\sqrt{\mathrm{sin}\:{x}}}\:{dx} \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{sin}\:{x}+\mathrm{cos}\:{x}}{\:\sqrt{\mathrm{sin}\:\mathrm{2}{x}}}{dx}+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{sin}\:{x}−\mathrm{cos}\:{x}}{\:\sqrt{\mathrm{sin}\:\mathrm{2}{x}}}{dx} \\ $$$$=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\int\:\frac{\mathrm{sin}\:{x}+\mathrm{cos}\:{x}}{\:\sqrt{\mathrm{1}−\left(\mathrm{sin}\:{x}−\mathrm{cos}\:{x}\right)^{\mathrm{2}} }}{dx}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\int\frac{\mathrm{sin}\:{x}−\mathrm{cos}\:{x}}{\:\sqrt{\left(\mathrm{sin}\:{x}+\mathrm{cos}\:{x}\right)^{\mathrm{2}} −\mathrm{1}}}{dx} \\ $$$$=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:\int\:\frac{{dt}}{\:\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\int\frac{{du}}{\:\sqrt{{u}^{\mathrm{2}} −\mathrm{1}}} \\ $$$$=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\mathrm{sin}^{−\mathrm{1}} \left({t}\right)−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\mathrm{ln}\:\left({u}+\sqrt{{u}^{\mathrm{2}} −\mathrm{1}}\right)+{c} \\ $$$$=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:\mathrm{sin}^{−\mathrm{1}} \left(\mathrm{sin}\:{x}−\mathrm{cos}\:{x}\right)−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\mathrm{ln}\:\left(\mathrm{sin}\:{x}+\mathrm{cos}\:{x}+\sqrt{\mathrm{sin}\:\mathrm{2}{x}}\right)+{c} \\ $$$$\left[\:{note}\:{t}\:=\:\mathrm{sin}\:{x}−\mathrm{cos}\:{x}\:,\:{u}=\mathrm{sin}\:{x}+\mathrm{cos}\:{x}\:\right] \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *