Menu Close

The-annual-salaries-of-employees-in-a-large-company-are-approximately-normally-disributed-with-a-mean-of-50-000-and-a-standard-deviation-of-20-000-a-what-percent-of-people-earn-less-than-40-000-

Tinku Tara 0 Comments
Pin




Question Number 97803 by pete last updated on 09/Jun/20
The annual salaries of employees in a large company are approximately normally disributed with a mean of $50,000 and a standard deviation of $20,000. a. what percent of people earn less than $40,000? b. what percent of people earn between $45,000 and $65,000? c. what percent of people earn more than $70,000?
$$\mathrm{The}\:\mathrm{annual}\:\mathrm{salaries}\:\mathrm{of}\:\mathrm{employees}\:\mathrm{in}\:\mathrm{a}\:\mathrm{large} \\ $$$$\mathrm{company}\:\mathrm{are}\:\mathrm{approximately}\:\mathrm{normally}\: \\ $$$$\mathrm{disributed}\:\mathrm{with}\:\mathrm{a}\:\mathrm{mean}\:\mathrm{of}\:\$\mathrm{50},\mathrm{000}\:\mathrm{and}\:\mathrm{a}\:\mathrm{standard} \\ $$$$\mathrm{deviation}\:\mathrm{of}\:\$\mathrm{20},\mathrm{000}. \\ $$$$\mathrm{a}.\:\mathrm{what}\:\mathrm{percent}\:\mathrm{of}\:\mathrm{people}\:\mathrm{earn}\:\mathrm{less}\:\mathrm{than} \\ $$$$\$\mathrm{40},\mathrm{000}? \\ $$$$\mathrm{b}.\:\mathrm{what}\:\mathrm{percent}\:\mathrm{of}\:\mathrm{people}\:\mathrm{earn}\:\mathrm{between} \\ $$$$\$\mathrm{45},\mathrm{000}\:\mathrm{and}\:\$\mathrm{65},\mathrm{000}? \\ $$$$\mathrm{c}.\:\mathrm{what}\:\mathrm{percent}\:\mathrm{of}\:\mathrm{people}\:\mathrm{earn}\:\mathrm{more}\:\mathrm{than} \\ $$$$\$\mathrm{70},\mathrm{000}? \\ $$
Answered by Rio Michael last updated on 10/Jun/20
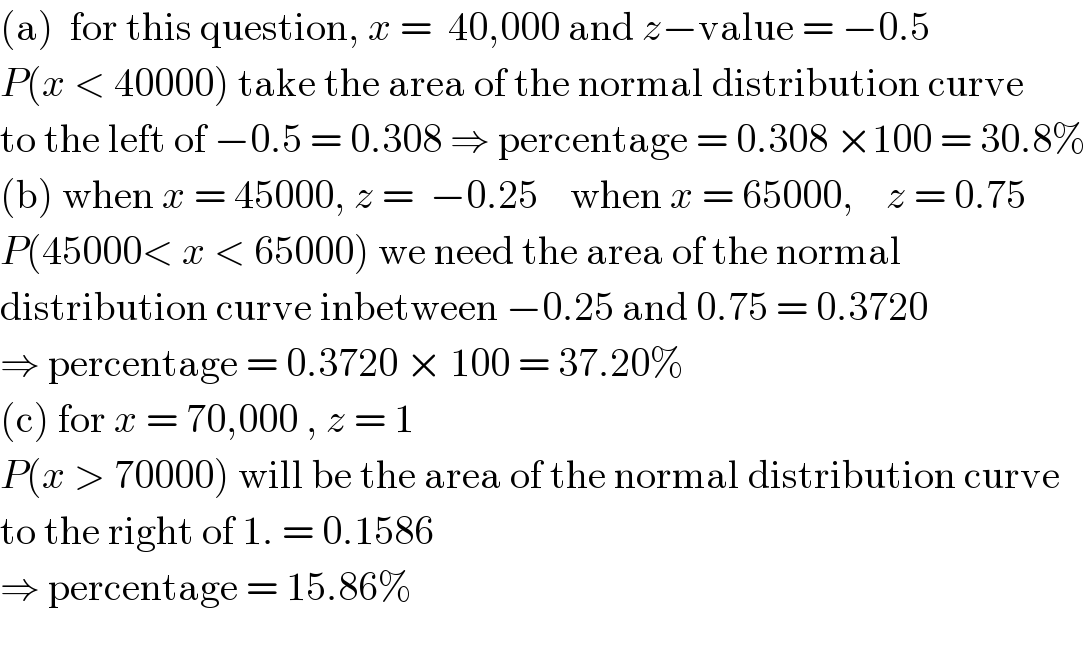
(a) for this question, x = 40,000 and z−value = −0.5 P(x < 40000) take the area of the normal distribution curve to the left of −0.5 = 0.308 ⇒ percentage = 0.308 ×100 = 30.8% (b) when x = 45000, z = −0.25 when x = 65000, z = 0.75 P(45000< x < 65000) we need the area of the normal distribution curve inbetween −0.25 and 0.75 = 0.3720 ⇒ percentage = 0.3720 × 100 = 37.20% (c) for x = 70,000 , z = 1 P(x > 70000) will be the area of the normal distribution curve to the right of 1. = 0.1586 ⇒ percentage = 15.86%
$$\left(\mathrm{a}\right)\:\:\mathrm{for}\:\mathrm{this}\:\mathrm{question},\:{x}\:=\:\:\mathrm{40},\mathrm{000}\:\mathrm{and}\:{z}−\mathrm{value}\:=\:−\mathrm{0}.\mathrm{5} \\ $$$${P}\left({x}\:<\:\mathrm{40000}\right)\:\mathrm{take}\:\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{normal}\:\mathrm{distribution}\:\mathrm{curve} \\ $$$$\mathrm{to}\:\mathrm{the}\:\mathrm{left}\:\mathrm{of}\:−\mathrm{0}.\mathrm{5}\:=\:\mathrm{0}.\mathrm{308}\:\Rightarrow\:\mathrm{percentage}\:=\:\mathrm{0}.\mathrm{308}\:×\mathrm{100}\:=\:\mathrm{30}.\mathrm{8\%} \\ $$$$\left(\mathrm{b}\right)\:\mathrm{when}\:{x}\:=\:\mathrm{45000},\:{z}\:=\:\:−\mathrm{0}.\mathrm{25}\:\:\:\:\mathrm{when}\:{x}\:=\:\mathrm{65000},\:\:\:\:{z}\:=\:\mathrm{0}.\mathrm{75} \\ $$$${P}\left(\mathrm{45000}<\:{x}\:<\:\mathrm{65000}\right)\:\mathrm{we}\:\mathrm{need}\:\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{normal} \\ $$$$\mathrm{distribution}\:\mathrm{curve}\:\mathrm{inbetween}\:−\mathrm{0}.\mathrm{25}\:\mathrm{and}\:\mathrm{0}.\mathrm{75}\:=\:\mathrm{0}.\mathrm{3720}\: \\ $$$$\Rightarrow\:\mathrm{percentage}\:=\:\mathrm{0}.\mathrm{3720}\:×\:\mathrm{100}\:=\:\mathrm{37}.\mathrm{20\%} \\ $$$$\left(\mathrm{c}\right)\:\mathrm{for}\:{x}\:=\:\mathrm{70},\mathrm{000}\:,\:{z}\:=\:\mathrm{1} \\ $$$${P}\left({x}\:>\:\mathrm{70000}\right)\:\mathrm{will}\:\mathrm{be}\:\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{normal}\:\mathrm{distribution}\:\mathrm{curve} \\ $$$$\mathrm{to}\:\mathrm{the}\:\mathrm{right}\:\mathrm{of}\:\mathrm{1}.\:=\:\mathrm{0}.\mathrm{1586}\: \\ $$$$\Rightarrow\:\mathrm{percentage}\:=\:\mathrm{15}.\mathrm{86\%} \\ $$$$\: \\ $$
Commented by pete last updated on 10/Jun/20
Thank u very much sir Rio
$$\mathrm{Thank}\:\mathrm{u}\:\mathrm{very}\:\mathrm{much}\:\mathrm{sir}\:\mathrm{Rio} \\ $$
Commented by Rio Michael last updated on 10/Jun/20
You are welcome
$$\mathrm{You}\:\mathrm{are}\:\mathrm{welcome} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *