Question Number 180335 by SLVR last updated on 10/Nov/22
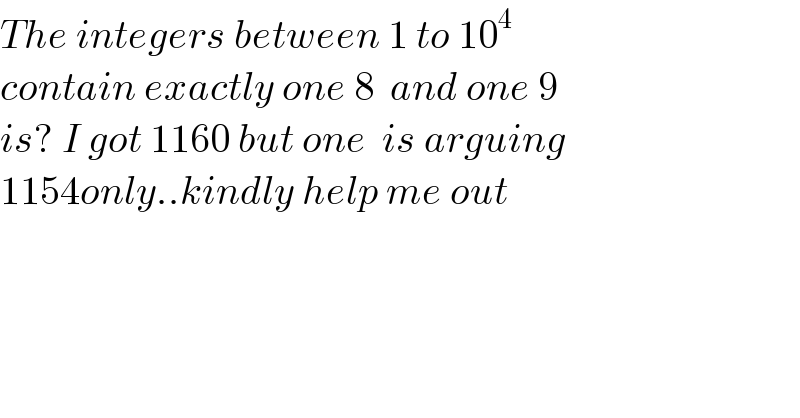
$${The}\:{integers}\:{between}\:\mathrm{1}\:{to}\:\mathrm{10}^{\mathrm{4}} \\ $$$${contain}\:{exactly}\:{one}\:\mathrm{8}\:\:{and}\:{one}\:\mathrm{9} \\ $$$${is}?\:{I}\:{got}\:\mathrm{1160}\:{but}\:{one}\:\:{is}\:{arguing} \\ $$$$\mathrm{1154}{only}..{kindly}\:{help}\:{me}\:{out} \\ $$
Commented by mr W last updated on 10/Nov/22
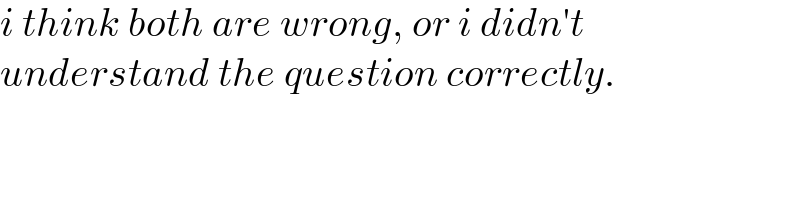
$${i}\:{think}\:{both}\:{are}\:{wrong},\:{or}\:{i}\:{didn}'{t} \\ $$$${understand}\:{the}\:{question}\:{correctly}. \\ $$
Answered by mr W last updated on 10/Nov/22

$$\left.\mathrm{1}\right)\:\mathrm{2}\:{digit}\:{numbers}: \\ $$$$\mathrm{89}\:{and}\:\mathrm{98}\:\Rightarrow\mathrm{2}\:{numbers} \\ $$$$ \\ $$$$\left.\mathrm{2}\right)\:\mathrm{3}\:{digit}\:{numbers}: \\ $$$${Y}\mathrm{89},\:\mathrm{8}{X}\mathrm{9},\:\mathrm{89}{X}\: \\ $$$$\Rightarrow\:\mathrm{2}×\left(\mathrm{7}+\mathrm{8}+\mathrm{8}\right)=\mathrm{46}\:{numbers} \\ $$$${X}=\mathrm{0},\mathrm{1},\mathrm{2},…,\mathrm{7}\: \\ $$$${Y}=\mathrm{1},\mathrm{2},…,\mathrm{7} \\ $$$$ \\ $$$$\left.\mathrm{3}\right)\:\mathrm{4}\:{digit}\:{numbers}: \\ $$$${YX}\mathrm{89}\:\Rightarrow\mathrm{2}×\mathrm{7}×\mathrm{8}=\mathrm{112} \\ $$$${Y}\mathrm{8}{X}\mathrm{9}\:\Rightarrow\mathrm{2}×\mathrm{7}×\mathrm{8} \\ $$$${Y}\mathrm{89}{X}\:\Rightarrow\mathrm{2}×\mathrm{7}×\mathrm{8} \\ $$$$\mathrm{8}{XX}\mathrm{9}\:\Rightarrow\mathrm{2}×\mathrm{8}×\mathrm{8}=\mathrm{128} \\ $$$$\mathrm{8}{X}\mathrm{9}{X}\:\Rightarrow\mathrm{2}×\mathrm{8}×\mathrm{8} \\ $$$$\mathrm{89}{XX}\:\Rightarrow\mathrm{2}×\mathrm{8}×\mathrm{8} \\ $$$$\Rightarrow\mathrm{3}×\mathrm{112}+\mathrm{3}×\mathrm{128}=\mathrm{720} \\ $$$$ \\ $$$${totally}:\:\mathrm{2}+\mathrm{46}+\mathrm{720}=\mathrm{768} \\ $$
Commented by SLVR last updated on 11/Nov/22
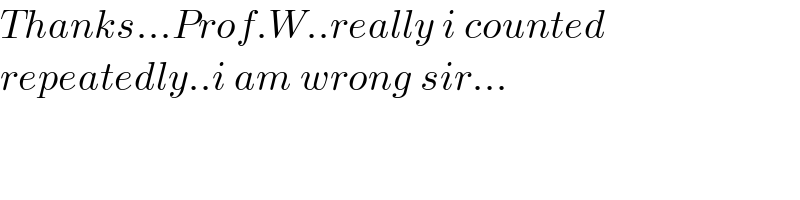
$${Thanks}…{Prof}.{W}..{really}\:{i}\:{counted} \\ $$$${repeatedly}..{i}\:{am}\:{wrong}\:{sir}… \\ $$
Answered by Acem last updated on 10/Nov/22
![You have three general cases 2 dig 3 dig 4 dig 89 x89 xx89 with 2! permutation^(8,9) x89 3! = 3 ×2^(8,9) xx89 ((4!)/(2!))= 12 = 6 ×2^(8,9) well let′s note cases • x′89 , 8x9 , 89x ∣_( x: 0→ 7) ^(x′: 1→ 7) • x′x89, x′8x9 , x′89x ∣_(x′: 1→7) ^(x: 0→7) 8xx9, 8x9x , 89xx^(x: 0→7) Num_(Numb.) = 2 [1+(7+2×8)+3(7×8+8×8)] = 2[1+23+24(15)]= 768 numbers](https://www.tinkutara.com/question/Q180349.png)
$${You}\:{have}\:{three}\:{general}\:{cases} \\ $$$$\:\mathrm{2}\:{dig}\:\:\:\:\:\:\:\:\:\mathrm{3}\:{dig}\:\:\:\:\:\:\:\mathrm{4}\:{dig} \\ $$$$\:\:\mathrm{89}\:\:\:\:\:\:\:\:\:\:\:\:\:\:{x}\mathrm{89}\:\:\:\:\:\:\:\:\:\:{xx}\mathrm{89}\:\:\:{with}\:\mathrm{2}!\:{permutation}^{\mathrm{8},\mathrm{9}} \\ $$$$ \\ $$$$\:{x}\mathrm{89}\:\:\:\:\:\:\:\mathrm{3}!\:=\:\mathrm{3}\:×\mathrm{2}^{\mathrm{8},\mathrm{9}} \\ $$$$\:{xx}\mathrm{89}\:\:\:\:\frac{\mathrm{4}!}{\mathrm{2}!}=\:\mathrm{12}\:=\:\mathrm{6}\:×\mathrm{2}^{\mathrm{8},\mathrm{9}} \:\:\: \\ $$$${well}\:{let}'{s}\:{note}\:{cases} \\ $$$$\bullet\:{x}'\mathrm{89}\:,\:\mathrm{8}{x}\mathrm{9}\:,\:\mathrm{89}{x}\:\mid_{\:{x}:\:\mathrm{0}\rightarrow\:\mathrm{7}} ^{{x}':\:\mathrm{1}\rightarrow\:\mathrm{7}} \: \\ $$$$ \\ $$$$\bullet\:{x}'{x}\mathrm{89},\:{x}'\mathrm{8}{x}\mathrm{9}\:,\:{x}'\mathrm{89}{x}\:\mid_{{x}':\:\mathrm{1}\rightarrow\mathrm{7}} ^{{x}:\:\mathrm{0}\rightarrow\mathrm{7}} \\ $$$$\:\:\:\:\mathrm{8}{xx}\mathrm{9},\:\mathrm{8}{x}\mathrm{9}{x}\:,\:\mathrm{89}{xx}\:^{{x}:\:\mathrm{0}\rightarrow\mathrm{7}} \\ $$$$ \\ $$$$\:{Num}_{{Numb}.} =\:\mathrm{2}\:\left[\mathrm{1}+\left(\mathrm{7}+\mathrm{2}×\mathrm{8}\right)+\mathrm{3}\left(\mathrm{7}×\mathrm{8}+\mathrm{8}×\mathrm{8}\right)\right] \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{2}\left[\mathrm{1}+\mathrm{23}+\mathrm{24}\left(\mathrm{15}\right)\right]=\:\mathrm{768}\:{numbers} \\ $$$$ \\ $$
