Question Number 173371 by sujeet924 last updated on 10/Jul/22
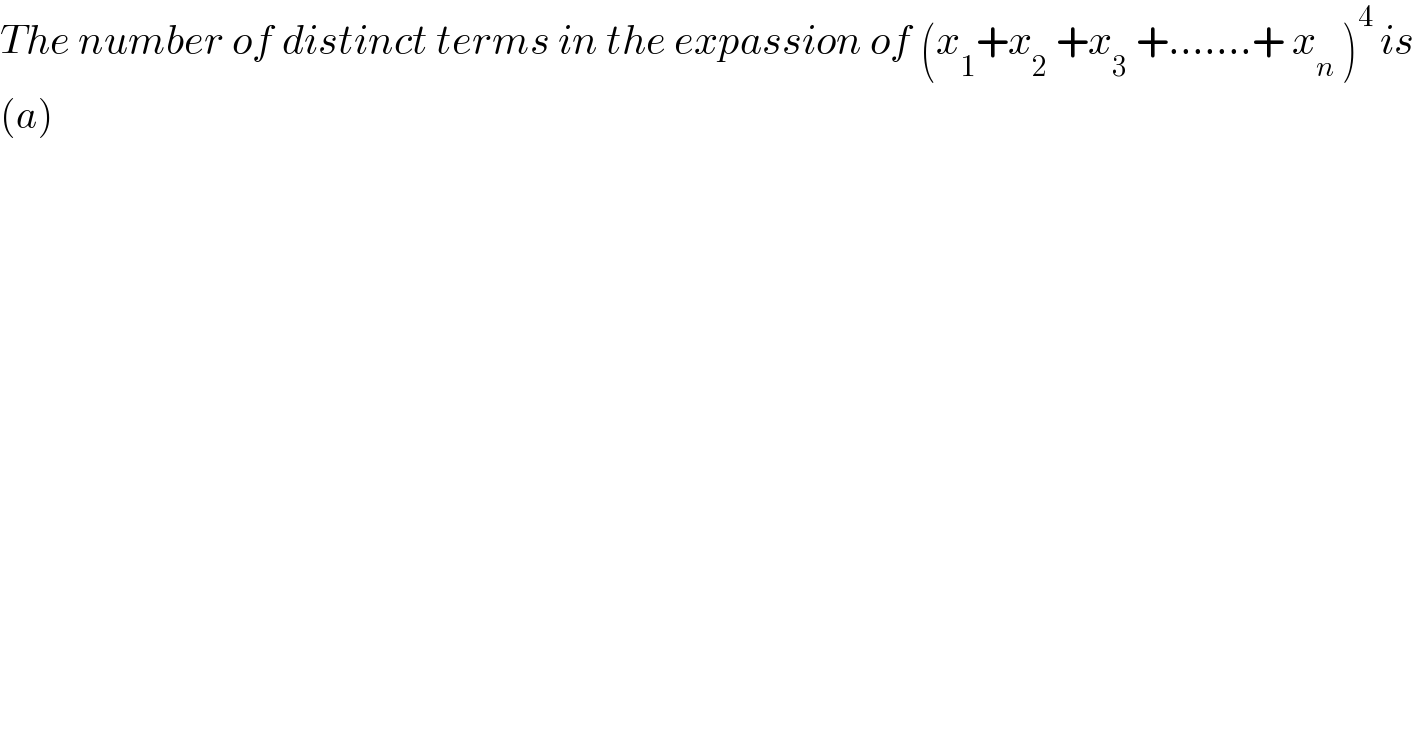
$${The}\:{number}\:{of}\:{distinct}\:{terms}\:{in}\:{the}\:{expassion}\:{of}\:\left({x}_{\mathrm{1}} +{x}_{\mathrm{2}} \:+{x}_{\mathrm{3}} \:+…….+\:{x}_{{n}} \:\right)^{\mathrm{4}\:} {is} \\ $$$$\left({a}\right)\: \\ $$
Commented by mr W last updated on 10/Jul/22

$${in}\:{expansion}\:{of}\:\left({x}_{\mathrm{1}} +{x}_{\mathrm{2}} +{x}_{\mathrm{3}} +…+{x}_{{n}} \right)^{{m}} \\ $$$${the}\:{general}\:{term}\:{is}\:{x}_{\mathrm{1}} ^{{k}_{\mathrm{1}} } {x}_{\mathrm{2}} ^{{k}_{\mathrm{2}} } {x}_{\mathrm{3}} ^{{k}_{\mathrm{3}} } …{x}_{{n}} ^{{k}_{{n}} } \\ $$$${with}\:\mathrm{0}\leqslant{k}_{{i}} \leqslant{m}\:{and}\:{k}_{\mathrm{1}} +{k}_{\mathrm{2}} +{k}_{\mathrm{3}} +…+{k}_{{n}} ={m} \\ $$$${there}\:{are}\:{C}_{{m}} ^{{n}+{m}−\mathrm{1}} \:{possibilities}.\:{i}.{e}. \\ $$$${the}\:{number}\:{of}\:{distinc}\:{terms}\:{is} \\ $$$${C}_{{m}} ^{{n}+{m}−\mathrm{1}} .\: \\ $$$${in}\:{case}\:{of}\:{m}=\mathrm{4},\:{it}'{s}\:{C}_{\mathrm{4}} ^{{n}+\mathrm{3}} . \\ $$
Commented by Tawa11 last updated on 13/Jul/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
