Question Number 173842 by byaw last updated on 19/Jul/22

$$ \\ $$The probability that an athlete will not win any of the three races is 1/4.
If the athlete runs in all the races, what is the probability that the athlete will win:
(I) only the second race:
If the athlete runs in all the races, what is the probability that the athlete will win:
(I) only the second race:
(ii) all the three races
(iii) only two of the races?
Answered by mr W last updated on 20/Jul/22
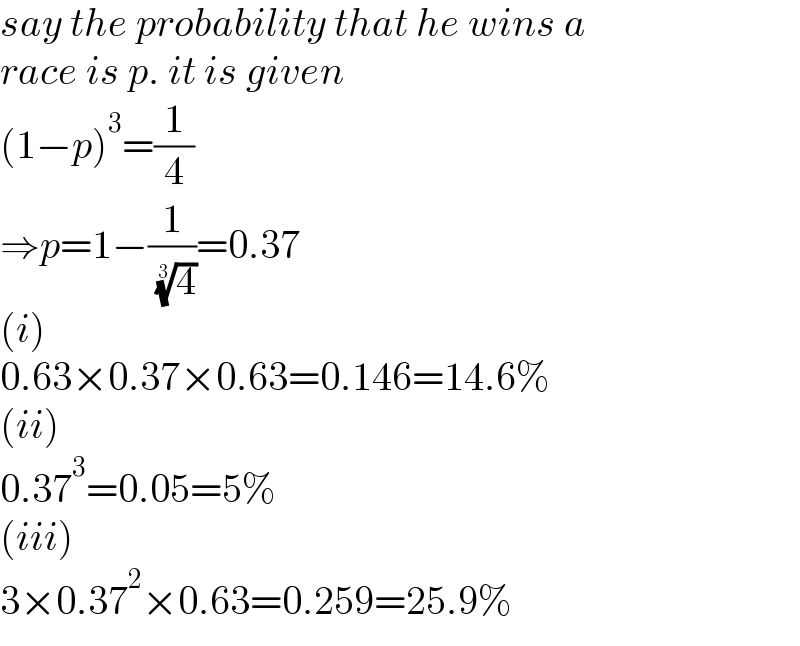
$${say}\:{the}\:{probability}\:{that}\:{he}\:{wins}\:{a} \\ $$$${race}\:{is}\:{p}.\:{it}\:{is}\:{given} \\ $$$$\left(\mathrm{1}−{p}\right)^{\mathrm{3}} =\frac{\mathrm{1}}{\mathrm{4}}\: \\ $$$$\Rightarrow{p}=\mathrm{1}−\frac{\mathrm{1}}{\:\sqrt[{\mathrm{3}}]{\mathrm{4}}}=\mathrm{0}.\mathrm{37} \\ $$$$\left({i}\right) \\ $$$$\mathrm{0}.\mathrm{63}×\mathrm{0}.\mathrm{37}×\mathrm{0}.\mathrm{63}=\mathrm{0}.\mathrm{146}=\mathrm{14}.\mathrm{6\%} \\ $$$$\left({ii}\right) \\ $$$$\mathrm{0}.\mathrm{37}^{\mathrm{3}} =\mathrm{0}.\mathrm{05}=\mathrm{5\%} \\ $$$$\left({iii}\right) \\ $$$$\mathrm{3}×\mathrm{0}.\mathrm{37}^{\mathrm{2}} ×\mathrm{0}.\mathrm{63}=\mathrm{0}.\mathrm{259}=\mathrm{25}.\mathrm{9\%} \\ $$
