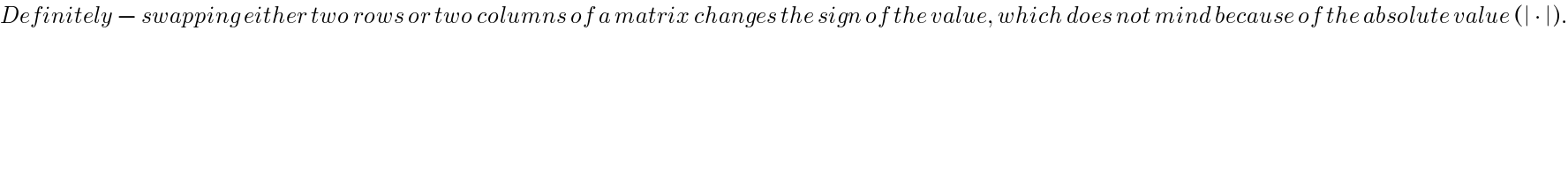The-sides-of-a-cubical-container-are-given-by-the-vectors-a-1-2i-3j-4k-a-2-i-2j-3k-a-3-3i-6j-4k-What-is-the-volumr-of-the-container-

Question Number 31933 by NECx last updated on 17/Mar/18

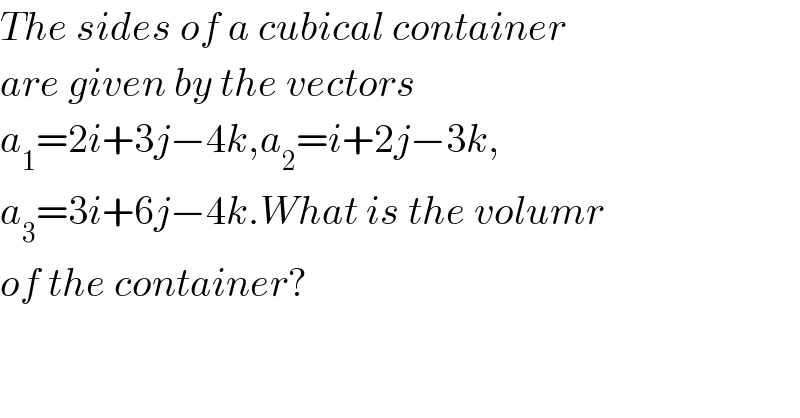
T h e s i d e s o f a c u b i c a l c o n t a i n e r

a r e g i v e n b y t h e v e c t o r s

a_{1} = 2 i + 3 j - 4 k, a_{2} = i + 2 j - 3 k,

a_{3} = 3 i + 6 j - 4 k . W h a t i s t h e v o l u m r

o f t h e c o n t a i n e r ?

Answered by JDamian last updated on 17/Mar/18

V =∣ a_{1} ∙ (a_{2} \times a_{3}) ∣=∣ d e t [\begin{matrix} a_{1 x} & a_{1 y} & a_{1 z} \\ a_{2 x} & a_{2 y} & a_{2 z} \\ a_{3 x} & a_{3 y} & a_{3 z} \end{matrix}] ∣=∣ d e t [\begin{matrix} 2 & 3 & - 4 \\ 1 & 2 & - 3 \\ 3 & 6 & - 4 \end{matrix}] ∣= 5

Commented by NECx last updated on 17/Mar/18

T h a n k s

Commented by NECx last updated on 17/Mar/18

w h a t i f I d i d ∣ a_{2} ∙ (a_{1} \times a_{3}) ∣ w o u l d

I g e t t h e s a m e v a l u e ?

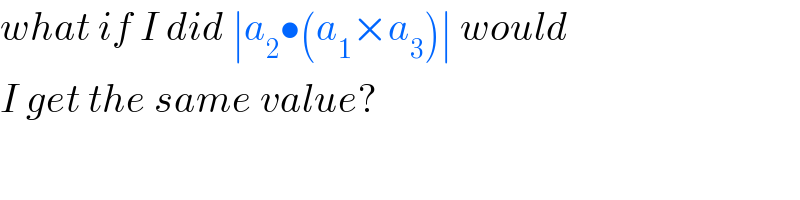
Commented by JDamian last updated on 18/Mar/18

D e f i n i t e l y - s w a p p i n g e i t h e r t w o r o w s o r t w o c o l u m n s o f a m a t r i x c h a n g e s t h e s i g n o f t h e v a l u e, w h i c h d o e s n o t m i n d b e c a u s e o f t h e a b s o l u t e v a l u e (∣ \cdot ∣) .

Facebook Tweet Pin