Question Number 48851 by vajpaithegrate@gmail.com last updated on 29/Nov/18
![the total no of solution of sin{x}=cos{x} where{x} denotes fractional part of x in [o 2π]is equal to ans:6](https://www.tinkutara.com/question/Q48851.png)
Commented by tanmay.chaudhury50@gmail.com last updated on 29/Nov/18
![sin{x}=cos{x} sin(x−[x])=cos(x−[x]) x={x}+[x] {x}=fractional part function [x]=greatest integer function from graph it is seen that in [0,2π] six(06) solution](https://www.tinkutara.com/question/Q48856.png)
Commented by tanmay.chaudhury50@gmail.com last updated on 29/Nov/18
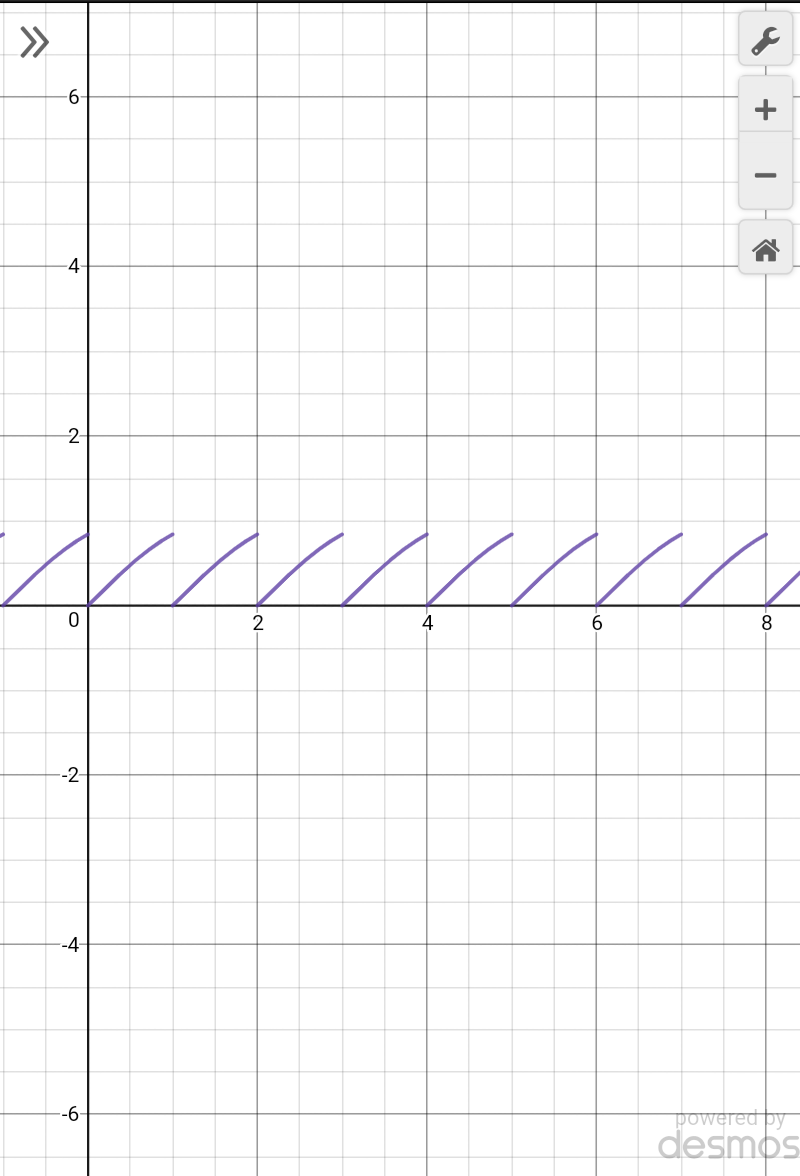
Commented by tanmay.chaudhury50@gmail.com last updated on 29/Nov/18
![graph of sin{x} sin(x−[x]](https://www.tinkutara.com/question/Q48858.png)
Commented by tanmay.chaudhury50@gmail.com last updated on 29/Nov/18
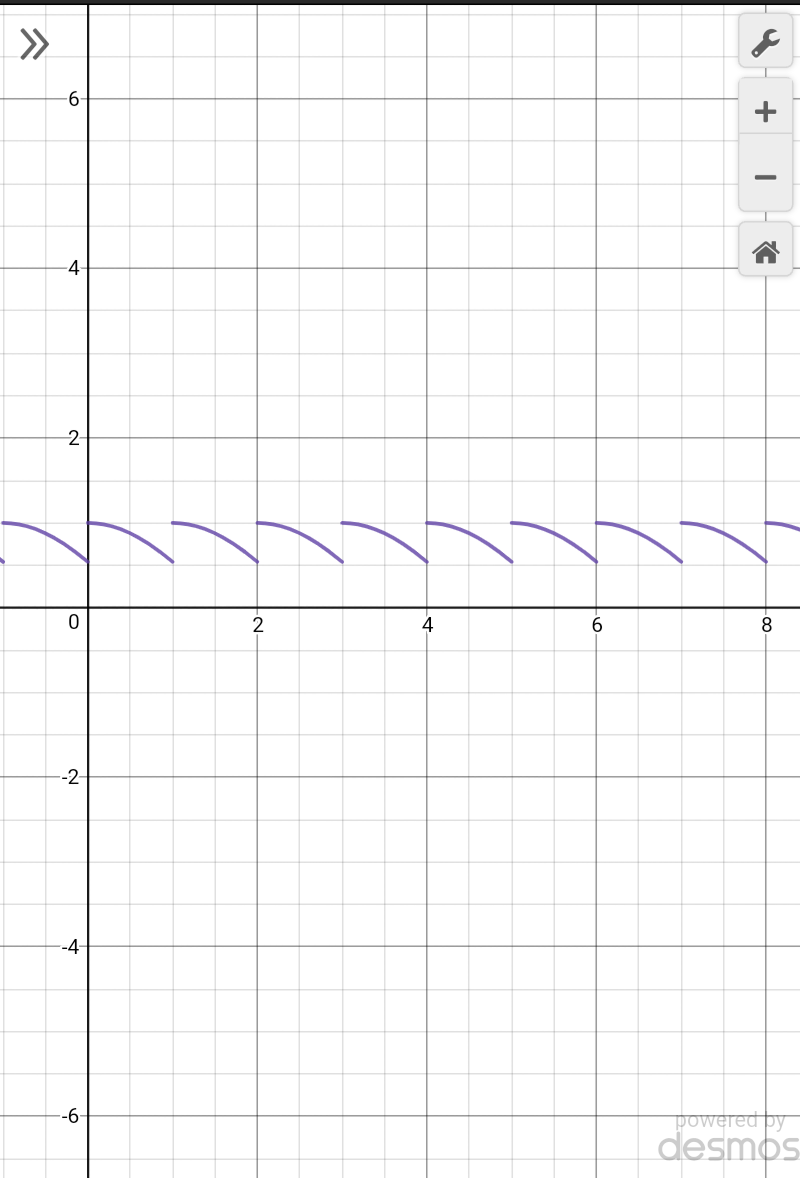
Commented by tanmay.chaudhury50@gmail.com last updated on 29/Nov/18
![graph of cos{x} graph of cos(x−[x])](https://www.tinkutara.com/question/Q48860.png)
Commented by tanmay.chaudhury50@gmail.com last updated on 29/Nov/18
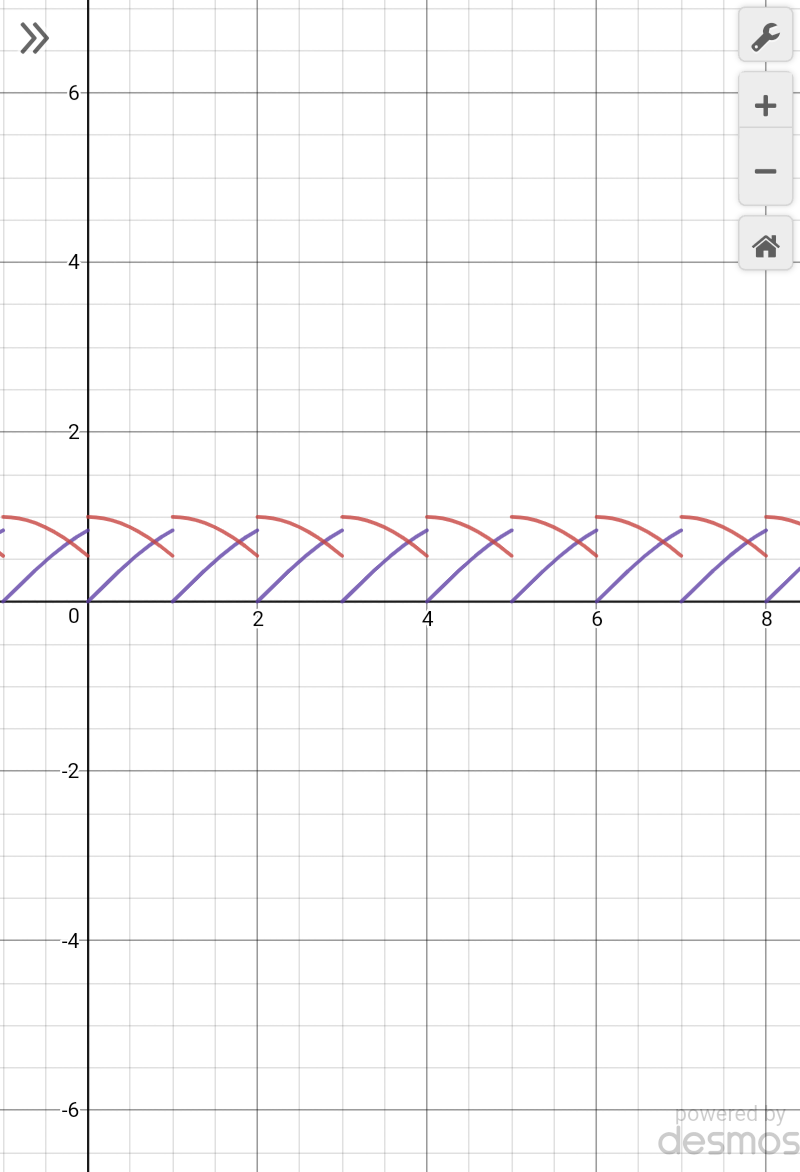
Commented by tanmay.chaudhury50@gmail.com last updated on 29/Nov/18

Commented by vajpaithegrate@gmail.com last updated on 29/Nov/18

Commented by vajpaithegrate@gmail.com last updated on 29/Nov/18
Answered by mr W last updated on 29/Nov/18
![let t={x} 0≤t<1 sin t=cos t ⇒tan t=1 ⇒t=π/4=0.785 ⇒solution is x=n+t with n=0,1,2,.... i.e. x=0.785, 1.785, 2.785,...,5.785, 6.785,...... 2π=6.283<6.785 ⇒6 solutions within [0, 2π]: x=0.785, 1.785, 2.785,...,5.785](https://www.tinkutara.com/question/Q48864.png)
Commented by vajpaithegrate@gmail.com last updated on 29/Nov/18

Commented by tanmay.chaudhury50@gmail.com last updated on 29/Nov/18

